Article contents
A bang-bang strategy for a finite fuel stochastic control problem
Published online by Cambridge University Press: 01 July 2016
Abstract
The problem treated is that of controlling a process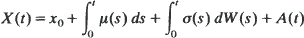 with values in [0, a]. The non-anticipative controls (µ(t), σ(t)) are selected from a set C(x) whenever X(t–) = x and the non-decreasing process A(t) is chosen by the controller subject to the condition
with values in [0, a]. The non-anticipative controls (µ(t), σ(t)) are selected from a set C(x) whenever X(t–) = x and the non-decreasing process A(t) is chosen by the controller subject to the condition  where y is a constant representing the initial amount of fuel. The object is to maximize the probability that X(t) reaches a. The optimal process is determined when the function
where y is a constant representing the initial amount of fuel. The object is to maximize the probability that X(t) reaches a. The optimal process is determined when the function  has a unique minimum on [0, a] and satisfies certain regularity conditions. The optimal process is a combination of ‘timid play' in which fuel is used gradually in the form of local time at 0, and ‘bold play' in which all the fuel is used at once.
has a unique minimum on [0, a] and satisfies certain regularity conditions. The optimal process is a combination of ‘timid play' in which fuel is used gradually in the form of local time at 0, and ‘bold play' in which all the fuel is used at once.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Applied Probability Trust 1992
Footnotes
Research supported by National Science Foundation Grants DMS-8801085 and DMS-8911548.
References
- 3
- Cited by

