Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Brozius, H. A.
1988.
Moment convergence of functions of the convex hull of a random sample.
Advances in Applied Probability,
Vol. 20,
Issue. 1,
p.
9.
Glendinning, R.H.
1991.
The convex hull of a dependent vector-valued process.
Journal of Statistical Computation and Simulation,
Vol. 38,
Issue. 1-4,
p.
219.
Molchanov, I. S.
1991.
Random sets. A survey of results and applications.
Ukrainian Mathematical Journal,
Vol. 43,
Issue. 12,
p.
1477.
Molchanov, Ilya S.
1993.
Limit theorems for convex hulls of random sets.
Advances in Applied Probability,
Vol. 25,
Issue. 2,
p.
395.
Molchanov, Ilya S.
1993.
Strong law of large numbers for unions of random closed sets.
Stochastic Processes and their Applications,
Vol. 46,
Issue. 2,
p.
199.
1993.
Statistics for Spatial Data.
p.
803.
Molchanov, Ilya S.
1993.
Limit theorems for convex hulls of random sets.
Advances in Applied Probability,
Vol. 25,
Issue. 2,
p.
395.
Mcbeth, Douglas
and
Resnick, Sidney
1994.
Stability of random sets generated by multivariate samples.
Communications in Statistics. Stochastic Models,
Vol. 10,
Issue. 3,
p.
549.
Zarepour, M.
1999.
Bootstrapping convex hulls.
Statistics & Probability Letters,
Vol. 45,
Issue. 1,
p.
55.
Pei, Tao
Zhu, A-Xing
Zhou, Chenghu
Li, Baolin
and
Qin, Chengzhi
2007.
Delineation of support domain of feature in the presence of noise.
Computers & Geosciences,
Vol. 33,
Issue. 7,
p.
952.
Balkema, A.A.
Embrechts, P.
and
Nolde, N.
2010.
Meta densities and the shape of their sample clouds.
Journal of Multivariate Analysis,
Vol. 101,
Issue. 7,
p.
1738.
Davis, Richard A.
and
Hueter, Irene
2013.
The convex hull of consecutive pairs of observations from some time series models.
Extremes,
Vol. 16,
Issue. 4,
p.
487.
Fresen, Daniel
and
Vitale, Richard
2014.
Concentration of random polytopes around the expected convex hull.
Electronic Communications in Probability,
Vol. 19,
Issue. none,
Nolde, Natalia
2014.
Geometric interpretation of the residual dependence coefficient.
Journal of Multivariate Analysis,
Vol. 123,
Issue. ,
p.
85.
Nolde, Natalia
2014.
The effect of aggregation on extremes from asymptotically independent light-tailed risks.
Extremes,
Vol. 17,
Issue. 4,
p.
615.
Molchanov, Ilya
2017.
Theory of Random Sets.
Vol. 87,
Issue. ,
p.
1.
Molchanov, Ilya
2017.
Theory of Random Sets.
Vol. 87,
Issue. ,
p.
379.
Molchanov, Ilya
2017.
Theory of Random Sets.
Vol. 87,
Issue. ,
p.
225.
Molchanov, Ilya
2017.
Theory of Random Sets.
Vol. 87,
Issue. ,
p.
317.
Molchanov, Ilya
2017.
Theory of Random Sets.
Vol. 87,
Issue. ,
p.
451.

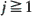 } is a sequence of i.i.d. random vectors in
} is a sequence of i.i.d. random vectors in 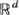 , when do there exist scaling constants
, when do there exist scaling constants 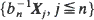 converges almost surely in the space of compact subsets of
converges almost surely in the space of compact subsets of 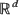 to a limit set? A multivariate regular variation condition on a properly defined distribution tail guarantees the almost sure convergence but without certain regularity conditions surprises can occur. When a density exists, an exponential form of regular variation plus some regularity guarantees the convergence.
to a limit set? A multivariate regular variation condition on a properly defined distribution tail guarantees the almost sure convergence but without certain regularity conditions surprises can occur. When a density exists, an exponential form of regular variation plus some regularity guarantees the convergence.