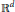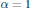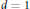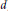Refine search
Actions for selected content:
2 results
Tail Bounds for the Stable Marriage of Poisson and Lebesgue
-
- Journal:
- Canadian Journal of Mathematics / Volume 61 / Issue 6 / 01 December 2009
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1279-1299
- Print publication:
- 01 December 2009
-
- Article
-
- You have access
- Export citation
The lattice of stable marriages and permutations
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics / Volume 33 / Issue 3 / December 1982
- Published online by Cambridge University Press:
- 09 April 2009, pp. 401-410
- Print publication:
- December 1982
-
- Article
-
- You have access
- Export citation