In this paper, we prove that the singular integral defined by

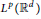${{T}_{\Omega ,a}}f(x)=\text{p}\text{.}\text{v}\text{.}{{\int }_{{{\mathbb{R}}^{d}}}}\frac{\Omega (x-y)}{|x-y{{|}^{d}}}\cdot {{m}_{x,y}}a\cdot f(y)dy$is bounded on ${{L}^{p}}({{\mathbb{R}}^{d}})$ for 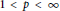$1\,<\,p\,<\,\infty $ and is of weak type (1,1), where 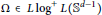$\Omega \,\in L\text{lo}{{\text{g}}^{+}}L({{S}^{d-1}})$ and 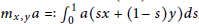${{m}_{x,y}}a\,=:\,\int{_{0}^{1}}\,a(sx\,+\,(1\,-\,s)y)ds$, with $a\,\in \,{{L}^{\infty }}({{\mathbb{R}}^{d}})\,$ satisfying some restricted conditions.

We establish the bounds of Marcinkiewicz integrals associated to surfaces of revolution generated by two polynomial mappings on Triebel–Lizorkin spaces and Besov spaces when their integral kernels are given by functions $\unicode[STIX]{x1D6FA}\in H^{1}(\text{S}^{n-1})\cup L(\log ^{+}L)^{1/2}(\text{S}^{n-1})$. Our main results represent improvements as well as natural extensions of many previously known results.

In this paper, we establish $BMO$ estimates for generalized commutators of rough fractional maximal and integral operators on generalized weighted Morrey spaces, respectively.

A class of generalized Marcinkiewicz integral operators is introduced, and, under rather weak conditions on the integral kernels, the boundedness of such operators on ${{L}^{p}}$ and Triebel–Lizorkin spaces is established.

In this paper, we give the ${{L}^{p}}$ boundedness for a class of parabolic Littlewood–Paley $g$-function with its kernel function $\Omega$ is in the Hardy space ${{H}^{1}}\left( {{S}^{n-1}} \right)$.

Let Ω(y′) be an H1(Sn−1) function on the unit sphere satisfying a certain cancellation condition. We study the Lp boundedness of the singular integral operator where α≥n and ρ is a norm function which is homogeneous with respect to certain nonistropic dilation. The result in the paper substantially improves and extends some known results.

In this paper the authors establish the $L^p$ boundedness for several classes of Marcinkiewicz integral operators with kernels satisfying a condition introduced by Grafakos and Stefanov in Indiana Univ. Math. J.47 (1998), 455–469.

AMS 2000 Mathematics subject classification: Primary 42B25; 42B99

In this note the authors give the L2(n) boundedness of a class of parametric Marcinkiewicz integral with kernel function Ω in L log+L(Sn−1) and radial function h(|x|) ∈ l ∞ l(Lq)(+) for 1 < q ≦.

As its corollary, the Lp (n)(2 < p < ∞) boundedness of and and with Ω in L log+L (Sn-1) and h(|x|) ∈ l∞ (Lq)(+) are also obtained. Here and are parametric Marcinkiewicz functions corresponding to the Littlewood-Paley g*λ-function and the Lusin area function S, respectively.

Let $b(t)$ be an ${{L}^{\infty }}$ function on $\mathbf{R}$, $\Omega ({y}')$ be an ${{H}^{1}}$ function on the unit sphere satisfying the mean zero property (1) and ${{Q}_{m}}(t)$ be a real polynomial on $\mathbf{R}$ of degree $m$ satisfying ${{Q}_{m}}(0)\,=\,0$. We prove that the singular integral operator

$${{T}_{Qm,}}b\left( f \right)\left( x \right)=p.v.\int\limits_{\mathbf{R}}^{n}{b\left( \left| y \right| \right)}\Omega \left( y \right){{\left| y \right|}^{-n}}f\left( x-{{Q}_{m}}\left( \left| y \right| \right){y}' \right)\,\,dy$$

is bounded in ${{L}^{p}}({{\mathbf{R}}^{n}})$ for $1<p<\infty $, and the bound is independent of the coefficients of ${{Q}_{m}}(t)$.