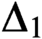 $\Delta_1$
GAMES
$\Delta_1$
GAMES
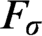 $F_\sigma $
GAMES AND REFLECTION IN
$F_\sigma $
GAMES AND REFLECTION IN 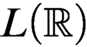 $L(\mathbb {R})$
$L(\mathbb {R})$