Refine search
Actions for selected content:
7 results
Prolactin-stimulated polymerization of acetyl-CoA carboxylase in explants of mid-pregnant rat mammary gland
-
- Journal:
- Journal of Dairy Research / Volume 68 / Issue 3 / August 2001
- Published online by Cambridge University Press:
- 15 October 2001, pp. 351-355
- Print publication:
- August 2001
-
- Article
- Export citation
A branching-process solution of the polydisperse coagulation equation
-
- Journal:
- Advances in Applied Probability / Volume 16 / Issue 1 / March 1984
- Published online by Cambridge University Press:
- 01 July 2016, pp. 56-69
- Print publication:
- March 1984
-
- Article
- Export citation
Relaxed Markov processes
-
- Journal:
- Advances in Applied Probability / Volume 15 / Issue 4 / December 1983
- Published online by Cambridge University Press:
- 01 July 2016, pp. 769-782
- Print publication:
- December 1983
-
- Article
- Export citation
Susceptibility to weathering of some Scottish rocks and their derived soils
-
- Journal:
- Transactions of the Royal Society of Edinburgh: Earth Sciences / Volume 73 / Issue 4 / 1983
- Published online by Cambridge University Press:
- 03 November 2011, pp. 191-203
- Print publication:
- 1983
-
- Article
- Export citation
Polymerisation processes with intrapolymer bonding. II. Stratified processes
-
- Journal:
- Advances in Applied Probability / Volume 12 / Issue 1 / March 1980
- Published online by Cambridge University Press:
- 01 July 2016, pp. 116-134
- Print publication:
- March 1980
-
- Article
- Export citation
Polymerisation processes with intrapolymer bonding. III. Several types of unit
-
- Journal:
- Advances in Applied Probability / Volume 12 / Issue 1 / March 1980
- Published online by Cambridge University Press:
- 01 July 2016, pp. 135-153
- Print publication:
- March 1980
-
- Article
- Export citation
Polymerisation processes with intrapolymer bonding. I. one type of unit
-
- Journal:
- Advances in Applied Probability / Volume 12 / Issue 1 / March 1980
- Published online by Cambridge University Press:
- 01 July 2016, pp. 94-115
- Print publication:
- March 1980
-
- Article
- Export citation
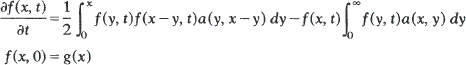
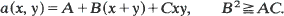
 diverges corresponds to a critical branching process, while post-critical times
diverges corresponds to a critical branching process, while post-critical times 