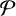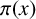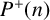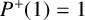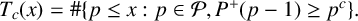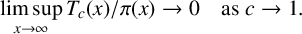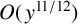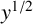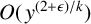Refine search
Actions for selected content:
3 results
ON A CONJECTURE ON SHIFTED PRIMES WITH LARGE PRIME FACTORS, II
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 111 / Issue 1 / February 2025
- Published online by Cambridge University Press:
- 13 September 2024, pp. 48-55
- Print publication:
- February 2025
-
- Article
- Export citation
A NOTE ON POWERFUL NUMBERS IN SHORT INTERVALS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 108 / Issue 1 / August 2023
- Published online by Cambridge University Press:
- 22 September 2022, pp. 99-106
- Print publication:
- August 2023
-
- Article
- Export citation
2 - The Sieves of Brun and Selberg
-
- Book:
- Bounded Gaps Between Primes
- Published online:
- 10 September 2021
- Print publication:
- 25 February 2021, pp 35-88
-
- Chapter
- Export citation