In this article, we establish a new atomic decomposition for 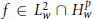 $f\,\in \,L_{w}^{2}\,\bigcap \,H_{w}^{p}$, where the decomposition converges in
$f\,\in \,L_{w}^{2}\,\bigcap \,H_{w}^{p}$, where the decomposition converges in  $L_{w}^{2}$-norm rather than in the distribution sense. As applications of this decomposition, assuming that
$L_{w}^{2}$-norm rather than in the distribution sense. As applications of this decomposition, assuming that  $T$ is a linear operator bounded on
$T$ is a linear operator bounded on  $L_{w}^{2}$ and
$L_{w}^{2}$ and 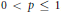 $0\,<\,p\,\le \,1$, we obtain (i) if
$0\,<\,p\,\le \,1$, we obtain (i) if  $T$ is uniformly bounded in
$T$ is uniformly bounded in  $L_{w}^{p}$-norm for all
$L_{w}^{p}$-norm for all 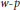 $w-p$-atoms, then
$w-p$-atoms, then  $T$ can be extended to be bounded from
$T$ can be extended to be bounded from  $H_{w}^{p}$ to
$H_{w}^{p}$ to  $L_{w}^{2}$; (ii) if
$L_{w}^{2}$; (ii) if  $T$ is uniformly bounded in
$T$ is uniformly bounded in  $H_{w}^{p}$-norm for all
$H_{w}^{p}$-norm for all 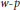 $w-p$-atoms, then
$w-p$-atoms, then  $T$ can be extended to be bounded on
$T$ can be extended to be bounded on  $H_{w}^{p}$; (iii) if
$H_{w}^{p}$; (iii) if  $T$ is bounded on
$T$ is bounded on  $H_{w}^{p}$, then
$H_{w}^{p}$, then  $T$ can be extended to be bounded from
$T$ can be extended to be bounded from  $H_{w}^{p}$ to
$H_{w}^{p}$ to  $L_{w}^{2}$.
$L_{w}^{2}$.