The singular spectrum of  $u$ in a convolution equation
$u$ in a convolution equation  $\mu *u\,=\,f$, where
$\mu *u\,=\,f$, where  $\mu$ and
$\mu$ and  $f$ are tempered ultra distributions of Beurling or Roumieau type is estimated by
$f$ are tempered ultra distributions of Beurling or Roumieau type is estimated by
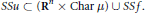 $$SSu\,\subset \,\left( {{\mathbf{R}}^{n}}\,\times \,\text{Char}\,\mu \right)\,\cup \,SSf$$
$$SSu\,\subset \,\left( {{\mathbf{R}}^{n}}\,\times \,\text{Char}\,\mu \right)\,\cup \,SSf$$
The same is done for 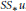 $S{{S}_{*}}u$.
$S{{S}_{*}}u$.
 —Propagation of Singularities
—Propagation of Singularities