INTRODUCTION
Brucellosis is a highly contagious zoonotic disease affecting terrestrial mammals. It is caused by species of the genus Brucella: B. melitensis, B. abortus, B. suis, B. neotomae, B. ovis and B. canis [Reference Moreno, Cloeckaert and Moriyón1]. Human brucellosis is endemic in Middle Eastern countries and recent reports suggest that its incidence may be increasing [Reference Benkirane2, Reference Refai3]. Prevention of human brucellosis relies on the control of the disease in animals, which has traditionally consisted of a combination of test-and-slaughter and/or vaccination [Reference Godfroid4]. Given that infected animals are the source of human infection, the increasing incidence of human brucellosis may be indicative of a similar trend in domestic animals.
The incidence of cattle brucellosis became alarming in Egypt following the large-scale importation of Friesian cows for the establishment of private and governmental farms in the late 1970s and early 1980s [Reference Refai, El-Gibaly, Adwi and Adams5]. Since then, several attempts have been made to control the disease either through national programmes or through bilateral projects in partnership with agencies or international organizations. Initially, these control measures focused on cattle only and did not prevent the spread of B. melitensis from sheep and goats to the cattle and buffaloes population [Reference Refai6]. Nowadays, B. melitensis biovar 3 is the predominant isolate from humans and animals in Egypt [Reference Refai3, Reference Jennings7, Reference Moghney8].
The current official control policy of brucellosis in Egypt consists of:
(i) Serological testing every 6 months of all female cows, buffaloes, sheep and goats aged ⩾6 months and valuable bulls, rams and bucks using the complement fixation test (CFT) and Rose Bengal plate test (RBPT), with removal of positive and suspicious cases and compensation to the owners [Reference Refai3, Reference Refai6–Reference Jennings9].
(ii) Quarantine and disinfection of farms with infected animals of any ruminant species which are serologically tested every 21 days using RBPT and CFT and declared free after three successive negative tests.
(iii) Voluntary vaccination of calves, lambs and kids which are serologically negative at age 3–6 months, using S19 vaccine for cattle and buffaloes and Rev1 vaccine for sheep and goats [Reference Refai6].
(iv) Reporting of aborted animals by veterinary authorities with samples from aborted animals submitted to laboratories for isolation and identification of the causative agent.
(v) Quarantine and serological testing (twice; at arrival and at parturition) of imported pregnant heifers.
(vi) Market control for dairy products by controllers of the Public Health and Veterinary sectors (a combined team from the Ministry of Health, Ministry of Agriculture and Ministry of Supply and Home Trade are responsible for collecting samples which are tested in two different laboratories belonging to the Ministry of Health and the Ministry of Agriculture) and trace-back of the positive samples to their origin [Reference Refai6]. Since their establishment in 1985 these control measures have been revised several times, the last one in 1999.
These revisions have included minor changes such as delaying the slaughtering of seropositive pregnant females until parturition, exclusion of farms vaccinated with B. abortus S19 from the test-and-slaughter programme and increases in compensation paid to farmers for animals slaughtered.
Reliable estimates of the frequency of animal brucellosis in the country as a whole or in large areas of the country are lacking [Reference Abdel-Hafeez10]. A recent study on animal (cattle, sheep, buffaloes, goats) seroprevalences in two villages in the Gharbia governorate reported no animal reactors in one village and 16% seroprevalence in the other village [Reference El Sherbini11]. Infection in humans reportedly increased from an annual incidence of 0·5/100 000 in 1994 to 1·9/100 000 population in 1998 [Reference Refai3]. A more recent study estimated an annual incidence of human brucellosis of 64 cases/100 000 in 2002 and 70 cases/100 000 in 2003 in the Fayoum governorate [Reference Jennings7]. This apparent increase in the incidence of human brucellosis in recent years, despite the existence of an official control programme that follows FAO/WHO/OIE guidelines for Middle Eastern countries [Reference Refai3], may reflect that either the official control programme is not suitable for the situation in Egypt or it is not being followed.
This present study has two objectives, first to assess the extent to which the test-and-slaughter and quarantine measures of the official control programme as outlined above have been adhered to in a selected area of the Nile Delta in Egypt (Kafr El Sheikh governorate), second to simulate the effectiveness of the current official test-and-slaughter programme at different levels of implementation on the prevalence of brucellosis in the small-ruminant population of a typical village in the Nile Delta.
METHODS
Assessment of the implementation of official control measures
Study area and livestock population
We studied the activities of the national brucellosis control programme in the Kafr El Sheikh governorate. This is one of the 27 governorates of Egypt. It is located in the northern part of the country between 31° 7′ North and 30° 57′ East. The governorate is divided into 10 districts (Fig. 1).
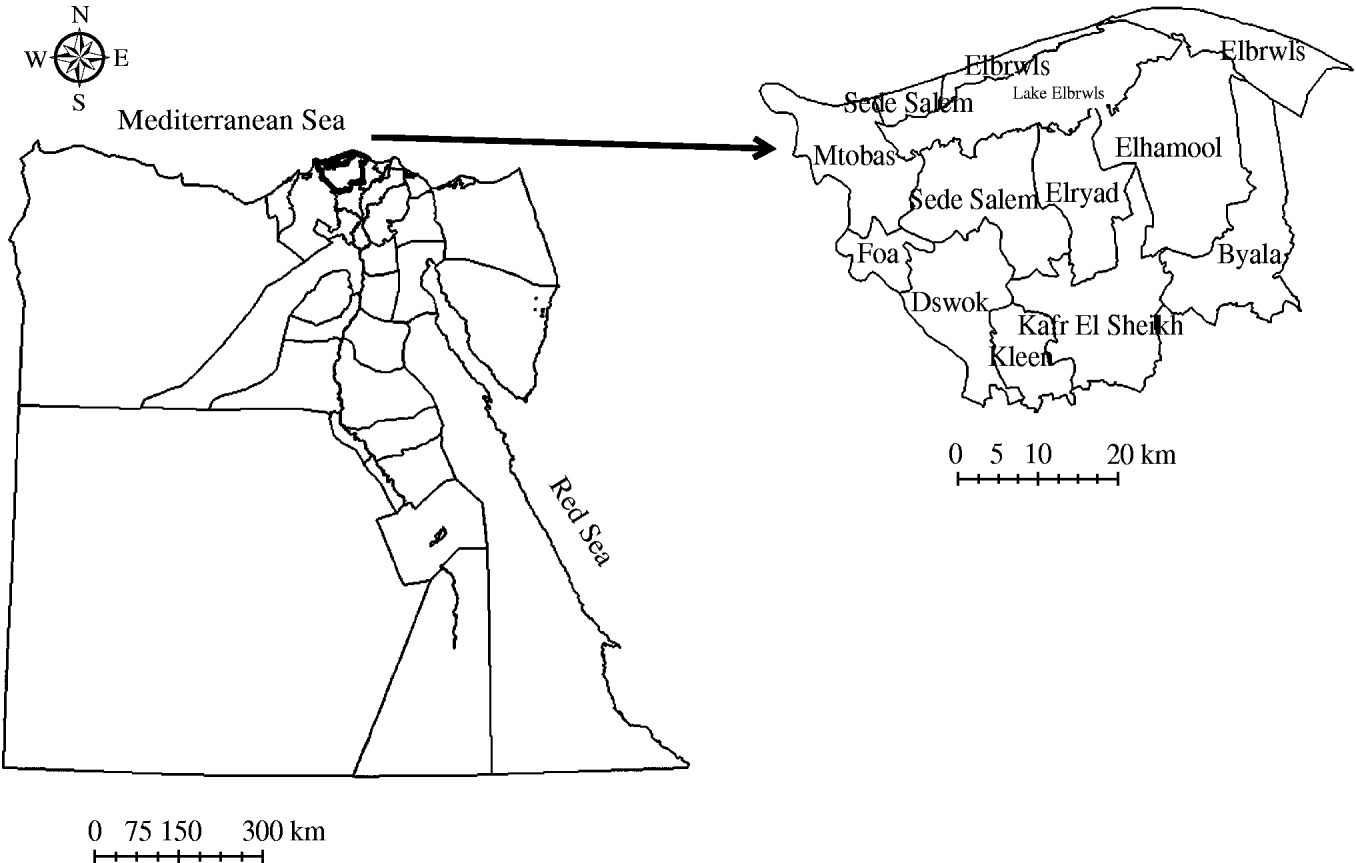
Fig. 1. Map of Egypt showing the location of Kafr El Sheikh governorate and its districts.
Data on the total numbers of cattle, buffaloes, sheep and goats by district were obtained from the official Local Veterinary Directorate of the Governorate for 2005. In this dataset, the total numbers of animals from the different species in each district were disaggregated by sex and age group. The total numbers for the different species for the whole governorate and the minimum and maximum in a single district were: total cattle 155 737 (3200–38 717), total buffaloes 103 019 (610–29 199), sheep 120 799 (2000–24 000) and goats 12 770 (300–3850). In Kafr El Sheikh, cattle and buffaloes are mainly raised in small groups ranging from 1 to 5 animals per household (extensive system). These animals stay on the farmer's land all day where they may have contact with animals from other households [Reference Aidaros12]. Within each village, there are usually 3 or 4 herders responsible for collective flocks that typically include between 20 and 200 sheep and goats from a number of households plus some animals owned by the herders themselves. Flocks often move outside the boundaries of the village [Reference Al-Keraby and Thomson13].
Data on the brucellosis control programme implemented in the study area
The number of animals of the different species tested for brucellosis and the number of positive cases were obtained, by district, from the official Local Veterinary Directorate of the Governorate. The available data consisted of annual figures for the years 1995, 1996, 1997, 2000, 2001, 2002, 2003, 2004, 2005 and 2006 and also monthly data for the years 2000, 2001, 2002 and 2005. The samples were not grouped by farm or household of origin. The only available data apart from the test result were the district of origin, date of sampling and species. There was no indication in the data of whether samples were from male or female livestock. As all female livestock aged ⩾6 months are sampled, whereas only valuable bulls, rams and bucks of the same age are sampled, and because the number of valuable bulls, rams and bucks in Egypt is very small relative to the number of females, the assumption was made that all samples were from female livestock.
Comparison of the official control measures and measures implemented
It is known from the field experience of the senior author of this work (Y.M.H.), which included informal interviews with local veterinarians during 2007 and 2008, that in recent years the official veterinary services have not implemented a vaccination programme in any species in the governorate. The test-and-slaughter policy is often conducted intermittently, primarily due to a lack of funding.
Test-and-slaughter policy
To assess the extent to which the actual serological testing met the target of testing all female animals aged ⩾6 months every 6 months, sampling fractions were obtained by dividing the number of tested animals every year by the baseline population (censuses 2005) [14]. To evaluate potential demographic and geographical biases of the sample in respect of the baseline populations, sampling fractions were obtained by species and by district and represented using choropleth maps (i.e. maps in which areas within a region of interest are shaded according to the value of attributes) [Reference Andrienko and Andrienko15]. In these maps, the minimum, average and maximum sampling fractions for each species in each district over the 10 years of available data were illustrated using different colour intensities.
Quarantine measures
To determine whether herds or flocks with seropositive cases were retested every 21 days until three successive negative tests were obtained, as indicated in the official control programme, we used monthly testing data from January 2000 to December 2002. Due to lack of data at individual animal or herd level, disease events were defined at district level. A disease event was, thus, a district in which at least one seropositive animal of a certain species was found in a given month. The probability of retesting was estimated as the proportion of disease events (districts with positive cases in a certain species) that were followed by the retesting, in the same district, of animals of the same species in the next month and in next two successive months. The relative risk of no submission of samples following disease events in the different species was calculated with cattle as the baseline group, using:
where D1 is the number of disease events in one particular species that was not followed by the resampling, in the same district, of animals of the same species within either 1 or 2 months; D2 is the number of disease events in cattle that was not followed by the resampling, in the same district, of cattle within either 1 or 2 months; N1 is the number of disease events in that particular species, and N2 is the number of disease events in cattle.
Simulation of the effect of different test-and-slaughter policies
A stochastic mathematical model was developed to assess the impact of the application of the current test-and-slaughter policy on the prevalence of brucellosis in the small-ruminant population. The unit of study was a village and the policies tested were test and slaughter of positive animals every 1, 2 and 5 years.
Model structure
The model simulated the dynamics of brucellosis within the sheep/goat population of a single village with 600 sheep/goats. It was assumed that each individual in the small-ruminant population existed in a mutually exclusive state; female lambs and kids used as replacement (B), susceptible small-ruminant females (S), infectious small-ruminant females (I) and positive non-infectious small-ruminant females (Pn). The infectious and the positive non-infectious animals constitute the positive animals in the population, and their proportion over the whole small-ruminant stock in the village is the within-village prevalence.
The number of newborn female lambs and kids kept every year as replacement (K) was assumed to be equal to the number of animals that die or are culled by the farmer regardless of their status against Brucella sp. (T1) plus the number of animals detected as seropositive to Brucella sp. and culled by the control campaign (T2); where:
T1S, T1I and T1Pn are the numbers of animals removed every year without having been tested for brucellosis due to mortality plus voluntary culling of susceptible, infectious and positive non-infectious animals, respectively. T2S, T2I and T2Pn are the numbers of animals detected as seropositive to Brucella sp. and culled by the control campaign every year from the groups of susceptible (false positives), infectious and positive non-infectious animals, respectively.
After age 6 months, newborn female lambs and kids kept for replacement become susceptible. Animals change status (from B to S, from S to I and from I to Pn) with different constant or probability flow rates.
Those susceptible females, that had effective contacts with infectious animals, become infectious at an infection rate (IR) of:
where β (transmission coefficient) is the number of animals that come into effective contact with one infectious animal per unit of time, NI is the number of infectious small-ruminant females per village and NS is the number of susceptible small-ruminant females per village. Other susceptible animals are removed from the population either due to mortality or voluntary culling (T1S) or due to the culling of false-positive animals by the control campaigns (T2S). Sixty days after the start of the infectious period (D), infectious animals become positive non-infectious. Some of the infectious and positive non-infectious animals are removed from the population by either mortality or voluntary culling (T1I and T1Pn, respectively) or as a result of testing positive during control campaigns (T2I and T2Pn, respectively). The equations of the flow rates and the general model structure are shown in Figure 2.
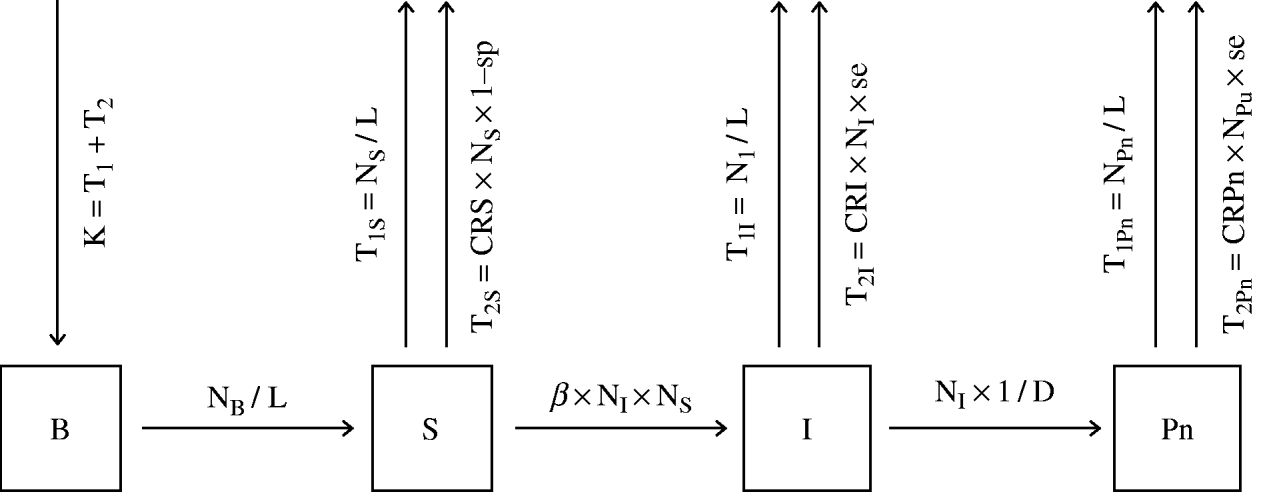
Fig. 2. Structure of a model for the simulation of the dynamics of brucellosis within the sheep/goat population of a village of Kafr El Sheikh governorate. B, Newborn female lambs and kids kept for replacement; S, susceptible females; I, infectious animals; Pn, positive non-infectious animals; NB, the number of female lambs and kids per village; K, the number of female lambs and kids kept for replacement every year; NI, the number of infectious small-ruminant females per village; NS. the number of susceptible small-ruminant females per village; NPn, the number of positive non-infectious small-ruminant females per village; T1S, T1I, T1Pn, the numbers of susceptible, infectious and positive non-infectious animals removed by normal mortality and culling every year, respectively; T2S, T2I, T2Pn, the numbers of seropositive susceptible, infectious and positive non-infectious animals removed every year by the control campaign; D, infectious period; L, life expectancy; se and sp, sensitivity and specificity of serological tests, respectively; CRS, CRI, CRPn, the proportion of susceptible, infectious and positive non-infectious animals tested every year, respectively.
Input parameters and assumptions
Whenever possible, input parameters were derived from the literature or our ongoing field work in the area. However, assumptions were necessary for other unknown parameters or for those with wide variability (Table 1).
Table 1. Assumptions of input parameters used in the model with symbols, values and distributions

The sheep/goat population was assumed to be closed with homogenous mixing and to be of fixed size. Hence, the number of newborn female animals kept in the village was assumed to be equal to the number of animals leaving the population as a result of mortality and voluntary culling (T1) plus the culling of positive animals detected by test-and-slaughter campaigns (T2). Our objective was to test the effectiveness of the applied practice (not the official guidelines), and for this reason, it was assumed that no vaccination against brucellosis was practised in the village. Life expectancy (L), which constitutes the average life expectancy in the sheep/goat population, was assumed to be 4 years for sheep and goats.
Our findings on the percentage of the total livestock population tested for brucellosis in any given year during the study period (presented in the Results section) show that no more than 5% of the total small-ruminant population in the governorate had been tested for brucellosis in any year. At district level, the maximum proportion of small ruminants tested for any district during the study period was 18%. It was assumed in this model that, under current circumstances, a test-and-slaughter campaign would test between 5% and 15% of the small-ruminant population in the village. This range of proportion of tested animals was considered realistic and was used as a stochastic input parameter with uniform probability distribution (Table 1).
Based on preliminary results of an ongoing serological survey in the area that shows within-village prevalence ranging between 5% and 15% for six randomly selected villages in the governorate, within-village seroprevalence was modelled as a stochastic input parameter with uniform distribution between 5% and 15%.
It was assumed that serological testing consisted of a combination of CFT and RBPT as stated in the official control programme. The sensitivity of CFT and RBPT were assumed to be 88·6% and 92·1%, respectively [Reference Garin-Bastuji16]. Their combined specificity has been reported to range from 68·8% to 100% [Reference Nielsen17]. In the model, both sensitivity and specificity values were used as stochastic input parameters ranging from 70% to 100%.
Sheep and goats were assumed to be non-susceptible to infection until reaching age 6 months [Reference Corbel18]. Following infection, the incubation period of the disease has been estimated to range between 8 and 20 days and the infectious period between 21 and 90 days or more [19]. An assumption was made that following infection, a susceptible animal remained infectious during a period (D) of 60 days.
The transmission coefficient (β) was derived by assuming that brucellosis is at endemic equilibrium in the village [Reference Heffernan, Smith and Wahl20]. At endemic equilibrium the number of newly infected animals produced by one already infectious animal during its infectious period (true reproduction number R) equals 1 (R=1), and
where R 0 is the basic reproduction number and represents the number of newly infected animals produced by an infectious animal introduced in a disease-free flock. S* is the proportion of susceptible animals in the village. The basic reproduction number (R 0) and the transmission coefficient (β) are related by the formula:
where D is the infectious period and N is the total number of animals in the village.
The transmission coefficient (β) was calculated using:
In a village with 5% prevalence, the transmission coefficient is 0·000034 animal/day and in a village with 15% prevalence, 0·000038 animal/day. A uniform probability distribution between these two values was used as stochastic parameter for the transmission coefficient (Table 1).
Model settings and validation of β estimates
The model was run for 1000 simulations for each control policy and for 10 replications using Powersim Studio 2005 (Powersim software AS). The time step was set to 1 month, with the testing and slaughtering of animals occurring once a year. Each model was run for a period of 20 years, the output was the within-village prevalence over 20 years. The model was run for 10 000 simulations after excluding the culling due to control campaigns to test if the β parameters used were in concordance with the assumed endemic equilibrium.
Effect of increasing the proportion of tested animals
The model was used to simulate the impact on the end prevalence of 20 years of implementation of disease control scenarios with different proportions of animals tested every year. The first simulation assumed that 20% of animals were tested every year, with subsequent simulations increasing this proportion by 10% up to 100% of animals tested every year.
Sensitivity analysis
Sensitivity analysis was conducted to assess the impact of transmission coefficient, life expectancy and combined sensitivity and specificity of the diagnostic tests on the end prevalence of brucellosis under the three scenarios of control measures. It was performed by decreasing and increasing one parameter at a time by 5% and 10% of its original value while keeping the original values of the remaining parameters (for the combined sensitivity and specificity of the serological tests, only decreasing values were assessed, since the original distribution used in the model had a maximum value of 100%). The models were run 10 000 times. The end prevalences of each model were recorded and compared with each other.
RESULTS
Assessment of the implementation of official control measures
Test-and-slaughter policy
The fractions of the total female stock aged ⩾6 months, sampled in any of the 10 years for which data was available, ranged from 2·2% to 6·5%. By species, the percentages sampled in a given year ranged between 2·2% and 7·5% for cattle, 1·4% and 7·4% for buffaloes, 0·9% and 4·8% for sheep and 0·4% and 4·6% for goats (Fig. 3 a, b).

Fig. 3. (a) Percentage of total female stock aged ⩾6 months and (b) percentage of female cattle, buffaloes, sheep and goats aged ⩾6 months, serologically tested for brucellosis in Kafr El Sheikh governorate every year from 1995 to 2006. No data were available for the years 1998 and 1999.
The sampling fractions by species and districts show that there was a marked spatial heterogeneity in the sampling. Cattle and buffaloes were consistently the species for which a higher proportion of the population was sampled (Fig. 4). However, even for these species, there were some districts in which not a single animal was sampled in any of the 10 years. Sampling was much less intense for sheep and goats, as in more than half of the districts there was no sampling of sheep or goats in any of the 10 years.

Fig. 4. Minimum, average and maximum brucellosis sampling fractions in different livestock species in the districts of Kafr El Sheikh from 1995 to 2005.
Quarantine measures
Between January 2000 and December 2002, 101 disease events (positive district with at least one seropositive animal detected in the month) were confirmed. The number of these events that would be detectable in our database if followed by retesting in the next month was 97 (Table 2). Eighty-two percent of the disease events serologically identified in Kafr El Sheikh governorate were followed by retesting in the following month. The lowest percentage of retesting was in goats (62%) and the highest was in cattle (91%). The relative risks of not having samples submitted following disease events in buffaloes, sheep or goats, compared with cattle, were 2·8, 2·5 and 4·2, respectively.
Table 2. Frequency with which the finding of brucellosis-positive animals in one district was followed by the retesting of animals of the same species in the same district in the following month and in the following two successive months, during 2000–2002
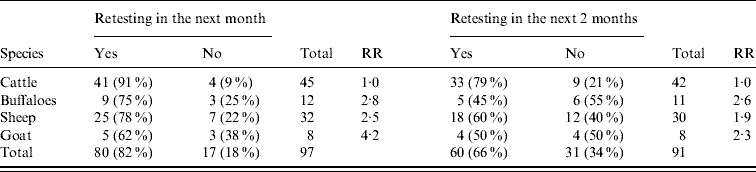
RR, Relative risk for not retesting positive herds in the different species.
When a more strict definition of retesting was used (sampling in the 2 months following a positive test), 91 events were detectable in our database. The percentage of these events that were followed by retesting in the following two successive months decreased to <66%. The highest percentage of retesting was in cattle (79%), while in other species only around half of the disease events were retested. The relative risks of no sample submission in two successive months following disease events in buffaloes, sheep or goats were 2·6, 1·9 and 2·3, respectively (Table 2).
Simulation of the effect of different test-and-slaughter policies
The values estimated for the transmission coefficient were consistent with the assumed endemic equilibrium state. The estimated average, 10th percentile and 90th percentile of within-village prevalence of brucellosis over the period of simulation (20 years) for the three different scenarios of implementation of control measures are shown (Fig. 5 a–c).
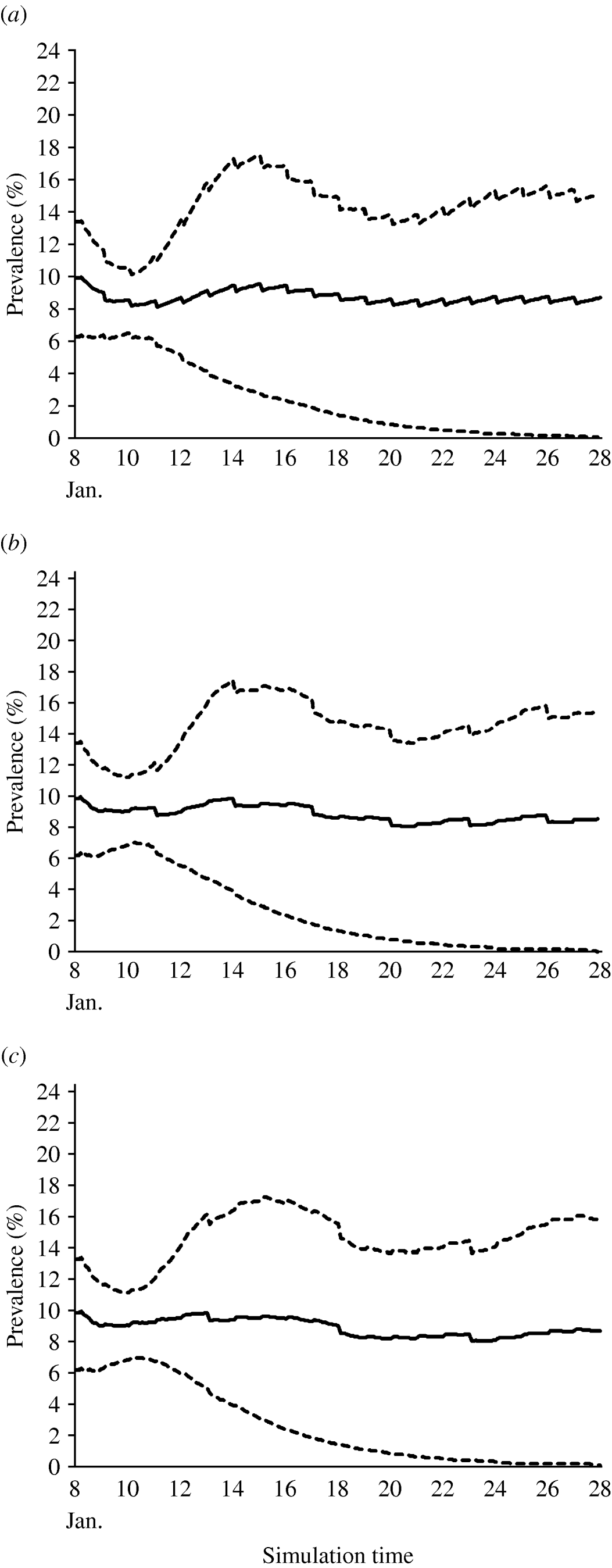
Fig. 5. The estimated average prevalence (——) of brucellosis, and the 10th and 90th percentiles (- - - -) over a 20-year time period with a test-and-slaughter programme applied (a) every year, (b) every 3 years and (c) every 5 years.
In the first scenario, with test and slaughter taking place in the village every year, the yearly disease prevalence was predicted to decrease gradually until the 5th year of simulation when it reached 8%. Then, the prevalence was predicted to become stable (endemic) at a level of almost 8·5%. The other two scenarios, with test and slaughter every 3 or 5 years, predicted the prevalence to become endemic at 9% during the 20-year simulation period.
Simulation of testing and slaughtering increasing proportions of animals demonstrated that, if the policy was applied every year, the prevalence of brucellosis steadily decreased as the proportion of animals tested increased. When 80% of animals were tested per year and the positive animals slaughtered, the prevalence of infection fell to <1% in 4 years since the beginning of simulations (Fig. 6). When the policy was applied every 3 and 5 years, increasing the proportion of animals tested resulted in a slow decrease in prevalence to around 2% and 3·5%, respectively, when 100% of animals were tested.
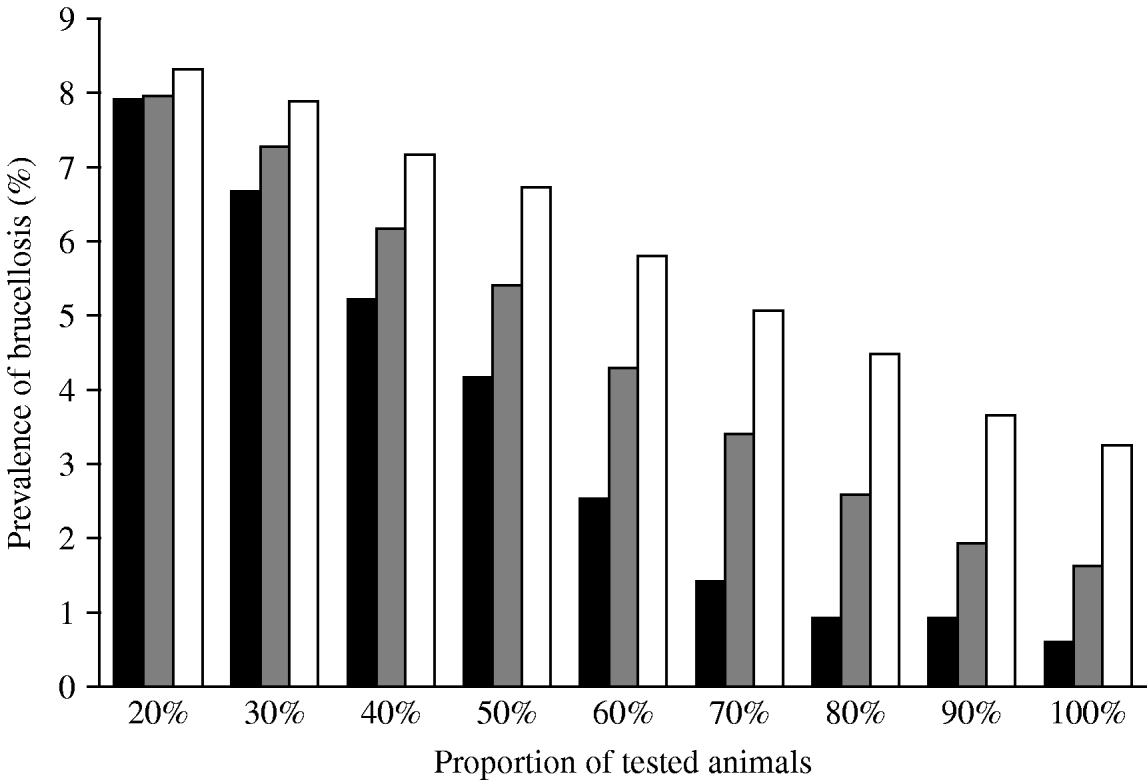
Fig. 6. Simulation of the effect of a test-and-slaughter programme with testing of different proportions of animals every 1 year (▪), 3 years (![]() ) or 5 years (□) on the within-village prevalence of brucellosis in small ruminants for a period of 20 years.
) or 5 years (□) on the within-village prevalence of brucellosis in small ruminants for a period of 20 years.
Results of the sensitivity analysis showed that changes in the value of the transmission coefficient had a considerable effect on the end prevalence for the three control scenarios, for example, increasing the transmission coefficient by 10% of its original value when the control policy was applied every year resulted in an end prevalence of 17%, while decreasing it by the same value resulted in end prevalence of 1%. Changes in life expectancy resulted in end prevalences of 9·4% and 6·75%. A 10% decrease in the value of combined specificity and sensitivity resulted in an end prevalence of 8%.
DISCUSSION
According to the official brucellosis control programme in Egypt, all female livestock aged ⩾6 months and valuable male livestock should be serologically tested against brucellosis every 6 months. It is apparent from the present study that the official control programme in Kafr El Sheikh governorate was not being adhered to between 1995 and 2006, as our analysis showed that <7% of female livestock were tested in any given year in the study area. Although we used a fixed census of animal population (2005), this is unlikely to have influenced our results significantly, since the total animal population does not change more than 10% across the 10 years of the study. During this period, the main trends in the governorate were towards an increase in the total number of cattle and buffalo farms with a decline in the total cattle and buffalo population (i.e. an increasing number of small cattle and buffalo farms), an increase in total goat population numbers generally and fluctuations without a clear trend for the sheep population [14].
The level of serological sampling varied markedly between different districts and species within the governorate. Kafr El Sheikh was the only district having consistent sampling every year, and a relatively high proportion of the total female livestock within this district was tested. In comparison, Dswok, which is the district with the largest livestock population in the governorate, was sampled in a very inconsistent manner, and only a small proportion of the total female livestock was tested. Cattle and buffaloes were consistently sampled in most districts during the 10-year study period. In contrast, sheep and goats were sampled in a very inconsistent manner except in three districts, and in relatively low proportions.
Although the specific reasons for lack of compliance with the official control programme are likely to be numerous, field observations by the senior author (Y.M.H.) suggest that this is the result of unrealistically high sampling targets given the resources available, poor compliance of livestock owners and the structure of the local production system. This could explain why only a small fraction of the official target is tested and why testing concentrates on certain areas such as the district of Kafr El Sheikh (where the government's official laboratory is based) and species kept in less mobile herds such as cattle. Lack of funding as reported by Refai [Reference Refai6] often prevents the continuation of this type of work and this may be the reason why official sampling targets were not achieved.
The quarantine, which according to the official control measures should be applied to infected farms/herds until three successive negative test results, was not consistently adhered to. Often, the finding of infection is not followed by retesting, which is particularly important for sheep and goats.
Focusing the sampling strategy and quarantine measures on specific districts like Kafr El Sheikh and specific livestock such as cattle can hinder control measures and permit the infection to persist and transmit between different localities of the governorate, especially with open markets for animal trading and an absence of the regulation of movement of animals between different regions. This may be particularly important for sheep and goats, which have been targeted with less intensity than cattle and buffaloes by the official control programme. Persistently high levels of infection in sheep and goats are also likely to have a major impact on the incidence of disease in humans, since contact with sheep and goats has been identified as being strongly associated with the risk of human infection [Reference El Sherbini11].
Using a relatively simple model, we have simulated the potential impact of different currently utilized test-and-slaughter regimes on the prevalence of brucellosis within the sheep/goat population of a village. The results of the simulations suggest that testing between 5% and 15% of animals every 1, 3 or 5 years and culling the seropositive animals would not be effective for significantly decreasing the prevalence of brucellosis in the small-ruminant population. Given our assumptions, the intensity with which infected animals are removed under the actual levels of implementation of the test-and-slaughter programme would permit brucellosis to remain endemic at a level of >8·5% of the sheep and goat population. These results are consistent with suggestions by other authors: that unless prevalence is low (⩽2%), vaccination alone or in combination with test and slaughter should be considered [Reference El Sherbini11]. With regard to the frequency of testing, the simulated scenarios showed that increasing the proportion of tested animals (when testing within a village occurred only every 3 or 5 years), resulted in a slow gradual decrease in the prevalence. When test and slaughter occurred every year, the prevalence of brucellosis decreased steadily as the proportion of tested animals increased until the prevalence reached <1% after 4 years of starting the programme when 80% of the total animal population were tested. However, in the simulation the end prevalence did not reach 0% even after testing of 100% of the population, and this may be attributed to the lack of sensitivity and specificity of serological tests. These findings highlight the importance of reaching a minimum frequency of testing and a minimum proportion of animals tested for the test-and-slaughter programme to significantly reduce the within-village prevalence of small-ruminant brucellosis.
Limited data was available for some of the input parameters of this model and a series of key simplifications have been made. Critically, due to lack of data at district level we have modelled a closed village, which is an oversimplification of the real situation as sheep and goats are mainly reared in mobile flocks which are likely to have contact with flocks from neighbouring villages. We also assumed that the newborn lambs and kids did not get infection before age 6 months as infection below this age is very rare [Reference Garin-Bastuji16], therefore lambs and kids have a minimal role in dissemination of the infection, which would not affect our final results. Lack of consideration of external sources of infection is likely to have resulted in over-optimistic end prevalence values. This further supports the finding here that the applied test-and-slaughter policy is inadequate. Sensitivity analysis demonstrated that the transmission coefficient parameter had an impact on the end prevalence of disease and accurate estimates of its value are required.
Despite its simplification of the complex dynamics of the disease and dependency on assumed data, our outcomes do not appear to be instinctively wrong and provide considerable insight on the probable disease dynamics in small-ruminant populations in the area and what can be reasonably expected from current control strategies. Although the same conclusion is likely to be reached if considering an open population with external sources of infection, a control policy for brucellosis in the region should also address between-village transmission by means of movement and market control and effective quarantine measures over infected herds. For the test-and-slaughter campaign to significantly reduce the prevalence of brucellosis, a minimum sampling fraction and frequency of testing has to be achieved. If the resources available are not enough to realistically achieve these targets, a control policy partially supported by vaccination could be a cost-effective alternative.
The veterinary authorities in Egypt and probably also other Middle Eastern countries, with high prevalence of brucellosis and similar production systems, should consider alternatives to the current brucellosis control strategies.
To our knowledge, this is the first attempt to simulate brucellosis in a small-ruminant population in the Middle Eastern context. Given the high incidence of human infection in the area [Reference Benkirane2], the likely key role of sheep and goats as a source of infection for humans, and the production losses associated with the infection [21], we believe this was a much needed exercise. Although simulation of a relatively simple and generic scenario compromises accuracy, it may help to generalize our results. The insights gained seem robust and sufficiently generic to be considered in other areas where brucellosis is endemic in small ruminants and test-and-slaughter programmes can only be realistically applied at protracted time intervals and/or low sampling fractions. The importance of carefully considering resource availability and baseline frequency of infection when such programmes are established is highlighted by our results. In endemic areas, it would be desirable that well designed surveys are conducted to provide reliable estimates of the frequency of infection. These surveys would help assess the suitability of the FAO/WHO/OIE guidelines for brucellosis control programmes in this and other areas in Middle Eastern countries. This study may provide some foundations for the establishment of such programmes in the area.
ACKNOWLEDGMENTS
We acknowledge the staff members of the General Organization of Veterinary Services in Cairo, the local Official Veterinary Directorate in Kafr El Sheikh and Dr A. Fayed (Professor of Infectious Diseases, Cairo University) for their help with the collection of data used in this study.
DECLARATION OF INTEREST
None.










