 $S$-PARTS OF TERMS OF INTEGER LINEAR RECURRENCE SEQUENCES
$S$-PARTS OF TERMS OF INTEGER LINEAR RECURRENCE SEQUENCESPublished online by Cambridge University Press: 29 November 2017
Let 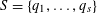 $S=\{q_{1},\ldots ,q_{s}\}$ be a finite, non-empty set of distinct prime numbers. For a non-zero integer
$S=\{q_{1},\ldots ,q_{s}\}$ be a finite, non-empty set of distinct prime numbers. For a non-zero integer 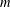 $m$, write
$m$, write  $m=q_{1}^{r_{1}}\cdots q_{s}^{r_{s}}M$, where
$m=q_{1}^{r_{1}}\cdots q_{s}^{r_{s}}M$, where 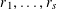 $r_{1},\ldots ,r_{s}$ are non-negative integers and
$r_{1},\ldots ,r_{s}$ are non-negative integers and 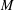 $M$ is an integer relatively prime to
$M$ is an integer relatively prime to 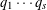 $q_{1}\cdots q_{s}$. We define the
$q_{1}\cdots q_{s}$. We define the  $S$-part
$S$-part 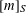 $[m]_{S}$ of
$[m]_{S}$ of 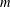 $m$ by
$m$ by 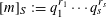 $[m]_{S}:=q_{1}^{r_{1}}\cdots q_{s}^{r_{s}}$. Let
$[m]_{S}:=q_{1}^{r_{1}}\cdots q_{s}^{r_{s}}$. Let  $(u_{n})_{n\geqslant 0}$ be a linear recurrence sequence of integers. Under certain necessary conditions, we establish that for every
$(u_{n})_{n\geqslant 0}$ be a linear recurrence sequence of integers. Under certain necessary conditions, we establish that for every 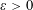 $\unicode[STIX]{x1D700}>0$, there exists an integer
$\unicode[STIX]{x1D700}>0$, there exists an integer 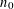 $n_{0}$ such that
$n_{0}$ such that 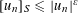 $[u_{n}]_{S}\leqslant |u_{n}|^{\unicode[STIX]{x1D700}}$ holds for
$[u_{n}]_{S}\leqslant |u_{n}|^{\unicode[STIX]{x1D700}}$ holds for  $n>n_{0}$. Our proof is ineffective in the sense that it does not give an explicit value for
$n>n_{0}$. Our proof is ineffective in the sense that it does not give an explicit value for 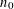 $n_{0}$. Under various assumptions on
$n_{0}$. Under various assumptions on 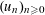 $(u_{n})_{n\geqslant 0}$, we also give effective, but weaker, upper bounds for
$(u_{n})_{n\geqslant 0}$, we also give effective, but weaker, upper bounds for  $[u_{n}]_{S}$ of the form
$[u_{n}]_{S}$ of the form 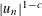 $|u_{n}|^{1-c}$, where
$|u_{n}|^{1-c}$, where  $c$ is positive and depends only on
$c$ is positive and depends only on 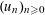 $(u_{n})_{n\geqslant 0}$ and
$(u_{n})_{n\geqslant 0}$ and 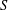 $S$.
$S$.