Published online by Cambridge University Press: 06 October 2009
Nucella lapillus and Littorina littorea afford examples of turbinately coiled shells with no space between the columella and the inner face of the whorls. Three constants are sufficient to determine the form of such shells: λ, the ratio between diameters of successive whorls; β, the semi-apical angle and ρ, the ratio of aperture length and breadth in the apertural plane passing through the axis. The variation of these three constants with size was examined and shown to be relatively small and insignificant in Nucella, but in Littorina it was significant.
For Nucella and Littorina these shell characteristics were used to relate for the nth whorl, the length along the shell spiral (ln) to the shell height (Hn), the latter being the measure usually employed in growth studies. This relation was derived mathematically and confirmed empirically as
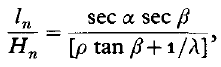
where α is the angle of the logarithmic spiral given by α = tan−1 2π sin β/loge λ. The increased resolution of the micro-growth band increments, which are measured along the shell spiral was directly related to the ln:Hn ratios.
Since the formula for the ln/Hn ratio (= shell conversion factor) includes λ, β and ρ, the three constants necessary to determine shell form in turbinately coiled shells of the type described, the shell conversion factor may be a better index of shell shape than indices based on a single constant. In species where the shell grows isometrically this ratio will remain constant, whereas changes in shell shape with size will influence this ratio. Such changes in shell shape during growth can be quantified in terms of the shell constants and when included in calculating the shell conversion factor, will show the relative magnitude of shell shape changes brought about by growth. The shell conversion factor may also be used to study the effects of ecological variations on the geometry of the shell.
The conical shell of the limpet, Patella vulgata, is geometrically a much simpler system in which direct shell measurements were used to relate length along the direction of maximum growth to the antero-posterior diameter of aperture.