No CrossRef data available.
Article contents
Three additive congruences to a large prime modulus
Part of:
Diophantine equations
Published online by Cambridge University Press: 09 April 2009
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let k ≥ 3 and n > 6k be positive integers. The equations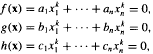 , with integer coefficients, have nontrivial p-adic solutions for all p > Ck8, where C is some positive constant. Further, for values k≥ K we can take C = 1 + O(K-½).
, with integer coefficients, have nontrivial p-adic solutions for all p > Ck8, where C is some positive constant. Further, for values k≥ K we can take C = 1 + O(K-½).
MSC classification
Secondary:
11D88: $p$-adic and power series fields
Information
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 55 , Issue 3 , December 1993 , pp. 355 - 368
- Copyright
- Copyright © Australian Mathematical Society 1993
References
Atkinson, O. D. and Cook, R. J. (1989), ‘Pairs of additive congruences to a large prime modulus’, J. Austral. Math. Soc. Series A 46, 438–455.CrossRefGoogle Scholar
Davenport, H. (1963), Analytic met hods for Diophantine equations and Diophantine inequalities, (Campus Publishers, Ann Arbor).Google Scholar
Davenport, H. and Lewis, D. J. (1969), ‘Simultaneous equations of additive type’, Philos. Trans. Roy. Soc. London Ser. A 264, 577–595.Google Scholar
Dodson, M. M. (1966), ‘Homogeneous additive congruences’, Philos. Trans. Roy. Soc. London Ser. A 261, 163–210.Google Scholar
Dörmer, E. (1990), ‘Simultaneous diagonal equations over certain p-adic fields’,J. Number Theory 36, 1–11.Google Scholar
Low, L., Pitman, J. and Wolff, A. (1988), ‘Simultaneous diagonal congruences’, J. Number Theory 29, 31–59.CrossRefGoogle Scholar
Schmid, W. M. (1984), ‘The solubility of certain p-adic equations’, J. Number Theory 19, 63–80.CrossRefGoogle Scholar
Wooley, T. D. (1990), ‘On simultaneous additive equations III’, Mathematika 37, 85–96.CrossRefGoogle Scholar

