No CrossRef data available.
Published online by Cambridge University Press: 09 April 2009
Let Nn(ω) be the number of real roots of the random algebraic equation Σnv = 0 avξv (ω)xv = 0, where the ξv(ω)'s are independent, identically distributed random variables belonging to the domain of attraction of the normal law with mean zero and P{ξv(ω) ≠ 0} > 0; also the av 's are nonzero real numbers such that (kn/tn) = 0(log n) where kn = max0≤v≤n |av| and tn = min0≤v≤n |av|. It is shown that for any sequence of positive constants (εn, n ≥ 0) satisfying εn → 0 and ε2nlog n → ∞ there is a positive constant μ so that 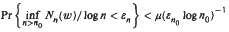 for all n0 sufficiently large.
for all n0 sufficiently large.