Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
de Giovanni, Francesco
and
Trombetti, Marco
2016.
A note on uncountable groups with modular subgroup lattice.
Archiv der Mathematik,
Vol. 107,
Issue. 6,
p.
581.
De Falco, M.
Evans, M. J.
de Giovanni, F.
and
Musella, C.
2018.
Permutability in uncountable groups.
Annali di Matematica Pura ed Applicata (1923 -),
Vol. 197,
Issue. 5,
p.
1417.
De Falco, M.
De Giovanni, F.
Musella, C.
and
Sysak, Y. P.
2019.
Uncountable groups in which normality is a transitive relation.
International Journal of Algebra and Computation,
Vol. 29,
Issue. 04,
p.
627.
De Giovanni, Francesco
and
Trombetti, Marco
2019.
Groups with restrictions on proper uncountable subgroups.
Studia Scientiarum Mathematicarum Hungarica,
Vol. 56,
Issue. 2,
p.
154.
De Falco, Maria
de Giovanni, Francesco
and
Musella, Carmela
2020.
A note on commutator subgroups in groups of large cardinality.
Monatshefte für Mathematik,
Vol. 191,
Issue. 2,
p.
249.
Ferrara, Maria
and
Trombetti, Marco
2022.
Generalized nilpotency in uncountable groups.
Forum Mathematicum,
Vol. 34,
Issue. 3,
p.
669.
Trombetti, Marco
2022.
Paranilpotency in uncountable groups.
Archiv der Mathematik,
Vol. 119,
Issue. 5,
p.
449.
FERRARA, MARIA
and
TROMBETTI, MARCO
2022.
GROUPS WITH MANY PRONORMAL SUBGROUPS.
Bulletin of the Australian Mathematical Society,
Vol. 105,
Issue. 1,
p.
75.
De Falco, Maria
Musella, Carmela
and
Trombetti, Marco
2024.
Obituary: Francesco de Giovanni (1955–2024).
Mediterranean Journal of Mathematics,
Vol. 21,
Issue. 3,
Capasso, Martina
and
Lancellotti, Liliana
2024.
Uncountable groups in which permutability is a transitive relation.
Journal of Group Theory,
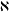 $\aleph$ in which all proper subgroups of cardinality
$\aleph$ in which all proper subgroups of cardinality 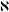 $\aleph$ are nilpotent. It is proved that such a group
$\aleph$ are nilpotent. It is proved that such a group  $G$ is nilpotent, provided that
$G$ is nilpotent, provided that 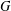 $G$ has no infinite simple homomorphic images and either
$G$ has no infinite simple homomorphic images and either 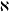 $\aleph$ has cofinality strictly larger than
$\aleph$ has cofinality strictly larger than 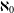 $\aleph _{0}$ or the generalized continuum hypothesis is assumed to hold. Furthermore, groups whose proper subgroups of large cardinality are soluble are studied in the last part of the paper.
$\aleph _{0}$ or the generalized continuum hypothesis is assumed to hold. Furthermore, groups whose proper subgroups of large cardinality are soluble are studied in the last part of the paper.