Article contents
Quantum-kinetic perspective on photovoltaic device operation in nanostructure-based solar cells
Published online by Cambridge University Press: 11 January 2018
Abstract
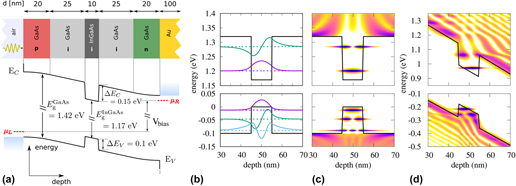
The implementation of a wide range of high-efficiency solar cell concepts is based on nanostructures with configuration-tunable optoelectronic properties. On the other hand, effective nano-optical light-trapping concepts enable the use of ultra-thin absorber architectures. In both cases, the local density of electronic and optical states deviates strongly from that of a homogeneous bulk material. At the same time, nonlocal and coherent phenomena like tunneling or ballistic transport become increasingly relevant. As a consequence, the semiclassical, diffusive bulk picture may no longer be appropriate to describe the physics of such devices. In this review, we provide a quantum-kinetic perspective on photovoltaic device operation that reaches beyond the limits of the standard simulation models for bulk solar cells. Deviations from bulk physics are assessed in ultra-thin film and nanostructure-based solar cell architectures by comparing predictions of semiclassical models with those of a more fundamental description based on nonequilibrium quantum statistical mechanics.
Keywords
Information
- Type
- Invited Review
- Information
- Copyright
- Copyright © Materials Research Society 2018
Footnotes
Contributing Editor: Sam Zhang
References
REFERENCES
- 7
- Cited by


