Article contents
Wave patterns of stationary gravity–capillary waves from a moving obstacle in a magnetic fluid
Published online by Cambridge University Press: 07 September 2022
Abstract
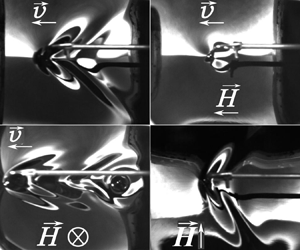
The influence of a magnetic field on the pattern of stationary waves formed on the surface of a magnetic fluid (ferrofluid) when an obstacle moves has been studied both theoretically and experimentally. It is found that a vertical magnetic field narrows the cone of stationary waves and increases their amplitude. In the wake region, the peaks of the Rosensweig instability appear in a magnetic field that is smaller than the critical field that determines this instability occurrence. A horizontal magnetic field parallel to the obstacle velocity expands the cone of waves but reduces their amplitude up to the suppression of stationary waves. A horizontal field perpendicular to the obstacle velocity also expands the cone of waves and stabilizes their amplitude.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
- 3
- Cited by


