Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Toussaint, D.
Chedevergne, F.
and
Léon, O.
2020.
Analysis of the different sources of stress acting in fully rough turbulent flows over geometrical roughness elements.
Physics of Fluids,
Vol. 32,
Issue. 7,
Khademishamami, Mahdi
and
Nardin, William
2020.
Experimental observations on fine sand winnowing from immobile gravel substrate.
Advances in Water Resources,
Vol. 142,
Issue. ,
p.
103659.
Ferreira, M.A.
and
Ganapathisubramani, B.
2021.
Scale interactions in velocity and pressure within a turbulent boundary layer developing over a staggered-cube array.
Journal of Fluid Mechanics,
Vol. 910,
Issue. ,
Tian, Guizhong
Zhu, Yesheng
Feng, Xiaoming
Zhou, Honggen
and
Zhang, Yaosheng
2021.
Investigation of the Turbulent Boundary Layer Structure over a Sparsely Spaced Biomimetic Spine-Covered Protrusion Surface.
ACS Omega,
Vol. 6,
Issue. 22,
p.
14220.
Ohta, Takashi
and
Nakatsuji, Keisuke
2021.
Spatial-scaling method and modified large eddy simulation to examine rough-wall turbulence.
Journal of Turbulence,
Vol. 22,
Issue. 7,
p.
413.
Li, Shilong
Yang, Xiaolei
Jin, Guodong
and
He, Guowei
2021.
Wall-resolved large-eddy simulation of turbulent channel flows with rough walls.
Theoretical and Applied Mechanics Letters,
Vol. 11,
Issue. 1,
p.
100228.
Gao, Jian
Agarwal, Karuna
and
Katz, Joseph
2021.
Experimental investigation of the three-dimensional flow structure around a pair of cubes immersed in the inner part of a turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 918,
Issue. ,
Cheng, Shyuan
Chamorro, Leonardo P.
and
Ansell, Phillip J.
2021.
On the H-type transition to turbulence—Laboratory experiments and reduced-order modeling.
Physics of Fluids,
Vol. 33,
Issue. 2,
Wang, Wenkang
Lozano-Durán, Adrián
Helmig, Rainer
and
Chu, Xu
2022.
Spatial and spectral characteristics of information flux between turbulent boundary layers and porous media.
Journal of Fluid Mechanics,
Vol. 949,
Issue. ,
Ganju, Sparsh
Bailey, Sean C.C.
and
Brehm, Christoph
2022.
Amplitude and wavelength scaling of sinusoidal roughness effects in turbulent channel flow at fixed.
Journal of Fluid Mechanics,
Vol. 937,
Issue. ,
Li, Shilong
Yang, Xiaolei
and
Lv, Yu
2022.
Predictive capability of the logarithmic law for roughness-modeled large-eddy simulation of turbulent channel flows with rough walls.
Physics of Fluids,
Vol. 34,
Issue. 8,
Deng, Bing-Qing
Yang, Zixuan
and
Shen, Lian
2022.
Bottom wall shear stress fluctuations in shallow-water Langmuir turbulence.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Feng, Xiaoming
Fan, Dongliang
Tian, Guizhong
and
Zhang, Yaosheng
2022.
Coupled Bionic Drag-Reducing Surface Covered by Conical Protrusions and Elastic Layer Inspired from Pufferfish Skin.
ACS Applied Materials & Interfaces,
Vol. 14,
Issue. 28,
p.
32747.
Liu, Yixun
Liu, Chun-Ho
Brasseur, Guy P.
and
Chao, Christopher Y. H.
2023.
Amplitude modulation of velocity fluctuations in the atmospheric flows over real urban morphology.
Physics of Fluids,
Vol. 35,
Issue. 2,
Sarwar, S.
Akiki, G.
Balachandar, S.
Sleigh, P. A.
and
Wright, N. G.
2024.
Direct numerical simulation of open-channel flow over a heterogeneous particle bed at low relative submergence.
Physics of Fluids,
Vol. 36,
Issue. 8,
Du, Zengzhi
Gao, An-Kang
You, Chenxi
Chen, Guo-Qing
Liu, Yongze
Li, Hongyuan
Lv, Pengyu
and
Duan, Huiling
2024.
Regulating turbulent separation by surface microstructures on a blunt plate.
Physics of Fluids,
Vol. 36,
Issue. 5,
Deshpande, Rahul
Kidanemariam, Aman G.
and
Marusic, Ivan
2024.
Pressure drag reduction via imposition of spanwise wall oscillations on a rough wall.
Journal of Fluid Mechanics,
Vol. 979,
Issue. ,
Wang, Xue
Zhou, Junjie
Liao, Wenbo
and
Yuan, Shihua
2024.
Research on Drag Reduction by Coating the Inner Wall of Hydraulic Pipeline.
Coatings,
Vol. 14,
Issue. 7,
p.
802.
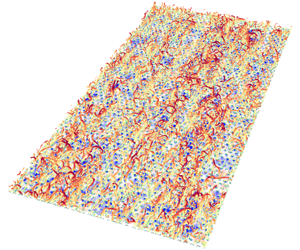
 $Re_{\unicode[STIX]{x1D70F}}=200{-}600$. For cases at
$Re_{\unicode[STIX]{x1D70F}}=200{-}600$. For cases at  $Re_{\unicode[STIX]{x1D70F}}=400$, the inner-scaled roughness height
$Re_{\unicode[STIX]{x1D70F}}=400$, the inner-scaled roughness height  $k^{+}=20$ was maintained while the spacing between roughness elements was varied from
$k^{+}=20$ was maintained while the spacing between roughness elements was varied from  $d/k=2$ to 4. Two additional rough-wall cases were performed at
$d/k=2$ to 4. Two additional rough-wall cases were performed at  $Re_{\unicode[STIX]{x1D70F}}=200$ and
$Re_{\unicode[STIX]{x1D70F}}=200$ and  $600$, where
$600$, where  $k^{+}=20$ and
$k^{+}=20$ and  $d/k=4$ were fixed to investigate the
$d/k=4$ were fixed to investigate the  $Re$ trends. For each case, wall shear stress was extracted from DNS by integrating the stress tensor over the rough surfaces. Spherical harmonics were employed to investigate the detailed spectral behaviour of the wall shear stress. Flow visualization near roughness elements was used to assist physical interpretations of the dominant flow features observed for various roughness characteristics. Analysis of amplitude modulation was applied to investigate the interactions between the ‘cell-averaged’ wall shear stress and outer, large-scale structures. A universal signal was obtained by removing the effects of outer, large-scale motions, based on the model proposed by Mathis et al. (J. Fluid Mech., vol. 715, 2013, pp. 163–180). Pre-multiplied spectra of the universal wall shear stress showed distinct behaviours at smaller scales for the ‘k-type’ roughness (
$Re$ trends. For each case, wall shear stress was extracted from DNS by integrating the stress tensor over the rough surfaces. Spherical harmonics were employed to investigate the detailed spectral behaviour of the wall shear stress. Flow visualization near roughness elements was used to assist physical interpretations of the dominant flow features observed for various roughness characteristics. Analysis of amplitude modulation was applied to investigate the interactions between the ‘cell-averaged’ wall shear stress and outer, large-scale structures. A universal signal was obtained by removing the effects of outer, large-scale motions, based on the model proposed by Mathis et al. (J. Fluid Mech., vol. 715, 2013, pp. 163–180). Pre-multiplied spectra of the universal wall shear stress showed distinct behaviours at smaller scales for the ‘k-type’ roughness ( $d/k=3{-}4$) compared to ‘d-type’ roughness (
$d/k=3{-}4$) compared to ‘d-type’ roughness ( $d/k=2$), whereas the spectra at larger scales appeared similar for both types of roughness. A scaling relation between the variance of universal wall shear stress and averaging cell dimensions was found for both ‘k-type’ and ‘d-type’ roughness, which can be useful in designing candidate wall models used in large-eddy simulation.
$d/k=2$), whereas the spectra at larger scales appeared similar for both types of roughness. A scaling relation between the variance of universal wall shear stress and averaging cell dimensions was found for both ‘k-type’ and ‘d-type’ roughness, which can be useful in designing candidate wall models used in large-eddy simulation.