Article contents
Secondary generation of breaking internal waves in confined basins by gravity currents
Published online by Cambridge University Press: 30 April 2021
Abstract
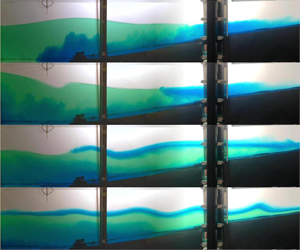
In confined stratified basins, wind forcing is an important mechanism responsible for the onset and generation of internal waves and seiches. Previous observations have also found that gravity currents in stratified environments can also initiate internal waves. We conducted a series of laboratory experiments to investigate the generation of internal motions due to such dense gravity currents on an incline entering a two-layer stratification, focusing in particular on the interaction between the onset of internal motions and topography and diapycnal mixing due to breaking internal waves. The baroclinic response of the ambient stratification to the gravity current is found to be analogous to a system forced by a surface wind stress, and the response as characterized by a Wedderburn-like number was found to be linearly proportional to the initial gravity current Richardson number. The generated internal motions are characterized as having a low-frequency internal surge and higher-frequency progressive internal waves. The overall mixing efficiency of the breaking internal wave was calculated and found to be low compared with similar previous studies.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 3
- Cited by


