Article contents
Amplification of random wave run-up on the front face of a box driven by tertiary wave interactions
Published online by Cambridge University Press: 02 May 2019
Abstract
Wave run-up phenomena driven by nonlinear wave interactions with a fixed rectangular box are investigated. Experiments are carried out in different types of uni-directional waves with normal incidence. Significant wave run-ups featuring tertiary interaction effects, similar to those reported by Molin et al. (J. Fluid Mech., vol. 528, 2005, pp. 323–354) for a fixed vertical plate, are observed in regular wave tests. Transient wave group tests are conducted for comparison, to facilitate the analysis of the tertiary interactions in irregular waves. The most striking observation is that the wave surface elevations at the centre of the front face of the fixed box can reach 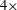 $4\times$ the incident waves even in irregular waves, much larger than the
$4\times$ the incident waves even in irregular waves, much larger than the  ${\sim}2\times$ predicted from linear theory and observed for the transient groups. The extra amplification builds up slowly and is localized on the weather side of the box. It is believed to result from tertiary interactions between the incident and reflected wave fields upstream, which induce a local lensing effect and thus wave focusing on the weather side. These interactions, though a nonlinear process, occur at the first harmonic quantities rather than high harmonics. Supporting evidence is extracted from random wave runs using NewWave analysis, where surface amplifications and phase lag – both key characteristics of tertiary wave interactions – are identified. The identification of these tertiary interactions in irregular waves is new, and may be of practical importance.
${\sim}2\times$ predicted from linear theory and observed for the transient groups. The extra amplification builds up slowly and is localized on the weather side of the box. It is believed to result from tertiary interactions between the incident and reflected wave fields upstream, which induce a local lensing effect and thus wave focusing on the weather side. These interactions, though a nonlinear process, occur at the first harmonic quantities rather than high harmonics. Supporting evidence is extracted from random wave runs using NewWave analysis, where surface amplifications and phase lag – both key characteristics of tertiary wave interactions – are identified. The identification of these tertiary interactions in irregular waves is new, and may be of practical importance.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 13
- Cited by

