Article contents
Reynolds and Mach number scaling in solenoidally-forced compressible turbulence using high-resolution direct numerical simulations
Published online by Cambridge University Press: 26 January 2016
Abstract
We report results from direct numerical simulation (DNS) of stationary compressible isotropic turbulence at very-high resolutions and a range of parameters using a massively parallel code at Taylor Reynolds numbers ( 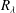 $R_{{\it\lambda}}$ ) ranging from
$R_{{\it\lambda}}$ ) ranging from 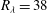 $R_{{\it\lambda}}=38$ to
$R_{{\it\lambda}}=38$ to 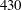 $430$ and turbulent Mach numbers (
$430$ and turbulent Mach numbers ( 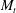 $M_{t}$ ) ranging from 0.1 to 0.6 on up to
$M_{t}$ ) ranging from 0.1 to 0.6 on up to 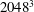 $2048^{3}$ grid resolutions. A stationary state is maintained by a stochastic solenoidal forcing at the largest scales. The focus is on the mechanisms of energy exchanges, namely, dissipation, pressure-dilatation correlation and the individual contributing variables. Compressibility effects are studied by decomposing velocity and pressure fields into solenoidal and dilatational components. We suggest a critical turbulent Mach number at about 0.3 that separate two different flow regimes – only at Mach numbers above this critical value do we observe dilatational effects to affect the flow behaviour in a qualitative manner. The equipartition of energy between the dilatational components of kinetic and potential energy, originally proposed for decaying flows at low
$2048^{3}$ grid resolutions. A stationary state is maintained by a stochastic solenoidal forcing at the largest scales. The focus is on the mechanisms of energy exchanges, namely, dissipation, pressure-dilatation correlation and the individual contributing variables. Compressibility effects are studied by decomposing velocity and pressure fields into solenoidal and dilatational components. We suggest a critical turbulent Mach number at about 0.3 that separate two different flow regimes – only at Mach numbers above this critical value do we observe dilatational effects to affect the flow behaviour in a qualitative manner. The equipartition of energy between the dilatational components of kinetic and potential energy, originally proposed for decaying flows at low 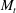 $M_{t}$ , presents significant scatter at low
$M_{t}$ , presents significant scatter at low 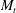 $M_{t}$ , but appears to be valid at high
$M_{t}$ , but appears to be valid at high 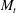 $M_{t}$ for stationary flows, which is explained by the different role of dilatational pressure in decaying and stationary flows, and at low and high
$M_{t}$ for stationary flows, which is explained by the different role of dilatational pressure in decaying and stationary flows, and at low and high 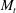 $M_{t}$ . While at low
$M_{t}$ . While at low 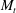 $M_{t}$ pressure possesses characteristics of solenoidal pressure, at high
$M_{t}$ pressure possesses characteristics of solenoidal pressure, at high 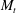 $M_{t}$ it behaves in similar ways to dilatational pressure, which results in significant changes in the dynamics of energy exchanges. This also helps explain the observed qualitative change in the skewness of pressure at high
$M_{t}$ it behaves in similar ways to dilatational pressure, which results in significant changes in the dynamics of energy exchanges. This also helps explain the observed qualitative change in the skewness of pressure at high 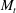 $M_{t}$ reported in the literature. Regions of high pressure are found to be correlated with regions of intense local expansions. In these regions, the density–temperature correlation is also seen to be relatively high. Classical scaling laws for low-order moments originally proposed for incompressible turbulence appear to be only weakly affected by compressibility for the range of
$M_{t}$ reported in the literature. Regions of high pressure are found to be correlated with regions of intense local expansions. In these regions, the density–temperature correlation is also seen to be relatively high. Classical scaling laws for low-order moments originally proposed for incompressible turbulence appear to be only weakly affected by compressibility for the range of 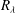 $R_{{\it\lambda}}$ and
$R_{{\it\lambda}}$ and 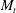 $M_{t}$ investigated.
$M_{t}$ investigated.
Information
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
- 107
- Cited by


