Published online by Cambridge University Press: 19 March 2021
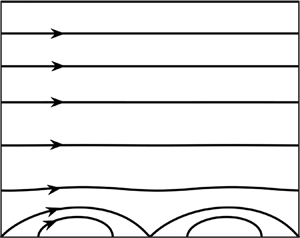
Pressure losses in laminar, pressure-gradient-driven channel flows modified by wall transpiration have been analysed in the range of Reynolds numbers guaranteeing flow stability. It was found that these losses were affected by the reduction of the effective channel opening due to formation of transpiration ‘bubbles’, by nonlinear streaming and by the elimination of direct contact between the stream and the bounding walls. It was further found that pressure losses can be reduced by properly selecting the transpiration pattern. It was determined that nonlinear streaming is the dominant effect as the transpiration wave number resulting in the largest reduction of pressure losses corresponds to the maximization of this streaming. Using transpiration at both walls further decreases pressure losses, but only when both transpiration patterns are in a proper relative position. The largest reduction of losses is achieved by concentrating transpiration at a single wave number. It is shown that the performance of finite-slot transpiration is well captured using just a few leading modes from the Fourier expansions describing the transpiration distribution. The analysis of energy expenditure shows that the use of transpiration increases the energy cost of the flow. Conditions leading to the minimization of this cost represent the most effective use of transpiration as a propulsion augmentation system.