Article contents
Isolated buoyant convection in a two-layered porous medium with an inclined permeability jump
Published online by Cambridge University Press: 09 September 2020
Abstract
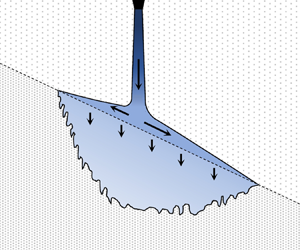
The migration of dense fluid through a saturated, layered porous medium leads to two end-member examples of buoyancy-driven flow, namely plumes and gravity currents. Herein we develop an integrated theoretical model to study this scenario for the special case where the boundary between the permeable layers, in a two-layered porous medium, is inclined at an angle to the horizontal. Far from being a routine detail, the inclination of the permeability jump leads to a symmetry-breaking: up- and downdip flows have different volume fluxes and travel different distances, possibly substantially different distances, before becoming arrested at the point where plume inflow balances basal draining. Our model predicts these associated run-out lengths and the transient approach thereto. Predictions are validated with measurements from similitude laboratory experiments, in which the upper and lower layers are comprised of glass beads of different diameters. Experiments are conducted for a range of inclination angles and also a range of plume source conditions. The experimental data suggest a complicated structure for the gravity currents, whose boundaries are blurred by dispersion in a manner not captured by our (sharp interface) model. This observation has particular significance in predicting the lateral spread of contaminated fluid through real geological formations, particularly in instances where for example groundwater contamination is of particular concern.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
- 9
- Cited by


