Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Tang, Lian
Wu, Yun-Ta
Wai, Onyx. W. H.
and
Lin, Pengzhi
2020.
Experimental Study of Turbulence and Entrained Air Characteristics in a Plunging Breaking Solitary Wave.
International Journal of Ocean and Coastal Engineering,
Vol. 03,
Issue. 01n02,
Do, Jitae
and
Chang, Kuang-An
2021.
Application of single-probe fiber optic reflectometry on phase discrimination and velocity and size determination in an oil–gas–water three-phase flow.
Measurement Science and Technology,
Vol. 32,
Issue. 10,
p.
105303.
Shi, Rui
Leng, Xinqian
and
Chanson, Hubert
2021.
Breaking bore roller characteristics: Turbulence statistics using optical techniques.
Coastal Engineering,
Vol. 168,
Issue. ,
p.
103893.
Na, Byoungjoon
and
Son, Sangyoung
2021.
Void fraction estimation using a simple combined wave gauge system under breaking waves.
Ocean Engineering,
Vol. 241,
Issue. ,
p.
110059.
Cui, Ting
He, Guanghua
Jiang, Meirong
Wang, Weizhi
Yuan, Lihao
Han, Duanfeng
Kamath, Arun
and
Bihs, Hans
2022.
Large eddy simulation of focused breaking waves with different wave steepness.
Ocean Modelling,
Vol. 179,
Issue. ,
p.
102122.
Chuang, Wei-Liang
2022.
Experimental investigation on fluid kinematics and impact pressure of flat plate impacts on pure and aerated water.
Ocean Engineering,
Vol. 266,
Issue. ,
p.
112837.
Na, Byoungjoon
and
Ko, Haeng Sik
2022.
Experimental Study on Impact Pressure at the Crown Wall of Rubble Mound Seawall and Velocity Fields using Bubble Image Velocimetry.
Journal of Korean Society of Coastal and Ocean Engineers,
Vol. 34,
Issue. 4,
p.
119.
Boettger, Daniel G.
Keating, Shane R.
Banner, Michael L.
Morison, Russel P.
and
Barthélémy, Xavier
2023.
An energetic signature for breaking inception in surface gravity waves.
Journal of Fluid Mechanics,
Vol. 959,
Issue. ,
Shi, Rui
Wüthrich, Davide
and
Chanson, Hubert
2023.
Air–water properties of unsteady breaking bores part 1: Novel Eulerian and Lagrangian velocity measurements using intrusive and non-intrusive techniques.
International Journal of Multiphase Flow,
Vol. 159,
Issue. ,
p.
104338.
Xu, Chang
and
Perlin, Marc
2023.
Parasitic waves and micro-breaking on highly nonlinear gravity–capillary waves in a convergent channel.
Journal of Fluid Mechanics,
Vol. 962,
Issue. ,
Liao, Zhiling
Zou, Qingping
Liu, Ye
Contardo, Stephanie
and
Li, Shaowu
2023.
Unified analytical solution for group-induced infragravity waves based on Green's function.
Journal of Fluid Mechanics,
Vol. 967,
Issue. ,
Tan, Ting
Ma, Yuxiang
Zhang, Jie
Niu, Xuyang
and
Chang, Kuang-An
2023.
Experimental study on flow kinematics of dam-break induced surge impacting onto a vertical wall.
Physics of Fluids,
Vol. 35,
Issue. 2,
Sou, In Mei
Wu, Yun-Ta
and
Liu, Philip L.-F.
2023.
Swash flows generated by a train of solitary waves on a planar slope.
Journal of Fluid Mechanics,
Vol. 968,
Issue. ,
Xue, Qingren
Liang, Shuxiu
Sun, Zhaochen
Xu, Yuanyuan
and
Hu, Xinyue
2023.
Quantitative research on the characteristics of flow field structures after wave-breaking in deep water.
Physics of Fluids,
Vol. 35,
Issue. 11,
Boettger, Daniel G.
Keating, Shane R.
Banner, Michael L.
Morison, Russel P.
and
Barthélémy, Xavier
2024.
Energetic inception of breaking in surface gravity waves under wind forcing.
Physical Review Fluids,
Vol. 9,
Issue. 5,
Kadari, Vinod Kumar
Dhote, Yashika
Kumar, Anil
Yewale, Nikhil
and
Dasgupta, Ratul
2024.
Wave breaking: spilling and plunging.
The European Physical Journal Special Topics,
Vol. 233,
Issue. 8-9,
p.
1685.
Chen, Jinshi
Raubenheimer, Britt
and
Elgar, Steve
2024.
Wave and Roller Transformation Over Barred Bathymetry.
Journal of Geophysical Research: Oceans,
Vol. 129,
Issue. 5,
Güner, H. Anıl Arı
2024.
Experimental investigation of flow and sediment transport on an equilibrium beach formed by surging breaker.
Applied Ocean Research,
Vol. 151,
Issue. ,
p.
104180.
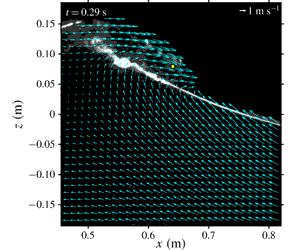
 $1.1C$ at the onset of breaking, with
$1.1C$ at the onset of breaking, with  $C$ being the phase speed of the primary breaking wave. The maximum horizontal velocity then reached
$C$ being the phase speed of the primary breaking wave. The maximum horizontal velocity then reached  $1.5C$ at approximately one-quarter of a wave period after the onset of breaking. The results also confirmed that the wavelet-educed turbulence length scale estimates are comparable to the previously reported values with different wave parameters, suggesting that the dependence of the size of energy-containing eddies on the physical scales of the breaking waves is insignificant. The measured void fraction showed a similarity profile although the measurement locations span one wavelength. The mean kinetic energy, turbulent kinetic energy, potential energy and total energy were quantified with and without the void fraction being accounted for. Results show near 60 % and 40 % overestimates of the kinetic energy and the potential energy if the void fraction is not considered. After correcting the density variation due to air entrainment, the total energy dissipated following an exponential decay, with 43 % and 65 % energy being dissipated at one and two wavelengths downstream from the breaking point, respectively. The equipartition assumption was found to be applicable before and during the entire breaking process in the present spilling breakers.
$1.5C$ at approximately one-quarter of a wave period after the onset of breaking. The results also confirmed that the wavelet-educed turbulence length scale estimates are comparable to the previously reported values with different wave parameters, suggesting that the dependence of the size of energy-containing eddies on the physical scales of the breaking waves is insignificant. The measured void fraction showed a similarity profile although the measurement locations span one wavelength. The mean kinetic energy, turbulent kinetic energy, potential energy and total energy were quantified with and without the void fraction being accounted for. Results show near 60 % and 40 % overestimates of the kinetic energy and the potential energy if the void fraction is not considered. After correcting the density variation due to air entrainment, the total energy dissipated following an exponential decay, with 43 % and 65 % energy being dissipated at one and two wavelengths downstream from the breaking point, respectively. The equipartition assumption was found to be applicable before and during the entire breaking process in the present spilling breakers.