Article contents
GORENSTEIN MODULES AND GORENSTEIN MODEL STRUCTURES
Published online by Cambridge University Press: 27 February 2017
Abstract
Given a complete hereditary cotorsion pair 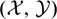 $(\mathcal{X}, \mathcal{Y})$ , we introduce the concept of
$(\mathcal{X}, \mathcal{Y})$ , we introduce the concept of 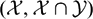 $(\mathcal{X}, \mathcal{X} \cap \mathcal{Y})$ -Gorenstein projective modules and study its stability properties. As applications, we first get two model structures related to Gorenstein flat modules over a right coherent ring. Secondly, for any non-negative integer n, we construct a cofibrantly generated model structure on Mod(R) in which the class of fibrant objects are the modules of Gorenstein injective dimension ≤ n over a left Noetherian ring R. Similarly, if R is a left coherent ring in which all flat left R-modules have finite projective dimension, then there is a cofibrantly generated model structure on Mod(R) such that the cofibrant objects are the modules of Gorenstein projective dimension ≤ n. These structures have their analogous in the category of chain complexes.
$(\mathcal{X}, \mathcal{X} \cap \mathcal{Y})$ -Gorenstein projective modules and study its stability properties. As applications, we first get two model structures related to Gorenstein flat modules over a right coherent ring. Secondly, for any non-negative integer n, we construct a cofibrantly generated model structure on Mod(R) in which the class of fibrant objects are the modules of Gorenstein injective dimension ≤ n over a left Noetherian ring R. Similarly, if R is a left coherent ring in which all flat left R-modules have finite projective dimension, then there is a cofibrantly generated model structure on Mod(R) such that the cofibrant objects are the modules of Gorenstein projective dimension ≤ n. These structures have their analogous in the category of chain complexes.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2017
References
REFERENCES
- 7
- Cited by

