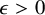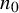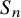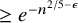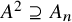1 Introduction
This paper employs tools from analysis of Boolean functions to address problems studied independently by group theorists and combinatorialists. The problems we study are those which can be reformulated as investigations about independent sets in Cayley graphs over symmetric groups.
1.1 Covering numbers of subsets of symmetric groups
The covering number of a generating set A in a group G is the minimal
![]() $\ell $
such that
$\ell $
such that
![]() $A^{\ell } = G.$
The problem of determining the covering numbers of conjugacy classes and their unions is fundamental in group theory, with highlights including the breakthroughs of Guralnick, Larsen, Liebeck, Shalev and Tiep [Reference Guralnick, Larsen and Tiep12, Reference Larsen and Shalev24, Reference Liebeck and Shalev26, Reference Shalev30].
$A^{\ell } = G.$
The problem of determining the covering numbers of conjugacy classes and their unions is fundamental in group theory, with highlights including the breakthroughs of Guralnick, Larsen, Liebeck, Shalev and Tiep [Reference Guralnick, Larsen and Tiep12, Reference Larsen and Shalev24, Reference Liebeck and Shalev26, Reference Shalev30].
A particular question that has been studied extensively is characterizing sets A such that
![]() $A^2=G$
. A well-known open problem in this area is Thompson’s conjecture which asserts that every finite simple group G contains a conjugacy class whose square is G.
$A^2=G$
. A well-known open problem in this area is Thompson’s conjecture which asserts that every finite simple group G contains a conjugacy class whose square is G.
Much of the research on characterizing sets whose square is the entire group has focused on the symmetric group, where this study goes back to Gleason, who showed in 1962 that for any n-cycle
![]() $\sigma \in S_n$
, the conjugacy class
$\sigma \in S_n$
, the conjugacy class
![]() $\sigma ^{S_n}$
satisfies
$\sigma ^{S_n}$
satisfies
![]() $(\sigma ^{S_n})^2 = A_n$
(see [Reference Husemoller13, Proposition 4]). For many years, results of this kind were achieved only for very restricted families of conjugacy classes, like the case where
$(\sigma ^{S_n})^2 = A_n$
(see [Reference Husemoller13, Proposition 4]). For many years, results of this kind were achieved only for very restricted families of conjugacy classes, like the case where
![]() $\sigma $
consists of two cycles (see, for example, [Reference Bertram1, Reference Brenner2, Reference Vishne32]). In a breakthrough paper from 2007, Larsen and Shalev [Reference Larsen and Shalev24] showed that for a sufficiently large n, if
$\sigma $
consists of two cycles (see, for example, [Reference Bertram1, Reference Brenner2, Reference Vishne32]). In a breakthrough paper from 2007, Larsen and Shalev [Reference Larsen and Shalev24] showed that for a sufficiently large n, if
![]() $\sigma \in S_n$
has at most
$\sigma \in S_n$
has at most
![]() $n^{1/128}$
cycles, then
$n^{1/128}$
cycles, then
![]() $(\sigma ^{S_n})^2 = A_n$
. As a random permutation
$(\sigma ^{S_n})^2 = A_n$
. As a random permutation
![]() $\sigma \in S_n$
has
$\sigma \in S_n$
has
![]() $O(\log n)$
cycles a.a.s., this shows that asymptotically,
$O(\log n)$
cycles a.a.s., this shows that asymptotically,
![]() $(\sigma ^{S_n})^2 = A_n$
holds for almost all permutations. In another breakthrough which followed shortly after, Larsen and Shalev [Reference Larsen and Shalev23] proved the same assertion for any
$(\sigma ^{S_n})^2 = A_n$
holds for almost all permutations. In another breakthrough which followed shortly after, Larsen and Shalev [Reference Larsen and Shalev23] proved the same assertion for any
![]() $\sigma \in S_n$
that has at most
$\sigma \in S_n$
that has at most
![]() $n^{1/4-\epsilon }$
cycles. Namely, they showed the following:
$n^{1/4-\epsilon }$
cycles. Namely, they showed the following:
Theorem 1.1 [Reference Larsen and Shalev23, Theorem 1.10].
For any
![]() $\epsilon>0,$
there exists an integer
$\epsilon>0,$
there exists an integer
![]() $n_0$
, such that for any
$n_0$
, such that for any
![]() $n>n_0$
and for any
$n>n_0$
and for any
![]() $\sigma \in S_n$
that has at most
$\sigma \in S_n$
that has at most
![]() $n^{1/4-\epsilon }$
cycles, we have
$n^{1/4-\epsilon }$
cycles, we have
![]() $(\sigma ^{S_n})^{2} = A_n.$
$(\sigma ^{S_n})^{2} = A_n.$
The number of cycles of a permutation is closely related to the density of its conjugacy class. (Throughout the paper, for finite sets
![]() $A,B$
, the density of A inside B is
$A,B$
, the density of A inside B is
![]() $\mu _B(A) = \frac {|A\cap B|}{|B|}$
, and when B is clear from the context, we shorten the notation to
$\mu _B(A) = \frac {|A\cap B|}{|B|}$
, and when B is clear from the context, we shorten the notation to
![]() $\mu (A)$
). Theorem 1.1 can be easily seen to be equivalent to the following:
$\mu (A)$
). Theorem 1.1 can be easily seen to be equivalent to the following:
Theorem 1.2 [Reference Larsen and Shalev23, Theorem 1.20].
For any
![]() $\epsilon>0,$
there exists an integer
$\epsilon>0,$
there exists an integer
![]() $n_0$
, such that for any
$n_0$
, such that for any
![]() $n>n_0$
and for any normal subset
$n>n_0$
and for any normal subset
![]() $A \subset S_n$
with
$A \subset S_n$
with
![]() $\mu (A)\ge e^{-n^{1/4 - \epsilon }}$
, we have
$\mu (A)\ge e^{-n^{1/4 - \epsilon }}$
, we have
![]() $A^2 \supseteq A_n.$
$A^2 \supseteq A_n.$
Determining the minimal density
![]() $\alpha (n)$
such that for any normal subset of
$\alpha (n)$
such that for any normal subset of
![]() $S_n$
with density
$S_n$
with density
![]() $\geq \alpha (n)$
we have
$\geq \alpha (n)$
we have
![]() $A^2\supseteq A_n$
, remains a very challenging open problem, and the results of [Reference Larsen and Shalev23] remained the ‘state of the art’ in the last 15 years (see, for example, [Reference Shalev31]).
$A^2\supseteq A_n$
, remains a very challenging open problem, and the results of [Reference Larsen and Shalev23] remained the ‘state of the art’ in the last 15 years (see, for example, [Reference Shalev31]).
1.1.1 Our results
We show that the assertions of Theorems 1.1 and 1.2 hold under a significantly weaker assumption on the set A.
Theorem 1.3. For any
![]() $\epsilon>0,$
there exists an integer
$\epsilon>0,$
there exists an integer
![]() $n_0$
, such that for any
$n_0$
, such that for any
![]() $n>n_0$
and for any
$n>n_0$
and for any
![]() $\sigma \in S_n$
that has at most
$\sigma \in S_n$
that has at most
![]() $n^{2/5-\epsilon }$
cycles, we have
$n^{2/5-\epsilon }$
cycles, we have
![]() $(\sigma ^{S_n})^{2} = A_n.$
$(\sigma ^{S_n})^{2} = A_n.$
Theorem 1.4. For any
![]() $\epsilon>0$
, there exists an integer
$\epsilon>0$
, there exists an integer
![]() $n_0$
, such that for any
$n_0$
, such that for any
![]() $n>n_0$
and for any normal subset
$n>n_0$
and for any normal subset
![]() $A \subset S_n$
with
$A \subset S_n$
with
![]() $\mu (A)\ge e^{-n^{2/5 - \epsilon }}$
, we have
$\mu (A)\ge e^{-n^{2/5 - \epsilon }}$
, we have
![]() $A^2 \supseteq A_n.$
$A^2 \supseteq A_n.$
We also prove a similar strengthening of the corresponding result for subsets of
![]() $A_n$
that was recently proved by Larsen and Tiep [Reference Larsen and Tiep25].
$A_n$
that was recently proved by Larsen and Tiep [Reference Larsen and Tiep25].
Theorem 1.5. For any
![]() $\epsilon>0$
, there exists an integer
$\epsilon>0$
, there exists an integer
![]() $n_0$
, such that for any
$n_0$
, such that for any
![]() $n>n_0$
and for any normal subset
$n>n_0$
and for any normal subset
![]() $A \subset A_n$
with
$A \subset A_n$
with
![]() $\mu (A)\ge e^{-n^{2/5 - \epsilon }}$
, we have
$\mu (A)\ge e^{-n^{2/5 - \epsilon }}$
, we have
![]() $A^2 \supseteq A_n \setminus \{1\}.$
$A^2 \supseteq A_n \setminus \{1\}.$
Theorem 1.5 significantly improves over a recent result of Lifshitz and Marmor [Reference Lifshitz and Marmor28, Corollary 2.11], which achieves the weaker conclusion
![]() $A^3=A_n$
under the stronger assumption
$A^3=A_n$
under the stronger assumption
![]() $\mu (A)\ge e^{-n^{1/3 - \epsilon }}$
.
$\mu (A)\ge e^{-n^{1/3 - \epsilon }}$
.
In terms of techniques, Larsen and Shalev [Reference Larsen and Shalev23, Reference Larsen and Shalev24] obtained their results by establishing upper bounds for the values of irreducible characters. Those character bounds have grown out to be fundamental to the study of covering numbers and have found various applications in other areas of mathematics. Our new results demonstrate the surprising role of a very different new tool – the recent result of Keevash and Lifshitz [Reference Keevash and Lifshitz16] on hypercontractivity for global functions over symmetric groups.
Regarding tightness of our results, we believe that the minimal density of A which guarantees
![]() $A^2 \supseteq A_n$
is significantly smaller than
$A^2 \supseteq A_n$
is significantly smaller than
![]() $e^{-n^{2/5 - \epsilon }}$
. In this context, it is worth noting that Garonzi and Maróti [Reference Garonzi and Maróti10] conjectured that there exists an absolute constant
$e^{-n^{2/5 - \epsilon }}$
. In this context, it is worth noting that Garonzi and Maróti [Reference Garonzi and Maróti10] conjectured that there exists an absolute constant
![]() $c>0$
, such that if
$c>0$
, such that if
![]() $A,B,C$
are normal subsets of an alternating group
$A,B,C$
are normal subsets of an alternating group
![]() $G=A_n$
of density
$G=A_n$
of density
![]() $\ge |G|^{-c}$
, then
$\ge |G|^{-c}$
, then
![]() $ABC=G$
. They achieved an essentially best possible result for four sets by showing that for any
$ABC=G$
. They achieved an essentially best possible result for four sets by showing that for any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 = n_0(\epsilon )$
, such that if
$n_0 = n_0(\epsilon )$
, such that if
![]() $n>n_0$
and
$n>n_0$
and
![]() $A,B,C,D$
are normal sets of density
$A,B,C,D$
are normal sets of density
![]() $\ge |G|^{-1/2 + \epsilon }$
, then
$\ge |G|^{-1/2 + \epsilon }$
, then
![]() $ABCD = G.$
Lifshitz and Marmor [Reference Lifshitz and Marmor28] speculated that a far-reaching generalization of Theorem 1.4 holds: If A is a normal subset of
$ABCD = G.$
Lifshitz and Marmor [Reference Lifshitz and Marmor28] speculated that a far-reaching generalization of Theorem 1.4 holds: If A is a normal subset of
![]() $S_n$
of density
$S_n$
of density
![]() $\ge (n!)^{-c},$
then
$\ge (n!)^{-c},$
then
![]() $A^2 \supseteq A_n$
.
$A^2 \supseteq A_n$
.
1.2 Independent sets in normal Cayley graphs
Theorem 1.2 can be restated in a graph theoretic terminology. Recall that a subset of the vertices of a graph is independent if it does not contain any edges. The largest size of an independent set in a graph is called its independence number. A Cayley graph
![]() $\mathrm {Cay}(G,A)$
is said to be normal if the set A is normal. For a set
$\mathrm {Cay}(G,A)$
is said to be normal if the set A is normal. For a set
![]() $I\subseteq S_n$
and for
$I\subseteq S_n$
and for
![]() $\tau \in S_n$
, it is easy to see that
$\tau \in S_n$
, it is easy to see that
![]() $\tau \notin I^{-1}I$
if and only if I is an independent set in the Cayley graph
$\tau \notin I^{-1}I$
if and only if I is an independent set in the Cayley graph
![]() $\mathrm {Cay}(S_n, \tau ^{S_n}).$
Since for a normal set
$\mathrm {Cay}(S_n, \tau ^{S_n}).$
Since for a normal set
![]() $I\subseteq S_n$
, we have
$I\subseteq S_n$
, we have
![]() $I^{-1}I=I^2$
, it is clear that the following theorem is a restatement of Theorem 1.2.
$I^{-1}I=I^2$
, it is clear that the following theorem is a restatement of Theorem 1.2.
Theorem 1.6 (Theorem 1.2 restated).
For any
![]() $\epsilon>0,$
there exists an integer
$\epsilon>0,$
there exists an integer
![]() $n_0$
, such that for any
$n_0$
, such that for any
![]() $n>n_0$
and for any
$n>n_0$
and for any
![]() $\tau \in A_n \setminus \{1\},$
the largest normal independent set in the Cayley graph
$\tau \in A_n \setminus \{1\},$
the largest normal independent set in the Cayley graph
![]() $\mathrm {Cay}(A_n,\tau ^{S_n})$
has size at most
$\mathrm {Cay}(A_n,\tau ^{S_n})$
has size at most
![]() $e^{-n^{1/4-\epsilon }}$
.
$e^{-n^{1/4-\epsilon }}$
.
The size of the largest normal independent set in
![]() $\mathrm {Cay}(A_n,\tau ^{S_n})$
is clearly bounded by the independence number of
$\mathrm {Cay}(A_n,\tau ^{S_n})$
is clearly bounded by the independence number of
![]() $\mathrm {Cay}(A_n,\tau ^{S_n})$
. A subfield of extremal combinatorics known as Erdős–Ko–Rado type theorems (see the book [Reference Godsil and Meagher11] and the thesis [Reference Filmus7]) is mostly devoted to the study of the independence numbers of graphs that that have a large group of symmetries. One breakthrough in this direction is the work of Ellis, Friedgut and Pilpel [Reference Ellis, Friedgut and Pilpel4] concerning the independence number of the Cayley graph
$\mathrm {Cay}(A_n,\tau ^{S_n})$
. A subfield of extremal combinatorics known as Erdős–Ko–Rado type theorems (see the book [Reference Godsil and Meagher11] and the thesis [Reference Filmus7]) is mostly devoted to the study of the independence numbers of graphs that that have a large group of symmetries. One breakthrough in this direction is the work of Ellis, Friedgut and Pilpel [Reference Ellis, Friedgut and Pilpel4] concerning the independence number of the Cayley graph
![]() $\mathrm {Cay}(S_n, A)$
, where A is the set of permutations with at most
$\mathrm {Cay}(S_n, A)$
, where A is the set of permutations with at most
![]() $t-1$
fixed points. Independent sets I in
$t-1$
fixed points. Independent sets I in
![]() $\mathrm {Cay}(A_n, A)$
are called t-intersecting, as in such a set I, any two permutations agree on at least t coordinates.
$\mathrm {Cay}(A_n, A)$
are called t-intersecting, as in such a set I, any two permutations agree on at least t coordinates.
Ellis, Friedgut and Pilpel showed that for any
![]() $n>n_0(t)$
, the largest t-intersecting sets in
$n>n_0(t)$
, the largest t-intersecting sets in
![]() $S_n$
are the t-umvirates, which are cosets of the subgroup of all permutations that fix a given set of size t. The minimal possible value of
$S_n$
are the t-umvirates, which are cosets of the subgroup of all permutations that fix a given set of size t. The minimal possible value of
![]() $n_0(t)$
was improved by Ellis and Lifshitz [Reference Ellis and Lifshitz6], then by Kupavskii and Zakharov [Reference Kupavskii and Zakharov22], and finally by Keller, Lifshitz, Minzer and Sheinfeld [Reference Keller, Lifshitz, Minzer and Sheinfeld20] who showed that
$n_0(t)$
was improved by Ellis and Lifshitz [Reference Ellis and Lifshitz6], then by Kupavskii and Zakharov [Reference Kupavskii and Zakharov22], and finally by Keller, Lifshitz, Minzer and Sheinfeld [Reference Keller, Lifshitz, Minzer and Sheinfeld20] who showed that
![]() $n_0(t)$
can be taken to be linear in t. Furthermore, the authors of [Reference Ellis and Lifshitz6, Reference Kupavskii and Zakharov22] showed that the results of Ellis, Friedgut and Pilpel extend to the sparser Cayley graph
$n_0(t)$
can be taken to be linear in t. Furthermore, the authors of [Reference Ellis and Lifshitz6, Reference Kupavskii and Zakharov22] showed that the results of Ellis, Friedgut and Pilpel extend to the sparser Cayley graph
![]() $\mathrm {Cay}(G,A'),$
where
$\mathrm {Cay}(G,A'),$
where
![]() $A'$
consists only of the permutations that have exactly
$A'$
consists only of the permutations that have exactly
![]() $t-1$
fixed points (though, starting at a larger value of
$t-1$
fixed points (though, starting at a larger value of
![]() $n_0(t)$
). The latter setting is known as the ‘forbidding one intersection’ problem; see [Reference Ellis, Keller and Lifshitz5].
$n_0(t)$
). The latter setting is known as the ‘forbidding one intersection’ problem; see [Reference Ellis, Keller and Lifshitz5].
When removing edges from a Cayley graph, its family of independent sets widens, making it increasingly challenging to establish effective upper bounds on the independence number. We prove the following result regarding the independence number of significantly sparser Cayley graphs, in which the generating set is a single conjugacy class.Footnote
1
In order to avoid sign issues, we restrict our attention to the alternating group
![]() $A_n$
.
$A_n$
.
Theorem 1.7. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $\delta ,n_0$
, such that the following holds for any
$\delta ,n_0$
, such that the following holds for any
![]() $t\in \mathbb {N}$
and
$t\in \mathbb {N}$
and
![]() $n>n_0+t$
. Let
$n>n_0+t$
. Let
![]() $\sigma \in A_n$
be a permutation with t fixed points. Then the largest independent set in the Cayley graph
$\sigma \in A_n$
be a permutation with t fixed points. Then the largest independent set in the Cayley graph
![]() $\mathrm {Cay}(A_n,\sigma ^{S_n})$
has density of at most
$\mathrm {Cay}(A_n,\sigma ^{S_n})$
has density of at most
![]() $\max (e^{-(n-t)^{1/3-\epsilon }}, (n-t)^{-\delta t})$
.
$\max (e^{-(n-t)^{1/3-\epsilon }}, (n-t)^{-\delta t})$
.
For
![]() $t<n^{1/3 -\epsilon }$
, Theorem 1.7 implies that the independence number of the Cayley graph
$t<n^{1/3 -\epsilon }$
, Theorem 1.7 implies that the independence number of the Cayley graph
![]() $\mathrm {Cay}(A_n,\sigma ^{S_n})$
is
$\mathrm {Cay}(A_n,\sigma ^{S_n})$
is
![]() $n^{-\Theta (t)}$
, as in this range, the assertion matches the trivial lower bound implied by the t-umvirates. Thus, the theorem shows that in terms of the order of magnitude, the results of [Reference Ellis and Lifshitz6, Reference Kupavskii and Zakharov22] for the ‘forbidding one intersection’ problem extend to the much sparser setting where only intersection inside a single conjugacy class is forbidden.
$n^{-\Theta (t)}$
, as in this range, the assertion matches the trivial lower bound implied by the t-umvirates. Thus, the theorem shows that in terms of the order of magnitude, the results of [Reference Ellis and Lifshitz6, Reference Kupavskii and Zakharov22] for the ‘forbidding one intersection’ problem extend to the much sparser setting where only intersection inside a single conjugacy class is forbidden.
For larger values of t, our bound improves upon the bound of Larsen and Shalev in two ways. First, our bound holds for all independent sets, while their bound applies only to normal independent sets. Moreover, even in the broader context of arbitrary independent sets in normal Cayley graphs, we improve the
![]() $1/4$
in the double exponent to
$1/4$
in the double exponent to
![]() $1/3$
.
$1/3$
.
Our main tool, which is interesting for its own sake, is the following stability result which says that a mild lower bound on the density of an independent set suffices to imply that it is heavily correlated with a t-umvirate. Given a set A, we write
![]() $\mu _A$
for the uniform measure on A.
$\mu _A$
for the uniform measure on A.
Theorem 1.8. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $\delta ,n_0$
, such that the following holds for any
$\delta ,n_0$
, such that the following holds for any
![]() $t\in \mathbb {N}$
and
$t\in \mathbb {N}$
and
![]() $n>n_0+t$
. Let
$n>n_0+t$
. Let
![]() $\sigma \in A_n$
be a permutation with t fixed points. Suppose that I is an independent set in the Cayley graph
$\sigma \in A_n$
be a permutation with t fixed points. Suppose that I is an independent set in the Cayley graph
![]() $\mathrm {Cay}(A_n,\sigma ^{S_n})$
of density
$\mathrm {Cay}(A_n,\sigma ^{S_n})$
of density
![]() $\ge e^{-n^{1/2 - \frac {\log _n t}{2} - \epsilon }}$
. Then there exists
$\ge e^{-n^{1/2 - \frac {\log _n t}{2} - \epsilon }}$
. Then there exists
![]() $\ell>0$
and an
$\ell>0$
and an
![]() $\ell $
-umvirate U, such that
$\ell $
-umvirate U, such that
1.3 Our methods: Hypercontractivity and bounds for the isotypic projections
Our proof combines character bounds with a recent tool known as ‘sharp hypercontractivity in the symmetric group’ due to Keevash and Lifshitz [Reference Keevash and Lifshitz16], which improves upon the earlier work of Filmus, Kindler, Lifshitz and Minzer [Reference Filmus, Kindler, Lifshitz and Minzer8].
The covering results of Larsen and Shalev are based upon character bounds. These can be used to show that conjugacy classes behave (in some senses) like random sets of the same density. Hypercontractivity serves a similar role to the character bounds for functions that are not necessarily class functions. We make use of this by applying it to study the restrictions of the conjugacy classes to the
![]() $\ell $
-umvirates (for various values of
$\ell $
-umvirates (for various values of
![]() $\ell $
). These restrictions satisfy the following spreadness notion (see [Reference Kupavskii and Zakharov22]), which is also known as globalness or quasiregularity in the literature (see [Reference Keevash, Lifshitz, Long and Minzer17, Reference Keller and Lifshitz18]).
$\ell $
). These restrictions satisfy the following spreadness notion (see [Reference Kupavskii and Zakharov22]), which is also known as globalness or quasiregularity in the literature (see [Reference Keevash, Lifshitz, Long and Minzer17, Reference Keller and Lifshitz18]).
Let
![]() $\delta>0$
. We say that a set
$\delta>0$
. We say that a set
![]() $A\subseteq S_n$
is
$A\subseteq S_n$
is
![]() $\delta $
-spread if for each
$\delta $
-spread if for each
![]() $\ell \ge 1$
and for each
$\ell \ge 1$
and for each
![]() $\ell $
-umvirate U,
$\ell $
-umvirate U,
In words, this means that no restriction to an
![]() $\ell $
-umvirate increases the density of A significantly.
$\ell $
-umvirate increases the density of A significantly.
Theorem 1.8 can be restated as an upper bound on the size of
![]() $\delta $
-spread independent sets in normal Cayley graphs. It lies in the heart of the paper, and the rest of our theorems are reduced to it by combinatorial arguments.
$\delta $
-spread independent sets in normal Cayley graphs. It lies in the heart of the paper, and the rest of our theorems are reduced to it by combinatorial arguments.
Sketch of proof for Theorem 1.8
For functions
![]() $f,g$
on a finite group G, we write
$f,g$
on a finite group G, we write
where
![]() $x\sim A$
denotes that x is chosen uniformly out of A. Denote by
$x\sim A$
denotes that x is chosen uniformly out of A. Denote by
![]() $\hat {G}$
the set of irreducible characters on G. For
$\hat {G}$
the set of irreducible characters on G. For
![]() $\chi \in \hat {G}$
, we write
$\chi \in \hat {G}$
, we write
![]() $f^{=\chi } = \chi (1) f * \chi .$
It is well known that f can be orthogonally decomposed as
$f^{=\chi } = \chi (1) f * \chi .$
It is well known that f can be orthogonally decomposed as
![]() $f = \sum _{\chi \in \hat {G}} f^{= \chi }$
. We denote the space of functions of the form
$f = \sum _{\chi \in \hat {G}} f^{= \chi }$
. We denote the space of functions of the form
![]() $f^{=\chi }$
by
$f^{=\chi }$
by
![]() $W_{\chi }$
.
$W_{\chi }$
.
Fix
![]() $\sigma \in A_n$
and write
$\sigma \in A_n$
and write
![]() $f = \frac {1_{(\sigma ^{S_n})}}{\mu _{A_n}(\sigma ^{S_n})}$
. It was known already to Frobenius that since f is a class function, for any
$f = \frac {1_{(\sigma ^{S_n})}}{\mu _{A_n}(\sigma ^{S_n})}$
. It was known already to Frobenius that since f is a class function, for any
![]() $\chi \in \hat {G}$
, the space
$\chi \in \hat {G}$
, the space
![]() $W_{\chi }$
is an eigenspace of the convolution operator
$W_{\chi }$
is an eigenspace of the convolution operator
![]() $g \mapsto f * g$
, which corresponds to the eigenvalue
$g \mapsto f * g$
, which corresponds to the eigenvalue
![]() $\frac {\chi (\sigma )}{\chi (1)}$
.
$\frac {\chi (\sigma )}{\chi (1)}$
.
Let
![]() $g=\frac {1_I}{\mu _{A_n}(I)}$
be the normalized indicator of an independent set I in the Cayley graph
$g=\frac {1_I}{\mu _{A_n}(I)}$
be the normalized indicator of an independent set I in the Cayley graph
![]() $\mathrm {Cay}(A_n,\sigma ^{S_n})$
. Then one can decompose
$\mathrm {Cay}(A_n,\sigma ^{S_n})$
. Then one can decompose
 $$ \begin{align} 0 = \langle f*g, g\rangle = \sum_{\chi\in \widehat{A_n}}\frac{\chi(\sigma)}{\chi(1)}\|g^{=\chi}\|_2^{2}. \end{align} $$
$$ \begin{align} 0 = \langle f*g, g\rangle = \sum_{\chi\in \widehat{A_n}}\frac{\chi(\sigma)}{\chi(1)}\|g^{=\chi}\|_2^{2}. \end{align} $$
The ‘main term’ of the above sum comes from the trivial representation
![]() $\chi = 1$
, which contributes
$\chi = 1$
, which contributes
![]() $\langle g, 1\rangle = \mathbb {E}[g] = 1$
to the sum. We proceed by showing that if
$\langle g, 1\rangle = \mathbb {E}[g] = 1$
to the sum. We proceed by showing that if
![]() $\sigma $
has t fixed points and I is ‘large’ (as a function of t) and
$\sigma $
has t fixed points and I is ‘large’ (as a function of t) and
![]() $\delta $
-spread, then the other terms are negligible compared to the main term, leading to a contradiction.
$\delta $
-spread, then the other terms are negligible compared to the main term, leading to a contradiction.
Our proof is divided into two parts – upper bounding the terms
![]() $\left |\frac {\chi (\sigma )}{\chi (1)}\right |$
and upper bounding the terms
$\left |\frac {\chi (\sigma )}{\chi (1)}\right |$
and upper bounding the terms
![]() $\|g^{=\chi }\|_2^2$
, for all
$\|g^{=\chi }\|_2^2$
, for all
![]() $\chi \in \widehat {A_n}\setminus \{1\}.$
To upper bound the terms
$\chi \in \widehat {A_n}\setminus \{1\}.$
To upper bound the terms
![]() $\left |\frac {\chi (\sigma )}{\chi (1)}\right |$
, we use the character bounds of Larsen–Shalev [Reference Larsen and Shalev23] and Larsen–Tiep [Reference Larsen and Tiep25] that take the form
$\left |\frac {\chi (\sigma )}{\chi (1)}\right |$
, we use the character bounds of Larsen–Shalev [Reference Larsen and Shalev23] and Larsen–Tiep [Reference Larsen and Tiep25] that take the form
![]() $\chi (\sigma )\le \chi (1)^{\beta },$
where
$\chi (\sigma )\le \chi (1)^{\beta },$
where
![]() $\beta $
depends only on
$\beta $
depends only on
![]() $\sigma $
and not on
$\sigma $
and not on
![]() $\chi $
. The main novel tool that we introduce in this paper is the following proposition which allows upper bounding the terms
$\chi $
. The main novel tool that we introduce in this paper is the following proposition which allows upper bounding the terms
![]() $\|g^{=\chi }\|_2^2$
.
$\|g^{=\chi }\|_2^2$
.
Proposition 1.9. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $\delta ,n_0>0$
, such that the following holds for all
$\delta ,n_0>0$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $\alpha <1-\epsilon $
and let
$\alpha <1-\epsilon $
and let
![]() $A\subseteq S_n$
be a
$A\subseteq S_n$
be a
![]() $\delta $
-spread set of density
$\delta $
-spread set of density
![]() $\ge e^{-n^{\alpha }}$
. Write
$\ge e^{-n^{\alpha }}$
. Write
![]() $g = \frac {1_A}{\mu (A)}$
. Then
$g = \frac {1_A}{\mu (A)}$
. Then
![]() $\|g^{=\chi }\|_2^2\le \chi (1)^{\alpha +\epsilon }$
for any
$\|g^{=\chi }\|_2^2\le \chi (1)^{\alpha +\epsilon }$
for any
![]() $\chi \in \hat {G}$
.
$\chi \in \hat {G}$
.
We prove Proposition 1.9 by appealing to the hypercontractivity theorem of Keevash and Lifshitz [Reference Keevash and Lifshitz16].
Combining the Larsen–Shalev and Larsen–Tiep bounds with ours, while choosing
![]() $\alpha $
appropriately, we obtain that
$\alpha $
appropriately, we obtain that
![]() $|\langle f*g,g \rangle - 1| \le \sum _{\chi \in \widehat {A_n}\setminus 1} \chi (1)^{-s}$
for an absolute constant
$|\langle f*g,g \rangle - 1| \le \sum _{\chi \in \widehat {A_n}\setminus 1} \chi (1)^{-s}$
for an absolute constant
![]() $s>0$
. At this point, we apply the Witten zeta function estimates of Liebeck and Shalev. For a finite group G, the Witten zeta function is given by
$s>0$
. At this point, we apply the Witten zeta function estimates of Liebeck and Shalev. For a finite group G, the Witten zeta function is given by
![]() $\zeta _G(s) = \sum _{\chi \in \hat {G}}\chi (1)^{-s}.$
Liebeck and Shalev [Reference Liebeck and Shalev27] showed that
$\zeta _G(s) = \sum _{\chi \in \hat {G}}\chi (1)^{-s}.$
Liebeck and Shalev [Reference Liebeck and Shalev27] showed that
![]() $\zeta _{A_n}(s) = 1+o(1)$
for any fixed
$\zeta _{A_n}(s) = 1+o(1)$
for any fixed
![]() $s>0$
, as n tends to infinity. This estimate yields
$s>0$
, as n tends to infinity. This estimate yields
![]() $|\langle f*g,g \rangle - 1| =o(1)$
in contradiction to Equation (1.1), thus completing the proof.Footnote
2
$|\langle f*g,g \rangle - 1| =o(1)$
in contradiction to Equation (1.1), thus completing the proof.Footnote
2
We deduce Theorems 1.3, 1.4 and 1.5 from Proposition 1.9 by proving that certain restricted conjugacy classes are
![]() $\delta $
-spread for an absolute constant
$\delta $
-spread for an absolute constant
![]() $\delta>0$
, and then following a similar route to the above sketch.
$\delta>0$
, and then following a similar route to the above sketch.
Structure of the paper
In Section 2, we present results from works of Larsen–Shalev [Reference Larsen and Shalev23], Larsen–Tiep [Reference Larsen and Tiep25] and Liebeck–Shalev [Reference Liebeck and Shalev27] that will be used in the sequel.
In Section 3, we prove Proposition 1.9. In Section 4, we prove a key theorem (Theorem 4.2) and deduce from it Theorems 1.7 and 1.8. In Section 5, we prove that certain restricted conjugacy classes admit some form of spreadness. In Section 6, we prove Theorems 1.3, 1.4 and 1.5.
2 Preliminaries from the Works of Larsen–Shalev, Larsen–Tiep, and Liebeck–Shalev
2.1 Character bounds using the parameter
 $E(\sigma )$
$E(\sigma )$
Recall that given a finite group G, we write
![]() $\widehat {G}$
for the set of its irreducible complex characters. Larsen and Shalev [Reference Larsen and Shalev23] introduced the parameter
$\widehat {G}$
for the set of its irreducible complex characters. Larsen and Shalev [Reference Larsen and Shalev23] introduced the parameter
![]() $E(\sigma )$
, defined as follows.
$E(\sigma )$
, defined as follows.
Definition 2.1. For
![]() $\sigma \in S_n$
, let
$\sigma \in S_n$
, let
![]() $f_{\sigma }(i)$
be the number of i-cycles in its cycle decomposition. Define the orbit growth sequence
$f_{\sigma }(i)$
be the number of i-cycles in its cycle decomposition. Define the orbit growth sequence
![]() $e_1,e_2,\ldots ,e_n$
via the equality
$e_1,e_2,\ldots ,e_n$
via the equality
 $$\begin{align*}e_1 + \cdots + e_k := \max \left(\frac{\log\left(\sum_{i=1}^k i \cdot f_{\sigma}(i)\right)}{\log n},0\right),\end{align*}$$
$$\begin{align*}e_1 + \cdots + e_k := \max \left(\frac{\log\left(\sum_{i=1}^k i \cdot f_{\sigma}(i)\right)}{\log n},0\right),\end{align*}$$
for each
![]() $1 \le k \le n$
. The function
$1 \le k \le n$
. The function
![]() $E(\sigma )$
is defined by
$E(\sigma )$
is defined by
 $$\begin{align*}E(\sigma):= \sum_{i=1}^n \frac{e_i}{i}.\end{align*}$$
$$\begin{align*}E(\sigma):= \sum_{i=1}^n \frac{e_i}{i}.\end{align*}$$
The main result of Larsen and Shalev [Reference Larsen and Shalev23] is the following character bound.
Theorem 2.2 [Reference Larsen and Shalev23, Theorem 1.1].
For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N},$
such that the following holds. Let
$n_0 \in \mathbb {N},$
such that the following holds. Let
![]() $n>n_0$
, let
$n>n_0$
, let
![]() $\chi $
be an irreducible character of
$\chi $
be an irreducible character of
![]() $S_n$
, and let
$S_n$
, and let
![]() $\sigma \in S_n$
. Then
$\sigma \in S_n$
. Then
We also make use of the following character bound of Larsen and Tiep [Reference Larsen and Tiep25].
Theorem 2.3 [Reference Larsen and Tiep25, Theorem 2].
For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
such that the following holds. Let
$n_0 \in \mathbb {N}$
such that the following holds. Let
![]() $n>n_0$
and suppose that
$n>n_0$
and suppose that
![]() $\sigma \in A_n$
satisfies
$\sigma \in A_n$
satisfies
![]() $\sigma ^{A_n}\ne \sigma ^{S_n}.$
Then for every character
$\sigma ^{A_n}\ne \sigma ^{S_n}.$
Then for every character
![]() $\chi $
of
$\chi $
of
![]() $A_n$
, we have
$A_n$
, we have
![]() $|\chi (\sigma )| \le \chi (1)^{\epsilon }.$
$|\chi (\sigma )| \le \chi (1)^{\epsilon }.$
These bounds combine to yield the following variant of Theorem 2.2 for
![]() $A_n$
.
$A_n$
.
Theorem 2.4. For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N},$
such that the following holds. Let
$n_0 \in \mathbb {N},$
such that the following holds. Let
![]() $n>n_0$
, let
$n>n_0$
, let
![]() $\chi $
be an irreducible character of
$\chi $
be an irreducible character of
![]() $A_n$
, and let
$A_n$
, and let
![]() $\sigma \in A_n$
. Then
$\sigma \in A_n$
. Then
Proof. Recall from the representation theory of
![]() $S_n$
and
$S_n$
and
![]() $A_n$
that every irreducible character
$A_n$
that every irreducible character
![]() $\chi $
of
$\chi $
of
![]() $S_n$
is either irreducible when restricted to
$S_n$
is either irreducible when restricted to
![]() $A_n$
or is the sum of two irreducible characters
$A_n$
or is the sum of two irreducible characters
![]() $\chi _1,\chi _2$
, such that
$\chi _1,\chi _2$
, such that
![]() $\chi _2(\sigma ) = \chi _1((12)\sigma (12))$
for all
$\chi _2(\sigma ) = \chi _1((12)\sigma (12))$
for all
![]() $\sigma \in A_n.$
Moreover, any irreducible character of
$\sigma \in A_n.$
Moreover, any irreducible character of
![]() $A_n$
can be obtained from an irreducible character of
$A_n$
can be obtained from an irreducible character of
![]() $S_n$
in one of these two ways.
$S_n$
in one of these two ways.
It follows that whenever
![]() $\sigma ^{A_n}= \sigma ^{S_n}$
, we have
$\sigma ^{A_n}= \sigma ^{S_n}$
, we have
![]() $\chi _1(\sigma ) = \chi _2(\sigma ) = \chi (\sigma )/2$
, and the assertion follows from Theorem 2.2. Otherwise, by Theorem 2.3, we have
$\chi _1(\sigma ) = \chi _2(\sigma ) = \chi (\sigma )/2$
, and the assertion follows from Theorem 2.2. Otherwise, by Theorem 2.3, we have
![]() $|\chi (\sigma )| \le \chi (1)^{\epsilon },$
which implies the assertion.
$|\chi (\sigma )| \le \chi (1)^{\epsilon },$
which implies the assertion.
2.2 Upper bounds for
 $E(\sigma )$
$E(\sigma )$
We now give several simple estimates for
![]() $E(\sigma )$
. First, we treat the case where
$E(\sigma )$
. First, we treat the case where
![]() $\sigma $
has t fixed points.
$\sigma $
has t fixed points.
Lemma 2.5. For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
such that the following holds for all
$n_0 \in \mathbb {N}$
such that the following holds for all
![]() $n>n_0$
. Suppose that
$n>n_0$
. Suppose that
![]() $\sigma \in S_n$
has t fixed points. Then
$\sigma \in S_n$
has t fixed points. Then
![]() $E(\sigma )\le \frac {1 + \log _{n}t}{2}$
.
$E(\sigma )\le \frac {1 + \log _{n}t}{2}$
.
Proof. Let
![]() $e_i$
be as in the definition of
$e_i$
be as in the definition of
![]() $E(\sigma )$
. We have
$E(\sigma )$
. We have
 $$ \begin{align*} E(\sigma) = \sum_{i=1}^n e_i/i = e_1 + \sum_{i=2}^n e_i/i \le e_1 +\frac{\sum_{i=2}^n e_i}{2} = e_1 + \frac{1-e_1}{2} = \frac{1+e_1}{2}=\frac{1 + \log_{n}t}{2}. \end{align*} $$
$$ \begin{align*} E(\sigma) = \sum_{i=1}^n e_i/i = e_1 + \sum_{i=2}^n e_i/i \le e_1 +\frac{\sum_{i=2}^n e_i}{2} = e_1 + \frac{1-e_1}{2} = \frac{1+e_1}{2}=\frac{1 + \log_{n}t}{2}. \end{align*} $$
We now treat the case where
![]() $\sigma $
has
$\sigma $
has
![]() $n^{o(1)}\ i$
-cycles for each ‘small’ i.
$n^{o(1)}\ i$
-cycles for each ‘small’ i.
Lemma 2.6. For any
![]() $\epsilon>0$
and any
$\epsilon>0$
and any
![]() $m \in \mathbb {N}$
, there exist
$m \in \mathbb {N}$
, there exist
![]() $\delta>0$
and
$\delta>0$
and
![]() $n_0 \in \mathbb {N}$
such that the following holds. Let
$n_0 \in \mathbb {N}$
such that the following holds. Let
![]() $n>n_0$
and suppose that
$n>n_0$
and suppose that
![]() $\sigma \in S_n$
has at most
$\sigma \in S_n$
has at most
![]() $n^{\delta }\ i$
-cycles for each
$n^{\delta }\ i$
-cycles for each
![]() $i < m$
. Then
$i < m$
. Then
![]() $E(\sigma ) \le 1/m +\epsilon .$
$E(\sigma ) \le 1/m +\epsilon .$
Proof. We have
 $$ \begin{align*} E(\sigma) = \sum_{i=1}^n e_i/i =\sum_{i=1}^{m-1} e_i/i +\sum_{i=m}^n e_i/i \le \sum_{i=1}^{m-1} \frac{\delta+\frac{i}{\log n}}{i} + \frac{\sum_{i=m}^n e_i}{m}\\ \le \delta \cdot 2\log(m) +m/\log n + 1/m \le 1/m + \epsilon.\\[-40pt] \end{align*} $$
$$ \begin{align*} E(\sigma) = \sum_{i=1}^n e_i/i =\sum_{i=1}^{m-1} e_i/i +\sum_{i=m}^n e_i/i \le \sum_{i=1}^{m-1} \frac{\delta+\frac{i}{\log n}}{i} + \frac{\sum_{i=m}^n e_i}{m}\\ \le \delta \cdot 2\log(m) +m/\log n + 1/m \le 1/m + \epsilon.\\[-40pt] \end{align*} $$
Another estimate for
![]() $E(\sigma )$
that we need is the following.
$E(\sigma )$
that we need is the following.
Lemma 2.7. For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
, such that the following holds for all
$n_0 \in \mathbb {N}$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $0<\alpha <1$
. Suppose that
$0<\alpha <1$
. Suppose that
![]() $\sigma \in S_n$
has no fixed points and has at most
$\sigma \in S_n$
has no fixed points and has at most
![]() $n^\alpha $
cycles of length at most
$n^\alpha $
cycles of length at most
![]() $\lceil 2/\epsilon \rceil $
. Then
$\lceil 2/\epsilon \rceil $
. Then
![]() $E(\sigma )\leq \alpha /2+\epsilon /2 + \frac {\log (2/\epsilon )}{\log (n)}$
.
$E(\sigma )\leq \alpha /2+\epsilon /2 + \frac {\log (2/\epsilon )}{\log (n)}$
.
Proof. We have
 $$ \begin{align*} \begin{split} E(\sigma) &= \sum_{i=1}^n e_i/i = \sum_{i=2}^{\lfloor 2/\epsilon \rfloor} e_i/i + \sum_{i=\lfloor 2/\epsilon \rfloor +1}^n e_i/i \le \frac{\sum_{i=2}^{\lfloor 2/\epsilon \rfloor} e_i}{2} + \frac{\sum_{i=\lfloor 2/\epsilon \rfloor +1}^n e_i}{2/\epsilon} \\ &\le \frac{\alpha}{2} + \frac{\log(2/\epsilon)}{\log(n)}+\frac{\epsilon}{2}, \end{split} \end{align*} $$
$$ \begin{align*} \begin{split} E(\sigma) &= \sum_{i=1}^n e_i/i = \sum_{i=2}^{\lfloor 2/\epsilon \rfloor} e_i/i + \sum_{i=\lfloor 2/\epsilon \rfloor +1}^n e_i/i \le \frac{\sum_{i=2}^{\lfloor 2/\epsilon \rfloor} e_i}{2} + \frac{\sum_{i=\lfloor 2/\epsilon \rfloor +1}^n e_i}{2/\epsilon} \\ &\le \frac{\alpha}{2} + \frac{\log(2/\epsilon)}{\log(n)}+\frac{\epsilon}{2}, \end{split} \end{align*} $$
where the last inequality holds since
![]() $e_2+\ldots +e_{\lfloor 2/\epsilon \rfloor } \le \alpha +\frac {\log (2/\epsilon )}{\log (n)}$
.
$e_2+\ldots +e_{\lfloor 2/\epsilon \rfloor } \le \alpha +\frac {\log (2/\epsilon )}{\log (n)}$
.
A similar argument yields the following lemma:
Lemma 2.8. For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
, such that the following holds for all
$n_0 \in \mathbb {N}$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $\alpha>0$
. Suppose that
$\alpha>0$
. Suppose that
![]() $\sigma \in S_n$
has at most
$\sigma \in S_n$
has at most
![]() $n^{\alpha }$
cycles. Then
$n^{\alpha }$
cycles. Then
![]() $E(\sigma ) \le \alpha +\epsilon $
.
$E(\sigma ) \le \alpha +\epsilon $
.
Proof. We have
 $$ \begin{align*} E(\sigma) = \sum_{i=1}^n e_i/i \le \sum_{i=1}^{\lfloor n^{\epsilon/2} \rfloor} e_i + \sum_{i=\lfloor n^{\epsilon/2} \rfloor +1}^n e_i/n^{\epsilon/2} \le \alpha+\epsilon/2+n^{-\epsilon/2} \le \alpha+\epsilon.\\[-48pt] \end{align*} $$
$$ \begin{align*} E(\sigma) = \sum_{i=1}^n e_i/i \le \sum_{i=1}^{\lfloor n^{\epsilon/2} \rfloor} e_i + \sum_{i=\lfloor n^{\epsilon/2} \rfloor +1}^n e_i/n^{\epsilon/2} \le \alpha+\epsilon/2+n^{-\epsilon/2} \le \alpha+\epsilon.\\[-48pt] \end{align*} $$
2.3 Squares of conjugacy classes
We use several results on squares of conjugacy classes in
![]() $A_n$
and in
$A_n$
and in
![]() $S_n$
, of Larsen and Shalev [Reference Larsen and Shalev23] and of Larsen and Tiep [Reference Larsen and Tiep25].
$S_n$
, of Larsen and Shalev [Reference Larsen and Shalev23] and of Larsen and Tiep [Reference Larsen and Tiep25].
Theorem 2.9 [Reference Larsen and Tiep25, Theorem 3].
There exists
![]() $n_0\in \mathbb {N}$
, such that the following holds for all
$n_0\in \mathbb {N}$
, such that the following holds for all
![]() $n>n_0$
. Suppose that
$n>n_0$
. Suppose that
![]() $\sigma \in A_n$
satisfies
$\sigma \in A_n$
satisfies
![]() $\sigma ^{A_n}\ne \sigma ^{S_n}$
. Then
$\sigma ^{A_n}\ne \sigma ^{S_n}$
. Then
Theorem 2.10 [Reference Larsen and Shalev23, Theorem 5.1].
For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
such that the following holds for all
$n_0 \in \mathbb {N}$
such that the following holds for all
![]() $n>n_0$
. Suppose that for some
$n>n_0$
. Suppose that for some
![]() $\sigma \in S_n,\tau \in A_n$
, we have
$\sigma \in S_n,\tau \in A_n$
, we have
![]() $2E(\sigma ) + E(\tau ) < 1-\epsilon $
. Then
$2E(\sigma ) + E(\tau ) < 1-\epsilon $
. Then
![]() $\tau \in (\sigma ^{S_n})^{2}.$
$\tau \in (\sigma ^{S_n})^{2}.$
Theorem 2.11 [Reference Larsen and Shalev23, Theorem 1.10].
For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
such that the following holds for all
$n_0 \in \mathbb {N}$
such that the following holds for all
![]() $n>n_0$
. Suppose that
$n>n_0$
. Suppose that
![]() $\sigma \in S_n$
has no fixed points, at most
$\sigma \in S_n$
has no fixed points, at most
![]() $n^{1-\epsilon }$
2-cycles and at most
$n^{1-\epsilon }$
2-cycles and at most
![]() $(1/4 - \epsilon )n$
cycles overall. Then
$(1/4 - \epsilon )n$
cycles overall. Then
![]() $\left (\sigma ^{S_n}\right )^2=A_n.$
$\left (\sigma ^{S_n}\right )^2=A_n.$
For integers
![]() $n,m$
such that
$n,m$
such that
![]() $m|n$
, we denote by
$m|n$
, we denote by
![]() $(m^{n/m})$
the conjugacy class of all
$(m^{n/m})$
the conjugacy class of all
![]() $\sigma \in S_n$
that consist of
$\sigma \in S_n$
that consist of
![]() $n/m\ m$
-cycles.
$n/m\ m$
-cycles.
Theorem 2.12 [Reference Larsen and Shalev23, Theorem 1.12].
There exists
![]() $n_0 \in \mathbb {N}$
such that for any
$n_0 \in \mathbb {N}$
such that for any
![]() $n>n_0$
and for any
$n>n_0$
and for any
![]() $m \ge 4$
that divides n, we have
$m \ge 4$
that divides n, we have
![]() $(m^{n/m})^{2} = A_n.$
$(m^{n/m})^{2} = A_n.$
We also make use of the following result due to Vishne [Reference Vishne32].
Theorem 2.13 [Reference Vishne32, Theorem 3.2].
For any even
![]() $n \in \mathbb {N}$
, the set
$n \in \mathbb {N}$
, the set
![]() $(2^{n/2})^2$
consists of the permutations that have an even number of i-cycles for each i.
$(2^{n/2})^2$
consists of the permutations that have an even number of i-cycles for each i.
2.4 The Witten zeta function
As was described in the introduction, we apply a result of Liebeck and Shalev [Reference Liebeck and Shalev27] concerning the Witten zeta function.
Recall that the Witten zeta function for a finite group G is defined by
 $$\begin{align*}\zeta(s) = \zeta_G(s) = \sum_{\chi \in \hat{G}} \chi(1)^{-s}. \end{align*}$$
$$\begin{align*}\zeta(s) = \zeta_G(s) = \sum_{\chi \in \hat{G}} \chi(1)^{-s}. \end{align*}$$
Theorem 2.14 [Reference Liebeck and Shalev27, Theorem 1.1, Corollary 2.7].
For any
![]() $\epsilon ,s>0$
, there exists
$\epsilon ,s>0$
, there exists
![]() $n_0$
such that for any
$n_0$
such that for any
![]() $n>n_0,$
we have
$n>n_0,$
we have
 $$\begin{align*}2-\epsilon \le \sum_{\chi \in \widehat{S_n}}\chi(1)^{-s} \leq 2+\epsilon, \qquad \mbox{and} \qquad 1-\epsilon \le \sum_{\chi \in \widehat{A_n}}\chi(1)^{-s} \leq 1+\epsilon. \end{align*}$$
$$\begin{align*}2-\epsilon \le \sum_{\chi \in \widehat{S_n}}\chi(1)^{-s} \leq 2+\epsilon, \qquad \mbox{and} \qquad 1-\epsilon \le \sum_{\chi \in \widehat{A_n}}\chi(1)^{-s} \leq 1+\epsilon. \end{align*}$$
3 From hypercontractivity to bounds for the finer isotypic decomposition
In this section, we prove Proposition 1.9. Let us recall its statement.
Proposition 1.9. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $\delta ,n_0>0$
, such that the following holds for all
$\delta ,n_0>0$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $\alpha <1-\epsilon $
and let
$\alpha <1-\epsilon $
and let
![]() $A\subseteq S_n$
be a
$A\subseteq S_n$
be a
![]() $\delta $
-spread set of density
$\delta $
-spread set of density
![]() $\ge e^{-n^{\alpha }}$
. Write
$\ge e^{-n^{\alpha }}$
. Write
![]() $g = \frac {1_A}{\mu (A)}$
. Then
$g = \frac {1_A}{\mu (A)}$
. Then
![]() $\|g^{=\chi }\|_2^2\le \chi (1)^{\alpha +\epsilon }$
for any
$\|g^{=\chi }\|_2^2\le \chi (1)^{\alpha +\epsilon }$
for any
![]() $\chi \in \hat {S_n}$
.
$\chi \in \hat {S_n}$
.
In the proof, we use a hypercontractive inequality of Keevash and Lifshitz for global functions over symmetric groups, as well as standard estimates for the dimensions of the characters.
3.1 The level-d inequality of Keevash and Lifshitz
Level-d inequalities bound the
![]() $L_2$
norm of certain ‘chunks’ of the orthogonal decomposition of a function, using hypercontractivity. The first level-d inequality was obtained in 1988 by Kahn, Kalai and Linial [Reference Kahn, Kalai and Linial14], for Boolean functions over the discrete cube
$L_2$
norm of certain ‘chunks’ of the orthogonal decomposition of a function, using hypercontractivity. The first level-d inequality was obtained in 1988 by Kahn, Kalai and Linial [Reference Kahn, Kalai and Linial14], for Boolean functions over the discrete cube
![]() $\{-1,1\}^n$
endowed with the uniform measure. It asserts that for any
$\{-1,1\}^n$
endowed with the uniform measure. It asserts that for any
![]() $f:\{-1,1\}^n \to \{0,1\}$
with
$f:\{-1,1\}^n \to \{0,1\}$
with
![]() $\mathbb {E}[f]=\alpha $
and for any
$\mathbb {E}[f]=\alpha $
and for any
![]() $d \leq 2\ln (1/\alpha )$
, the coefficients of the Fourier-Walsh expansion of f (namely,
$d \leq 2\ln (1/\alpha )$
, the coefficients of the Fourier-Walsh expansion of f (namely,
![]() $f=\sum _{S \subset [n]} \hat f(S)\chi _S$
) satisfy
$f=\sum _{S \subset [n]} \hat f(S)\chi _S$
) satisfy
![]() $||\sum _{|S|=d} \hat f(S)\chi _S||_2^2 \leq (2e/d)^d \alpha ^2 \ln (1/\alpha )^d$
(see [Reference O’Donnell29, Chapter 9]). Level-d inequalities turned out to be very useful, and have diverse applications.
$||\sum _{|S|=d} \hat f(S)\chi _S||_2^2 \leq (2e/d)^d \alpha ^2 \ln (1/\alpha )^d$
(see [Reference O’Donnell29, Chapter 9]). Level-d inequalities turned out to be very useful, and have diverse applications.
In [Reference Keevash, Lifshitz, Long and Minzer17], Keevash, Lifshitz, Long and Minzer (see also Khot, Minzer and Safra [Reference Khot, Minzer and Safra21]) showed that level-d inequalities can be obtained in much more general settings under the additional assumption that the function is ‘global’ (or ‘spread’) – i.e., that no restriction of
![]() $O(1)$
coordinates can increase its
$O(1)$
coordinates can increase its
![]() $L_2$
-norm significantly. Filmus, Kindler, Lifshitz and Minzer [Reference Filmus, Kindler, Lifshitz and Minzer8] were the first to use the technique of Keevash et al. to obtain a level-d inequality for global functions over symmetric groups. Here, we use a sharp level-d inequality which was recently proved by Keevash and Lifshitz [Reference Keevash and Lifshitz16], building upon a sharp version of the inequality of Keevash et al. that was obtained by Keller, Lifshitz and Marcus [Reference Keller, Lifshitz and Marcus19].
$L_2$
-norm significantly. Filmus, Kindler, Lifshitz and Minzer [Reference Filmus, Kindler, Lifshitz and Minzer8] were the first to use the technique of Keevash et al. to obtain a level-d inequality for global functions over symmetric groups. Here, we use a sharp level-d inequality which was recently proved by Keevash and Lifshitz [Reference Keevash and Lifshitz16], building upon a sharp version of the inequality of Keevash et al. that was obtained by Keller, Lifshitz and Marcus [Reference Keller, Lifshitz and Marcus19].
In order to state the level-d inequality due to Keevash and Lifshitz [Reference Keevash and Lifshitz16], we need a few more notations. The terminology we use follows [Reference Ellis, Friedgut and Pilpel4] in providing a degree decomposition for the symmetric group, which corresponds to the decomposition of the Fourier-Walsh expansion over the discrete cube into ‘degree levels’
![]() $f^{=d}=\sum _{|S|=d} \hat f(S)\chi _S$
that appears in the original level-d inequality.
$f^{=d}=\sum _{|S|=d} \hat f(S)\chi _S$
that appears in the original level-d inequality.
A dictator
![]() $U_{i\to j}$
is the set of permutations that send i to j. The intersection of d distinct dictators is called a d-umvirate if it is nonempty. The d-umvirates correspond to pairs of d-tuples
$U_{i\to j}$
is the set of permutations that send i to j. The intersection of d distinct dictators is called a d-umvirate if it is nonempty. The d-umvirates correspond to pairs of d-tuples
![]() $I,J$
and we denote by
$I,J$
and we denote by
![]() $U_{I\to J}$
the set of permutations sending the tuple I to the tuple J. The restriction of a function f to a d-umvirate
$U_{I\to J}$
the set of permutations sending the tuple I to the tuple J. The restriction of a function f to a d-umvirate
![]() $U_{I \to J}$
is denoted by
$U_{I \to J}$
is denoted by
![]() $f_{I\to J}$
and is called a d-restriction. We write
$f_{I\to J}$
and is called a d-restriction. We write
![]() $\|f_{I\to J}\|_p$
for the
$\|f_{I\to J}\|_p$
for the
![]() $L_p$
-norm of f with respect to the uniform measure on the d-umvirate
$L_p$
-norm of f with respect to the uniform measure on the d-umvirate
![]() $U_{I\to J}$
.
$U_{I\to J}$
.
A function f is said to be r-global if
![]() $\|f_{I\to J}\|_2 \le r^{|I|}\|f\|_2$
for all d-restrictions
$\|f_{I\to J}\|_2 \le r^{|I|}\|f\|_2$
for all d-restrictions
![]() $f_{I\to J}$
, for all values of d. A set A is r-global if its indicator function is r-global. Note that a set A is
$f_{I\to J}$
, for all values of d. A set A is r-global if its indicator function is r-global. Note that a set A is
![]() $\delta $
-spread if and only if it is
$\delta $
-spread if and only if it is
![]() $n^{\delta }$
-global.Footnote
3
$n^{\delta }$
-global.Footnote
3
For a partition
![]() $\lambda =(\lambda _1,\lambda _2,\ldots ,\lambda _t) \vdash n$
, the strict level of a representation
$\lambda =(\lambda _1,\lambda _2,\ldots ,\lambda _t) \vdash n$
, the strict level of a representation
![]() $V_{\lambda }$
that corresponds to
$V_{\lambda }$
that corresponds to
![]() $\lambda $
is
$\lambda $
is
![]() $n-\lambda _1$
. The level of
$n-\lambda _1$
. The level of
![]() $V_{\lambda }$
is the minimum between the strict levels of
$V_{\lambda }$
is the minimum between the strict levels of
![]() $V_{\lambda }$
and
$V_{\lambda }$
and
![]() $V_{\lambda '}$
, where
$V_{\lambda '}$
, where
![]() $\lambda '$
is the conjugate partition of n. The space of matrix coefficients of an irreducible representation V is the space spanned by the functions
$\lambda '$
is the conjugate partition of n. The space of matrix coefficients of an irreducible representation V is the space spanned by the functions
![]() $f_{v,\varphi }\colon G\to \mathbb {C}$
indexed by
$f_{v,\varphi }\colon G\to \mathbb {C}$
indexed by
![]() $v\in V,\varphi \in V^*$
that are given by
$v\in V,\varphi \in V^*$
that are given by
We write
![]() $W_d$
for the sum of the spaces of matrix coefficients for all representations of level d, and denote by
$W_d$
for the sum of the spaces of matrix coefficients for all representations of level d, and denote by
![]() $f^{\approx d}$
the projection of f onto
$f^{\approx d}$
the projection of f onto
![]() $W_{d}$
.
$W_{d}$
.
Keevash and Lifshitz proved the following:
Theorem 3.1 [Reference Keevash and Lifshitz16, Theorem 4.1].
There exists
![]() $C>0$
, such that for any
$C>0$
, such that for any
![]() $n \in \mathbb {N}$
and for any
$n \in \mathbb {N}$
and for any
![]() $r>1$
, if
$r>1$
, if
![]() $A \subseteq S_n$
is r-global and
$A \subseteq S_n$
is r-global and
![]() $d\le \min (\tfrac {1}{8}\log (1/\mu (A)), 10^{-5}n)$
, then
$d\le \min (\tfrac {1}{8}\log (1/\mu (A)), 10^{-5}n)$
, then
3.2 Proof of Proposition 1.9
Recall that any irreducible character
![]() $\chi $
is the trace of a unique irreducible representation
$\chi $
is the trace of a unique irreducible representation
![]() $\rho $
, and that we have
$\rho $
, and that we have
![]() $\chi (1)=\mathrm {dim}(\rho )$
. We say that the level of an irreducible character
$\chi (1)=\mathrm {dim}(\rho )$
. We say that the level of an irreducible character
![]() $\chi $
is the level of the unique irreducible representation that corresponds to it.
$\chi $
is the level of the unique irreducible representation that corresponds to it.
In the proof, we use the following lower bounds on the dimensions of low level irreducible representations.
Lemma 3.2 [Reference Ellis, Friedgut and Pilpel4, Claim 1 and Theorem 19].
There exists
![]() $n_0 \in \mathbb {N}$
, such that the following holds for all
$n_0 \in \mathbb {N}$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $d\le n/200$
and let
$d\le n/200$
and let
![]() $\chi $
be an irreducible character of
$\chi $
be an irreducible character of
![]() $S_n$
of level
$S_n$
of level
![]() $\ge d$
. Then
$\ge d$
. Then
![]() $\chi (1) \ge \left (\frac {n}{ed}\right )^d.$
$\chi (1) \ge \left (\frac {n}{ed}\right )^d.$
Proof of Proposition 1.9.
Let
![]() $A\subseteq S_n$
be a
$A\subseteq S_n$
be a
![]() $\delta $
-spread set of density
$\delta $
-spread set of density
![]() $\ge e^{-n^{\alpha }}$
, let
$\ge e^{-n^{\alpha }}$
, let
![]() $g = \frac {1_A}{\mu (A)}$
, and let
$g = \frac {1_A}{\mu (A)}$
, and let
![]() $\chi $
be a character of level d. We may assume w.l.o.g. that
$\chi $
be a character of level d. We may assume w.l.o.g. that
![]() $\mu (A)=e^{-n^{\alpha }}$
. We consider two cases:
$\mu (A)=e^{-n^{\alpha }}$
. We consider two cases:
-
1.
 $d \geq \min (10^{-5}n, \frac {1}{8}n^{\alpha })$
. In this case, we may upper bound
$d \geq \min (10^{-5}n, \frac {1}{8}n^{\alpha })$
. In this case, we may upper bound
 $\|g^{=\chi }\|_2 \le \|g\|_2 = e^{n^{\alpha }} \leq e^{n^{1-\epsilon }}$
, while by Lemma 3.2, for a sufficiently large n, we have
$\|g^{=\chi }\|_2 \le \|g\|_2 = e^{n^{\alpha }} \leq e^{n^{1-\epsilon }}$
, while by Lemma 3.2, for a sufficiently large n, we have
 $\chi (1) \ge \min (\left (\frac {n}{ed}\right )^d , \left (\frac {200}{e}\right )^{n/200}).$
This implies that the statement of the proposition holds provided that
$\chi (1) \ge \min (\left (\frac {n}{ed}\right )^d , \left (\frac {200}{e}\right )^{n/200}).$
This implies that the statement of the proposition holds provided that
 $n_0$
is sufficiently large.
$n_0$
is sufficiently large. -
2.
 $d \leq \min (10^{-5}n, \frac {1}{8}n^{\alpha })$
. In this case, we may apply Theorem 3.1 to obtain and by Lemma 3.2, the right-hand side is smaller than
$d \leq \min (10^{-5}n, \frac {1}{8}n^{\alpha })$
. In this case, we may apply Theorem 3.1 to obtain and by Lemma 3.2, the right-hand side is smaller than $$\begin{align*}\|g^{= \chi}\|_2^2\le \mu(A)^2 \left( C n^{4\delta} d^{-1} \log (1/\mu(A)) \right)^d \le \left( Cd^{-1} n^{\alpha + 4\delta} \right)^d,\end{align*}$$
$$\begin{align*}\|g^{= \chi}\|_2^2\le \mu(A)^2 \left( C n^{4\delta} d^{-1} \log (1/\mu(A)) \right)^d \le \left( Cd^{-1} n^{\alpha + 4\delta} \right)^d,\end{align*}$$
 $\chi (1)^{\alpha +\epsilon }$
, provided that
$\chi (1)^{\alpha +\epsilon }$
, provided that
 $\delta $
is sufficiently small and
$\delta $
is sufficiently small and
 $n_0$
is sufficiently large.
$n_0$
is sufficiently large.
This completes the proof.
3.3 Bounds for the finer isotypic decomposition over
 $A_n$
$A_n$
We shall make use also of the following variant of Proposition 1.9 for
![]() $A_n$
.
$A_n$
.
Proposition 3.3. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $\delta ,n_0>0$
, such that the following holds for all
$\delta ,n_0>0$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $\alpha <1-\epsilon $
and let
$\alpha <1-\epsilon $
and let
![]() $A\subseteq A_n$
be a
$A\subseteq A_n$
be a
![]() $\delta $
-spread set of density
$\delta $
-spread set of density
![]() $\mu _{A_n}(A)\ge e^{-n^{\alpha }}$
. Write
$\mu _{A_n}(A)\ge e^{-n^{\alpha }}$
. Write
![]() $g = \frac {1_A}{\mu _{A_n}(A)}$
. Then
$g = \frac {1_A}{\mu _{A_n}(A)}$
. Then
![]() $\|g^{=\chi }\|_2^2\le \chi (1)^{\alpha +\epsilon }$
for any
$\|g^{=\chi }\|_2^2\le \chi (1)^{\alpha +\epsilon }$
for any
![]() $\chi \in \widehat {A_n}$
.
$\chi \in \widehat {A_n}$
.
Proof. For a partition
![]() $\lambda $
, let us write
$\lambda $
, let us write
![]() $\lambda '$
for the conjugate partition obtained by replacing the roles of the rows and the columns in its Young diagram. Recall that the corresponding characters satisfy
$\lambda '$
for the conjugate partition obtained by replacing the roles of the rows and the columns in its Young diagram. Recall that the corresponding characters satisfy
![]() $\chi _{\lambda } = \chi _{\lambda '}\cdot \mathrm {sign}$
.
$\chi _{\lambda } = \chi _{\lambda '}\cdot \mathrm {sign}$
.
It is well known that all irreducible characters of
![]() $A_n$
are obtained from characters of
$A_n$
are obtained from characters of
![]() $S_n$
, in one of two possible ways:
$S_n$
, in one of two possible ways:
-
1. Characters that correspond to partitions
 $\lambda $
with
$\lambda $
with
 $\lambda \ne \lambda '$
: In this case, the characters
$\lambda \ne \lambda '$
: In this case, the characters
 $\chi _{\lambda }$
and
$\chi _{\lambda }$
and
 $\chi _{\lambda '}$
restrict to the same irreducible character of
$\chi _{\lambda '}$
restrict to the same irreducible character of
 $A_n$
.
$A_n$
. -
2. Characters that correspond to partitions
 $\lambda $
with
$\lambda $
with
 $\lambda = \lambda '$
. In this case, the restriction of
$\lambda = \lambda '$
. In this case, the restriction of
 $\chi _{\lambda }$
to
$\chi _{\lambda }$
to
 $A_n$
splits to the sum of two irreducible characters, which we denote by
$A_n$
splits to the sum of two irreducible characters, which we denote by
 $\chi _{\lambda _1}$
and
$\chi _{\lambda _1}$
and
 $\chi _{\lambda _2}$
, that have the same dimension.
$\chi _{\lambda _2}$
, that have the same dimension.
To handle the characters of the second type, we note that for any such
![]() $\lambda $
, the level of
$\lambda $
, the level of
![]() $\chi _{\lambda }$
is necessarily
$\chi _{\lambda }$
is necessarily
![]() $\ge n/2 - 1$
. Therefore, by Lemma 3.2, we have
$\ge n/2 - 1$
. Therefore, by Lemma 3.2, we have
 $$\begin{align*}\chi_{\lambda_1}(1) = \chi_{\lambda_2}(1)\ge \frac{1}{2}\left(\frac{200}{e}\right)^{n/200},\end{align*}$$
$$\begin{align*}\chi_{\lambda_1}(1) = \chi_{\lambda_2}(1)\ge \frac{1}{2}\left(\frac{200}{e}\right)^{n/200},\end{align*}$$
which implies that
provided that n is sufficiently large with respect to
![]() $\epsilon $
.
$\epsilon $
.
We now handle the characters of the first type. Let h be the extension of g to
![]() $S_n$
whose value on the odd permutations is
$S_n$
whose value on the odd permutations is
![]() $0$
. Write
$0$
. Write
![]() $h= \sum _{\lambda \vdash n} h^{=\chi _{\lambda }}.$
Let
$h= \sum _{\lambda \vdash n} h^{=\chi _{\lambda }}.$
Let
![]() $\lambda \ne \lambda '$
and let
$\lambda \ne \lambda '$
and let
![]() $\chi $
be the restriction of
$\chi $
be the restriction of
![]() $\chi _{\lambda }$
to
$\chi _{\lambda }$
to
![]() $A_n$
. Then
$A_n$
. Then
![]() $g^{=\chi } = \chi (1) g*\chi .$
We would like to write this convolution in terms of convolutions over
$g^{=\chi } = \chi (1) g*\chi .$
We would like to write this convolution in terms of convolutions over
![]() $S_n$
to which we will be able to apply Proposition 1.9.
$S_n$
to which we will be able to apply Proposition 1.9.
Let
![]() $\tilde {\chi } = \chi _{\lambda } + \chi _{\lambda '}.$
Then we have
$\tilde {\chi } = \chi _{\lambda } + \chi _{\lambda '}.$
Then we have
![]() $\tilde {\chi }(\sigma )=2\chi (\sigma )$
for all
$\tilde {\chi }(\sigma )=2\chi (\sigma )$
for all
![]() $\sigma \in A_n$
and
$\sigma \in A_n$
and
![]() $\tilde {\chi }(\sigma )=0$
for all
$\tilde {\chi }(\sigma )=0$
for all
![]() $\sigma \in S_n \setminus A_n$
. Therefore, the functions
$\sigma \in S_n \setminus A_n$
. Therefore, the functions
![]() $g*\chi $
and
$g*\chi $
and
![]() $h * \tilde {\chi }$
agree on
$h * \tilde {\chi }$
agree on
![]() $A_n$
(note that the first convolution takes place in
$A_n$
(note that the first convolution takes place in
![]() $A_n$
and the second takes place in
$A_n$
and the second takes place in
![]() $S_n$
). Hence,
$S_n$
). Hence,
The desired upper bound on
![]() $g^{=\chi }$
now follows from the triangle inequality, when applying Proposition 1.9 to h.
$g^{=\chi }$
now follows from the triangle inequality, when applying Proposition 1.9 to h.
4 Upper bounding spread independent sets in normal Cayley graphs
In this section, we prove Theorem 1.8, as well as several related results. We begin with a proposition that explains how to combine character bounds with hypercontractivity to upper bound the size of spread independent sets.
Recall that any class function
![]() $h:A_n \to \mathbb {C}$
can be uniquely represented as a linear combination of irreducible characters:
$h:A_n \to \mathbb {C}$
can be uniquely represented as a linear combination of irreducible characters:
![]() $h=\sum _{\chi \in \widehat {A_n}} h_{\chi } \chi $
. The coefficient of
$h=\sum _{\chi \in \widehat {A_n}} h_{\chi } \chi $
. The coefficient of
![]() $\chi $
in this expansion is denoted by
$\chi $
in this expansion is denoted by
![]() $\hat h(\chi )$
. Note that if
$\hat h(\chi )$
. Note that if
![]() $A=\sigma ^{S_n}$
for some
$A=\sigma ^{S_n}$
for some
![]() $\sigma \in A_n$
and
$\sigma \in A_n$
and
![]() $h=1_A$
, then for any
$h=1_A$
, then for any
![]() $\chi \in \widehat {A_n}$
, we have
$\chi \in \widehat {A_n}$
, we have
![]() $\hat h(\chi ) = \mu _{A_n}(A)\chi (\sigma )$
.
$\hat h(\chi ) = \mu _{A_n}(A)\chi (\sigma )$
.
Proposition 4.1. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $n_0 \in \mathbb {N}$
and
$n_0 \in \mathbb {N}$
and
![]() $\delta>0$
such that the following holds for all
$\delta>0$
such that the following holds for all
![]() $n>n_0$
and all
$n>n_0$
and all
![]() $0<\alpha <1-\epsilon $
. Suppose that
$0<\alpha <1-\epsilon $
. Suppose that
![]() $I,I'\subseteq G$
are
$I,I'\subseteq G$
are
![]() $\delta $
-spread subsets of
$\delta $
-spread subsets of
![]() $A_n$
, such that
$A_n$
, such that
![]() $\mu _{A_n}(I),\mu _{A_n}(I')>e^{-n^{1 - \alpha -\epsilon }}$
. Suppose additionally that
$\mu _{A_n}(I),\mu _{A_n}(I')>e^{-n^{1 - \alpha -\epsilon }}$
. Suppose additionally that
![]() $A\subseteq A_n$
is a normal set with
$A\subseteq A_n$
is a normal set with
 $$\begin{align*}\frac{\widehat{1_A}(\chi)}{\mu_{A_n}(A)} < \chi(1)^{\alpha}\end{align*}$$
$$\begin{align*}\frac{\widehat{1_A}(\chi)}{\mu_{A_n}(A)} < \chi(1)^{\alpha}\end{align*}$$
for every irreducible character
![]() $\chi $
of
$\chi $
of
![]() $A_n$
. Then the sets
$A_n$
. Then the sets
![]() $I,I'$
span at least one edge in the Cayley graph
$I,I'$
span at least one edge in the Cayley graph
![]() $\mathrm {Cay}(A_n,A)$
.
$\mathrm {Cay}(A_n,A)$
.
Proof. Write
![]() $h = \frac {1_A}{\mu _{A_n}(A)}$
. Let
$h = \frac {1_A}{\mu _{A_n}(A)}$
. Let
![]() $T_A$
be the operator associated with the Cayley graph generated by A (i.e.,
$T_A$
be the operator associated with the Cayley graph generated by A (i.e.,
![]() $T_A g = h * g$
). Let
$T_A g = h * g$
). Let
![]() $W_{\chi }: = \mathrm {span}\{g\chi \}_{g\in A_n}$
(where
$W_{\chi }: = \mathrm {span}\{g\chi \}_{g\in A_n}$
(where
![]() $g\chi $
is defined as
$g\chi $
is defined as
![]() $1_g * \chi $
) be the isotypic component of
$1_g * \chi $
) be the isotypic component of
![]() $\chi $
. Each
$\chi $
. Each
![]() $W_{\chi }$
consists of the union of all the irreducible representations
$W_{\chi }$
consists of the union of all the irreducible representations
![]() $\rho _{\chi }$
that correspond to
$\rho _{\chi }$
that correspond to
![]() $\chi $
in
$\chi $
in
![]() $L^2(A_n)$
. Hence, it follows from the Peter-Weyl theorem that each
$L^2(A_n)$
. Hence, it follows from the Peter-Weyl theorem that each
![]() $W_{\chi }$
is an irreducible
$W_{\chi }$
is an irreducible
![]() $A_n\times A_n$
representation appearing in
$A_n\times A_n$
representation appearing in
![]() $L^2(A_n)$
exactly once. The operator
$L^2(A_n)$
exactly once. The operator
![]() $T_A$
commutes with the action of
$T_A$
commutes with the action of
![]() $A_n\times A_n$
, and thus, it follows from Schur’s lemma that the restriction of
$A_n\times A_n$
, and thus, it follows from Schur’s lemma that the restriction of
![]() $T_A$
to
$T_A$
to
![]() $W_{\chi }$
is multiplication by a scalar. To compute the scalar it is sufficient to compute
$W_{\chi }$
is multiplication by a scalar. To compute the scalar it is sufficient to compute
![]() $T_A\chi $
. By Frobenius, we therefore obtain that the eigenvalue corresponding to
$T_A\chi $
. By Frobenius, we therefore obtain that the eigenvalue corresponding to
![]() $W_{\chi }$
is given by
$W_{\chi }$
is given by
![]() $\frac {\hat {h}(\chi )}{\chi (1)}$
. Write
$\frac {\hat {h}(\chi )}{\chi (1)}$
. Write
![]() $f= \frac {1_{I}}{\mu _{G}(I)}$
and
$f= \frac {1_{I}}{\mu _{G}(I)}$
and
![]() $g=\frac {1_{I'}}{\mu _{G}(I')}.$
We have
$g=\frac {1_{I'}}{\mu _{G}(I')}.$
We have
 $$ \begin{align} \langle T_A f , g \rangle = \sum_{\chi} \frac{\widehat{h}(\chi)}{\chi(1)}\langle f^{=\chi} , g^{=\chi} \rangle. \end{align} $$
$$ \begin{align} \langle T_A f , g \rangle = \sum_{\chi} \frac{\widehat{h}(\chi)}{\chi(1)}\langle f^{=\chi} , g^{=\chi} \rangle. \end{align} $$
By Proposition 3.3, applied with
![]() $\epsilon /2$
in place of
$\epsilon /2$
in place of
![]() $\epsilon $
, and Theorem 2.14, we therefore have
$\epsilon $
, and Theorem 2.14, we therefore have
 $$\begin{align*}\left| \langle T_A f , g\rangle - 1\right| \le \sum_{\chi \in \widehat{A_n}\setminus \{\mathrm{triv}\}} \chi(1)^{\alpha - 1}\|f^{=\chi}\|_2 \|g^{=\chi}\|_2\le \sum_{\chi \in \widehat{A_n} \setminus \{triv\}} \chi(1)^{-\epsilon/2} = o(1).\end{align*}$$
$$\begin{align*}\left| \langle T_A f , g\rangle - 1\right| \le \sum_{\chi \in \widehat{A_n}\setminus \{\mathrm{triv}\}} \chi(1)^{\alpha - 1}\|f^{=\chi}\|_2 \|g^{=\chi}\|_2\le \sum_{\chi \in \widehat{A_n} \setminus \{triv\}} \chi(1)^{-\epsilon/2} = o(1).\end{align*}$$
Hence, we have
![]() $\langle T_A f , g \rangle \ne 0$
, provided that n is sufficiently large, which implies that there exists an edge between I and
$\langle T_A f , g \rangle \ne 0$
, provided that n is sufficiently large, which implies that there exists an edge between I and
![]() $I'$
.
$I'$
.
The following theorem follows by combining Proposition 4.1 with the results of Larsen–Shalev [Reference Larsen and Shalev23] and Larsen–Tiep [Reference Larsen and Tiep25] presented in Section 2.
Theorem 4.2. For any
![]() $\epsilon>0$
, there exist
$\epsilon>0$
, there exist
![]() $n_0 \in \mathbb {N}$
and
$n_0 \in \mathbb {N}$
and
![]() $\delta>0$
, such that the following holds for all
$\delta>0$
, such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $\sigma \in A_n$
and write
$\sigma \in A_n$
and write
![]() $E(\sigma ) = \alpha .$
Then every
$E(\sigma ) = \alpha .$
Then every
![]() $\delta $
-spread independent set in the Cayley graph
$\delta $
-spread independent set in the Cayley graph
![]() $\mathrm {Cay}(A_n,\sigma ^{S_n})$
has density
$\mathrm {Cay}(A_n,\sigma ^{S_n})$
has density
![]() $\le e^{-n^{1-\alpha -\epsilon }}.$
$\le e^{-n^{1-\alpha -\epsilon }}.$
Proof. Let
![]() $A = \sigma ^{S_n}$
. As
$A = \sigma ^{S_n}$
. As
![]() $E(\sigma )=\alpha $
, we may apply Theorem 2.4 to deduce that for any character
$E(\sigma )=\alpha $
, we may apply Theorem 2.4 to deduce that for any character
![]() $\chi $
of
$\chi $
of
![]() $A_n$
,
$A_n$
,
 $$\begin{align*}\frac{\widehat{1_A}(\chi)}{\mu_{A_n}(A)}=\chi(\sigma) \le \chi(1)^{\alpha+\epsilon/2}.\end{align*}$$
$$\begin{align*}\frac{\widehat{1_A}(\chi)}{\mu_{A_n}(A)}=\chi(\sigma) \le \chi(1)^{\alpha+\epsilon/2}.\end{align*}$$
The assertion now follows from Proposition 4.1, when substituting
![]() $\alpha +\epsilon /2$
in place of
$\alpha +\epsilon /2$
in place of
![]() $\alpha $
and
$\alpha $
and
![]() $\epsilon /2$
in place of
$\epsilon /2$
in place of
![]() $\epsilon $
.
$\epsilon $
.
Now we are ready to present the proofs of Theorems 1.7 and 1.8.
Proof of Theorem 1.7.
Let
![]() $\epsilon>0$
, let
$\epsilon>0$
, let
![]() $\delta ,n_0$
(depending on
$\delta ,n_0$
(depending on
![]() $\epsilon $
) be determined below, and let I be an independent set in
$\epsilon $
) be determined below, and let I be an independent set in
![]() $Cay(A_n,\sigma ^{S_n})$
, where
$Cay(A_n,\sigma ^{S_n})$
, where
![]() $n \geq n_0+t$
and
$n \geq n_0+t$
and
![]() $\sigma \in A_n$
is a permutation with t fixed points. Assume on the contrary that
$\sigma \in A_n$
is a permutation with t fixed points. Assume on the contrary that
![]() $\mu _{A_n}(I)>\max (e^{-(n-t)^{1/3-\epsilon }},(n-t)^{-\delta t})$
. We obtain a contradiction in a three-step argument.
$\mu _{A_n}(I)>\max (e^{-(n-t)^{1/3-\epsilon }},(n-t)^{-\delta t})$
. We obtain a contradiction in a three-step argument.
Step 1: Reducing to the case
![]() $t \leq n^{1/3}$
. We use the following observation. Let
$t \leq n^{1/3}$
. We use the following observation. Let
![]() $1 \leq \ell \le t$
and let
$1 \leq \ell \le t$
and let
![]() $\sigma '$
be obtained from
$\sigma '$
be obtained from
![]() $\sigma $
by deleting
$\sigma $
by deleting
![]() $\ell $
of its fixed points. For each
$\ell $
of its fixed points. For each
![]() $\ell $
-umvirate
$\ell $
-umvirate
![]() $\tau U$
, with U the subgroup of all permutations that fix a given set of size
$\tau U$
, with U the subgroup of all permutations that fix a given set of size
![]() $\ell $
, the set
$\ell $
, the set
![]() $I' = \tau ^{-1}I\cap U$
is independent in the Cayley graph
$I' = \tau ^{-1}I\cap U$
is independent in the Cayley graph
![]() $\mathrm {Cay}(U,(\sigma ')^{U})$
which is isomorphic to
$\mathrm {Cay}(U,(\sigma ')^{U})$
which is isomorphic to
![]() $\mathrm {Cay}(A_{n-\ell },(\sigma ')^{S_{n-\ell }})$
.
$\mathrm {Cay}(A_{n-\ell },(\sigma ')^{S_{n-\ell }})$
.
If
![]() $t>n^{1/3}$
, we may reduce the number of fixed points by applying this process with
$t>n^{1/3}$
, we may reduce the number of fixed points by applying this process with
![]() $\ell = \lceil t - (n-t)^{1/3} \rceil $
, choosing an
$\ell = \lceil t - (n-t)^{1/3} \rceil $
, choosing an
![]() $\ell $
-umvirate
$\ell $
-umvirate
![]() $\tau U$
such that
$\tau U$
such that
![]() $\frac {\mu (I \cap \tau U)}{\mu (U)} \geq \mu _{A_n}(I)$
. The resulting set
$\frac {\mu (I \cap \tau U)}{\mu (U)} \geq \mu _{A_n}(I)$
. The resulting set
![]() $I'$
is independent in the Cayley graph
$I'$
is independent in the Cayley graph
![]() $\mathrm {Cay}(A_{n'},(\sigma ')^{A_{n'}})$
with
$\mathrm {Cay}(A_{n'},(\sigma ')^{A_{n'}})$
with
![]() $n'=n-\ell $
, where the number
$n'=n-\ell $
, where the number
![]() $t'=\lfloor (n-t)^{1/3} \rfloor $
of fixed points of
$t'=\lfloor (n-t)^{1/3} \rfloor $
of fixed points of
![]() $\sigma '$
satisfies
$\sigma '$
satisfies
![]() $(n')^{1/3-\epsilon /2} \leq t'\leq (n')^{1/3}$
, provided that
$(n')^{1/3-\epsilon /2} \leq t'\leq (n')^{1/3}$
, provided that
![]() $n_0$
is sufficiently large as a function of
$n_0$
is sufficiently large as a function of
![]() $\epsilon $
.
$\epsilon $
.
To see that
![]() $\mu _{A_{n'}}(I')>\max (e^{-(n'-t')^{1/3-\epsilon }},(n'-t')^{-\delta t'})$
, note that for any
$\mu _{A_{n'}}(I')>\max (e^{-(n'-t')^{1/3-\epsilon }},(n'-t')^{-\delta t'})$
, note that for any
![]() $\epsilon ,\delta>0$
, for any sufficiently large
$\epsilon ,\delta>0$
, for any sufficiently large
![]() $n_0$
(depending on
$n_0$
(depending on
![]() $\epsilon ,\delta $
), and for any
$\epsilon ,\delta $
), and for any
![]() $n,t$
such that
$n,t$
such that
![]() $t\gg n^{1/3-\epsilon }$
and
$t\gg n^{1/3-\epsilon }$
and
![]() $n-t\geq n_0$
, we have
$n-t\geq n_0$
, we have
![]() $e^{-(n-t)^{1/3-\epsilon }}\gg (n-t)^{-\delta t}$
. Hence,
$e^{-(n-t)^{1/3-\epsilon }}\gg (n-t)^{-\delta t}$
. Hence,
 $$ \begin{align*} \mu_{A_{n'}}(I') &\geq \mu_{A_n}(I)>\max(e^{-(n-t)^{1/3-\epsilon}},(n-t)^{-\delta t}) = e^{-(n-t)^{1/3-\epsilon}} = e^{-(n'-t')^{1/3-\epsilon}} \\ &=\max(e^{-(n'-t')^{1/3-\epsilon}},(n'-t')^{-\delta t'}), \end{align*} $$
$$ \begin{align*} \mu_{A_{n'}}(I') &\geq \mu_{A_n}(I)>\max(e^{-(n-t)^{1/3-\epsilon}},(n-t)^{-\delta t}) = e^{-(n-t)^{1/3-\epsilon}} = e^{-(n'-t')^{1/3-\epsilon}} \\ &=\max(e^{-(n'-t')^{1/3-\epsilon}},(n'-t')^{-\delta t'}), \end{align*} $$
where the last equality holds since
![]() $t' \geq (n')^{1/3-\epsilon /2}$
. Therefore,
$t' \geq (n')^{1/3-\epsilon /2}$
. Therefore,
![]() $I'$
satisfies the ‘contrary assumption’ for
$I'$
satisfies the ‘contrary assumption’ for
![]() $(n',t')$
in place of
$(n',t')$
in place of
![]() $(n,t)$
. This shows that we may assume w.l.o.g. that
$(n,t)$
. This shows that we may assume w.l.o.g. that
![]() $t\le n^{1/3}$
.
$t\le n^{1/3}$
.
Step 2: Reducing to the case where I is
![]() $\delta $
-spread. Similarly to the first step, we may also assume that I is
$\delta $
-spread. Similarly to the first step, we may also assume that I is
![]() $\delta $
-spread, as otherwise we may iteratively find
$\delta $
-spread, as otherwise we may iteratively find
![]() $\ell $
-umvirates in which the density of A is
$\ell $
-umvirates in which the density of A is
![]() $\ge \mu (A)n^{\delta \ell }$
until we are stuck. The set
$\ge \mu (A)n^{\delta \ell }$
until we are stuck. The set
![]() $I"$
we obtain at the end of this process is an independent
$I"$
we obtain at the end of this process is an independent
![]() $\delta $
-spread set in the Cayley graph
$\delta $
-spread set in the Cayley graph
![]() $\mathrm {Cay}(A_{n"},(\sigma ")^{A_{n"}})$
, where
$\mathrm {Cay}(A_{n"},(\sigma ")^{A_{n"}})$
, where
![]() $\sigma "$
has
$\sigma "$
has
![]() $t"$
fixed points and
$t"$
fixed points and
![]() $n"=n-(t-t")$
. Its measure satisfies
$n"=n-(t-t")$
. Its measure satisfies
![]() $\mu _{A_{n"}}(I") \geq \max (e^{-(n"-t")^{1/3-\epsilon }},(n"-t")^{-\delta t"})$
, as in the transition from
$\mu _{A_{n"}}(I") \geq \max (e^{-(n"-t")^{1/3-\epsilon }},(n"-t")^{-\delta t"})$
, as in the transition from
![]() $(n,t)$
to
$(n,t)$
to
![]() $(n",t")$
, the left term remains unchanged and the increase of the right term is less than the density increase by a factor of
$(n",t")$
, the left term remains unchanged and the increase of the right term is less than the density increase by a factor of
![]() $n^{\delta \ell }$
which we obtain in each
$n^{\delta \ell }$
which we obtain in each
![]() $\ell $
-restriction. This shows that we may assume w.l.o.g. that I is
$\ell $
-restriction. This shows that we may assume w.l.o.g. that I is
![]() $\delta $
-spread.
$\delta $
-spread.
Step 3: Applying Theorem 1.8. Assuming that
![]() $t \leq n^{1/3}$
and that I is
$t \leq n^{1/3}$
and that I is
![]() $\delta $
-spread, we can apply Theorem 1.8, with the same value of
$\delta $
-spread, we can apply Theorem 1.8, with the same value of
![]() $\epsilon $
, to deduce that
$\epsilon $
, to deduce that
which contradicts the assumption
![]() $\mu _{A_n}(I)>\max (e^{-(n-t)^{1/3-\epsilon }},(n-t)^{-\delta t})$
. This completes the proof (with
$\mu _{A_n}(I)>\max (e^{-(n-t)^{1/3-\epsilon }},(n-t)^{-\delta t})$
. This completes the proof (with
![]() $\delta $
being the same as in Theorem 1.8 and
$\delta $
being the same as in Theorem 1.8 and
![]() $n_0$
being sufficiently large).
$n_0$
being sufficiently large).
5 The spreadness of conjugacy classes and their restrictions
In this section, we prove that ‘large’ conjugacy classes of permutations with not-too-many short cycles are spread and that the same holds for their restrictions inside t-umvirates (under certain additional conditions). In order to state our goal more precisely, we introduce some more terminology.
A d-restriction of a function is its restriction to a d-umvirate
![]() $U_{I\rightarrow J}$
with
$U_{I\rightarrow J}$
with
![]() $|I|=d$
. A k-chain is a restriction of the form
$|I|=d$
. A k-chain is a restriction of the form
![]() $i_1\rightarrow i_2\rightarrow \ldots \rightarrow i_{k+1}$
(i.e.,
$i_1\rightarrow i_2\rightarrow \ldots \rightarrow i_{k+1}$
(i.e.,
![]() $i_1 \to i_2, i_2 \to i_3, \ldots , i_k \to i_{k+1})$
, where
$i_1 \to i_2, i_2 \to i_3, \ldots , i_k \to i_{k+1})$
, where
![]() $i_1,\ldots i_{k+1}$
are all different. In other words, a k-chain is the restriction to the k-umvirate
$i_1,\ldots i_{k+1}$
are all different. In other words, a k-chain is the restriction to the k-umvirate
![]() $U_{I\rightarrow J}$
, where
$U_{I\rightarrow J}$
, where
![]() $I=(i_1,\ldots ,i_k)$
, and
$I=(i_1,\ldots ,i_k)$
, and
![]() $J=(i_2,\ldots ,i_{k+1})$
. We say that a
$J=(i_2,\ldots ,i_{k+1})$
. We say that a
![]() $k_1$
-chain
$k_1$
-chain
![]() $(i_1\rightarrow i_2\rightarrow \ldots \rightarrow i_{k_1+1})$
and
$(i_1\rightarrow i_2\rightarrow \ldots \rightarrow i_{k_1+1})$
and
![]() $k_2$
-chain
$k_2$
-chain
![]() $(j_1\rightarrow j_2\rightarrow \ldots \rightarrow j_{k_2+1})$
are disjoint if all the coordinates
$(j_1\rightarrow j_2\rightarrow \ldots \rightarrow j_{k_2+1})$
are disjoint if all the coordinates
![]() $i_1,\ldots ,i_{k_1+1},j_1,\ldots ,j_{k_2+1} $
are different. We say that the length of a
$i_1,\ldots ,i_{k_1+1},j_1,\ldots ,j_{k_2+1} $
are different. We say that the length of a
![]() $k_1$
-chain is
$k_1$
-chain is
![]() $k_1$
. A k-restriction is a k-cycle if it takes the form
$k_1$
. A k-restriction is a k-cycle if it takes the form
![]() $i_1\rightarrow i_2\rightarrow \ldots \rightarrow i_{k}\rightarrow i_1$
. Every d-restriction can be decomposed to disjoint cycles and k-chains that we call the parts of the restriction.
$i_1\rightarrow i_2\rightarrow \ldots \rightarrow i_{k}\rightarrow i_1$
. Every d-restriction can be decomposed to disjoint cycles and k-chains that we call the parts of the restriction.
We prove the following lemma, as well as a variant of it (Lemma 5.3 below) that will be used in the sequel. For the sake of convenience, we use the term ‘globalness’ throughout this section. Recall that a set is
![]() $\delta $
-spread if and only if it is
$\delta $
-spread if and only if it is
![]() $n^{\delta }$
-global.
$n^{\delta }$
-global.
Lemma 5.1. There exists
![]() $n_0>0$
such that the following holds for all
$n_0>0$
such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $r\ge 25$
, and let
$r\ge 25$
, and let
![]() $A\subseteq S_n$
be a conjugacy class of density at least
$A\subseteq S_n$
be a conjugacy class of density at least
![]() $e^{-n}$
, such that all the permutations in A have at most
$e^{-n}$
, such that all the permutations in A have at most
![]() $(r/2)^{\ell }\ \ell $
-cycles for each
$(r/2)^{\ell }\ \ell $
-cycles for each
![]() $\ell $
. Then A is r-global.
$\ell $
. Then A is r-global.
In order to prove the lemma, we first prove the following claim which calculates the measure of restrictions without cycles.
Claim 5.2. Let A be a normal set. Let
![]() $d>0$
and let
$d>0$
and let
![]() $A_{I\to J}$
be a d-restriction that consists of t chains. Denote the chain lengths of
$A_{I\to J}$
be a d-restriction that consists of t chains. Denote the chain lengths of
![]() $A_{I\to J}$
by
$A_{I\to J}$
by
![]() $i_1 - 1,\ldots , i_t - 1$
. Let P be the probability that for a random permutation
$i_1 - 1,\ldots , i_t - 1$
. Let P be the probability that for a random permutation
![]() $\tau \sim A$
, and for all
$\tau \sim A$
, and for all
![]() $1 \leq \ell \le t$
, the length of the cycle containing
$1 \leq \ell \le t$
, the length of the cycle containing
![]() $\ell $
in
$\ell $
in
![]() $\tau $
is at least
$\tau $
is at least
![]() $i_{\ell }.$
Then
$i_{\ell }.$
Then
 $$\begin{align*}\mu(A_{I\to J}) = \mu(A) \cdot P \cdot \left[\left(1-\frac{t}{n}\right)\left(1-\frac{t}{n-1}\right)\cdots\left(1 - \frac{t}{n+1-|I|}\right)\right]^{-1}. \end{align*}$$
$$\begin{align*}\mu(A_{I\to J}) = \mu(A) \cdot P \cdot \left[\left(1-\frac{t}{n}\right)\left(1-\frac{t}{n-1}\right)\cdots\left(1 - \frac{t}{n+1-|I|}\right)\right]^{-1}. \end{align*}$$
Proof. Decompose the d-restriction
![]() $A_{I\rightarrow J}$
into its chain parts
$A_{I\rightarrow J}$
into its chain parts
Consider the family of d-umvirates
![]() $U_{\sigma (I)\to \sigma (J)} = \sigma U_{I\to J}\sigma ^{-1}$
, for all permutations
$U_{\sigma (I)\to \sigma (J)} = \sigma U_{I\to J}\sigma ^{-1}$
, for all permutations
![]() $\sigma \in S_n$
that fix each of
$\sigma \in S_n$
that fix each of
![]() $a_{11},\ldots , a_{t1}$
. It is clear that any two non-equal d-umvirates of this form are pairwise disjoint (as sets of permutations). Moreover, since A is normal, the measure of A inside each such d-umvirate is the same.
$a_{11},\ldots , a_{t1}$
. It is clear that any two non-equal d-umvirates of this form are pairwise disjoint (as sets of permutations). Moreover, since A is normal, the measure of A inside each such d-umvirate is the same.
Without loss of generality, we may assume
![]() $a_{11} = 1,\ldots , a_{t1} = t.$
Observe that
$a_{11} = 1,\ldots , a_{t1} = t.$
Observe that
![]() $A\cap (\bigcup _{\sigma \in U_{(1,\ldots , t)\to (1,\ldots , t)}}U_{\sigma (I)\to \sigma (J)})$
consists of all the permutations in A for which for all
$A\cap (\bigcup _{\sigma \in U_{(1,\ldots , t)\to (1,\ldots , t)}}U_{\sigma (I)\to \sigma (J)})$
consists of all the permutations in A for which for all
![]() $1 \leq \ell \leq t$
, the length of the cycle that contains
$1 \leq \ell \leq t$
, the length of the cycle that contains
![]() $\ell $
is
$\ell $
is
![]() $\ge i_{\ell }$
. Hence, we have
$\ge i_{\ell }$
. Hence, we have
Therefore, in order to prove the claim, all that remains is computing the orbit of
![]() $U_{I\to J}$
with respect to the action of the group
$U_{I\to J}$
with respect to the action of the group
![]() $U_{(1,\ldots , t)\to (1,\ldots ,t)}$
on
$U_{(1,\ldots , t)\to (1,\ldots ,t)}$
on
![]() $S_n$
by conjugation. By the orbit stabilizer theorem, its size is
$S_n$
by conjugation. By the orbit stabilizer theorem, its size is
As
![]() $\mu (U_{I\to J}) = [n(n-1)\cdot \ldots \cdot (n-i_1-i_2-\ldots -i_t+t+1)]^{-1}$
, the claim follows by rearranging.
$\mu (U_{I\to J}) = [n(n-1)\cdot \ldots \cdot (n-i_1-i_2-\ldots -i_t+t+1)]^{-1}$
, the claim follows by rearranging.
Proof of Lemma 5.1.
Given a restriction
![]() $A_{I\rightarrow J}$
of A, we view it as a composition of two restrictions, denoted by
$A_{I\rightarrow J}$
of A, we view it as a composition of two restrictions, denoted by
![]() $I_1\rightarrow J_1$
and
$I_1\rightarrow J_1$
and
![]() $I_2\rightarrow J_2$
, where the restriction
$I_2\rightarrow J_2$
, where the restriction
![]() $I_1\rightarrow J_1$
consists of all the cycle parts of
$I_1\rightarrow J_1$
consists of all the cycle parts of
![]() $I\to J$
, and the restriction
$I\to J$
, and the restriction
![]() $I_2\rightarrow J_2$
consists of the chain parts. Let us consider each restriction separately.
$I_2\rightarrow J_2$
consists of the chain parts. Let us consider each restriction separately.
Density increase in a restriction consisting of cycles. By the orbit stabilizer theorem, if
![]() $\sigma $
has
$\sigma $
has
![]() $f_{\sigma }(i)$
cycles of length i for each i, then the density of its conjugacy class
$f_{\sigma }(i)$
cycles of length i for each i, then the density of its conjugacy class
![]() $\sigma ^{S_n}$
in
$\sigma ^{S_n}$
in
![]() $S_n$
is
$S_n$
is
![]() $1/\prod (i^{f_{\sigma }(i)}\cdot f_{\sigma }(i)!)$
. Therefore, when removing a cycle of size
$1/\prod (i^{f_{\sigma }(i)}\cdot f_{\sigma }(i)!)$
. Therefore, when removing a cycle of size
![]() $\ell $
from
$\ell $
from
![]() $\sigma $
, the measure of the corresponding conjugacy class increases by a factor of
$\sigma $
, the measure of the corresponding conjugacy class increases by a factor of
![]() $\ell \cdot f_{\sigma }(\ell )$
. By assumption, we have
$\ell \cdot f_{\sigma }(\ell )$
. By assumption, we have
![]() $f_{\sigma }(\ell ) \leq (r/2)^{\ell }$
, and hence, when deleting an
$f_{\sigma }(\ell ) \leq (r/2)^{\ell }$
, and hence, when deleting an
![]() $\ell $
-cycle from
$\ell $
-cycle from
![]() $\sigma $
, the density of the corresponding conjugacy class increases by a factor of
$\sigma $
, the density of the corresponding conjugacy class increases by a factor of
![]() $ \le (\ell ^{1/\ell } r/2)^{\ell }.$
Set
$ \le (\ell ^{1/\ell } r/2)^{\ell }.$
Set
![]() $A' = A_{I_1\rightarrow J_1}$
, and write
$A' = A_{I_1\rightarrow J_1}$
, and write
![]() $k= |I_1|, n' = n-k$
. We obtain that
$k= |I_1|, n' = n-k$
. We obtain that
![]() $\mu (A^{\prime }_{I_1\rightarrow J_1})\le r^k\mu (A)$
, by sequentially removing cycles from
$\mu (A^{\prime }_{I_1\rightarrow J_1})\le r^k\mu (A)$
, by sequentially removing cycles from
![]() $\sigma $
and taking into account the measure increment at each step.
$\sigma $
and taking into account the measure increment at each step.
Density increase in a restriction consisting of chains. Denote the lengths of the chains in the restriction by
![]() $i_1-1,i_2-1,\ldots ,i_t-1$
. Note that we may assume that
$i_1-1,i_2-1,\ldots ,i_t-1$
. Note that we may assume that
![]() $(i_1-1) + \ldots + (i_t-1) < n/3$
, for otherwise, the lemma holds trivially. Hence, we have
$(i_1-1) + \ldots + (i_t-1) < n/3$
, for otherwise, the lemma holds trivially. Hence, we have
![]() $1 - \frac {t}{n+1-|I|}>1/2$
, and consequently,
$1 - \frac {t}{n+1-|I|}>1/2$
, and consequently,
 $$\begin{align*}\left[\left(1-\frac{t}{n}\right)\left(1-\frac{t}{n-1}\right)\cdots\left(1 - \frac{t}{n+1-|I|}\right)\right]^{-1} \leq 2^n. \end{align*}$$
$$\begin{align*}\left[\left(1-\frac{t}{n}\right)\left(1-\frac{t}{n-1}\right)\cdots\left(1 - \frac{t}{n+1-|I|}\right)\right]^{-1} \leq 2^n. \end{align*}$$
Therefore, the upper bound
![]() $\mu (A_{I\to J}) = \mu (A^{\prime }_{I_2\to J_2}) \le 2^{|I_2|}\mu (A') \leq r^{|I|}\mu (A)$
follows immediately from Claim 5.2, applied with
$\mu (A_{I\to J}) = \mu (A^{\prime }_{I_2\to J_2}) \le 2^{|I_2|}\mu (A') \leq r^{|I|}\mu (A)$
follows immediately from Claim 5.2, applied with
![]() $A'$
in place of A.
$A'$
in place of A.
Lemma 5.3. There exist
![]() $n_0 \in \mathbb {N}$
and
$n_0 \in \mathbb {N}$
and
![]() $C>0$
, such that the following holds for all
$C>0$
, such that the following holds for all
![]() $n>n_0$
and all
$n>n_0$
and all
![]() $r\ge 20$
. Let
$r\ge 20$
. Let
![]() $\sigma \in S_n$
be a permutation that has at most
$\sigma \in S_n$
be a permutation that has at most
![]() $r/20$
fixed points and 2-cycles, and at most
$r/20$
fixed points and 2-cycles, and at most
![]() $(r/20)^{\ell /3}\ \ell $
-cycles for each
$(r/20)^{\ell /3}\ \ell $
-cycles for each
![]() $\ell \ge 3$
. Suppose in addition that
$\ell \ge 3$
. Suppose in addition that
![]() $A = \sigma ^{S_n}$
has density
$A = \sigma ^{S_n}$
has density
![]() $\ge e^{-\sqrt {n/C}}.$
Let
$\ge e^{-\sqrt {n/C}}.$
Let
![]() $d \le \frac {\sqrt {n}}{rC}$
and suppose that
$d \le \frac {\sqrt {n}}{rC}$
and suppose that
![]() $A_{I\to J}$
is a d-restriction of A whose parts consist only of 1-chains and 2-chains. Then
$A_{I\to J}$
is a d-restriction of A whose parts consist only of 1-chains and 2-chains. Then
![]() $A_{I\to J}$
is r-global.
$A_{I\to J}$
is r-global.
Proof. Let A be a conjugacy class that satisfies the assumptions of the lemma, and let
![]() $A_{I\to J}$
be a d-restriction of A. Let
$A_{I\to J}$
be a d-restriction of A. Let
![]() $A_{I'\to J'}$
be an
$A_{I'\to J'}$
be an
![]() $\ell $
-restriction of
$\ell $
-restriction of
![]() $A_{I\to J}.$
Our goal is to show that
$A_{I\to J}.$
Our goal is to show that
![]() $\mu (A_{I'\to J'}) \le r^{\ell }\mu (A_{I\to J})$
.
$\mu (A_{I'\to J'}) \le r^{\ell }\mu (A_{I\to J})$
.
Similarly to the proof of Lemma 5.1, we view the restriction
![]() $I' \to J'$
as a composition of two restrictions, denoted by
$I' \to J'$
as a composition of two restrictions, denoted by
![]() $I_1\rightarrow J_1$
and
$I_1\rightarrow J_1$
and
![]() $I_2\rightarrow J_2$
, where the restriction
$I_2\rightarrow J_2$
, where the restriction
![]() $I_1\rightarrow J_1$
consists of all the cycle parts, and the restriction
$I_1\rightarrow J_1$
consists of all the cycle parts, and the restriction
![]() $I_2\rightarrow J_2$
consists of the chain parts. We will show that
$I_2\rightarrow J_2$
consists of the chain parts. We will show that
 $$ \begin{align} \frac{\mu(A_{I' \to J'})}{\mu(A_{I \to J})}= \frac{\mu(A_{I_1 \to J_1})}{\mu(A_{(I \cap I_1) \to (J \cap J_1)})}\cdot \frac{\mu(A_{I' \to J'})/\mu(A_{I_1 \to J_1})}{\mu(A_{I \to J})/\mu(A_{(I \cap I_1) \to (J \cap J_1)})} \leq r^{\ell}, \end{align} $$
$$ \begin{align} \frac{\mu(A_{I' \to J'})}{\mu(A_{I \to J})}= \frac{\mu(A_{I_1 \to J_1})}{\mu(A_{(I \cap I_1) \to (J \cap J_1)})}\cdot \frac{\mu(A_{I' \to J'})/\mu(A_{I_1 \to J_1})}{\mu(A_{I \to J})/\mu(A_{(I \cap I_1) \to (J \cap J_1)})} \leq r^{\ell}, \end{align} $$
by considering each restriction separately.
Density increase in the restriction
![]() $I_1\rightarrow J_1$
consisting of cycles. Here, we have to bound the density increase
$I_1\rightarrow J_1$
consisting of cycles. Here, we have to bound the density increase
![]() $\frac {\mu (A_{I_1 \to J_1})}{\mu (A_{(I \cap I_1) \to (J \cap J_1)})}$
. It will be more convenient for us to bound the density increase
$\frac {\mu (A_{I_1 \to J_1})}{\mu (A_{(I \cap I_1) \to (J \cap J_1)})}$
. It will be more convenient for us to bound the density increase
![]() $\mu (A_{I_1 \to J_1})/\mu (A)$
instead. To see that this is sufficient, note that by Claim 5.2, we have
$\mu (A_{I_1 \to J_1})/\mu (A)$
instead. To see that this is sufficient, note that by Claim 5.2, we have
Indeed, denoting the lengths of the chains in the restriction
![]() $(A_{(I \cap I_1) \to (J \cap J_1)})$
by
$(A_{(I \cap I_1) \to (J \cap J_1)})$
by
![]() $i_1-1,i_2-1,\ldots ,i_s-1$
, the claim implies that
$i_1-1,i_2-1,\ldots ,i_s-1$
, the claim implies that
![]() $\mu (A_{(I \cap I_1) \to (J \cap J_1)})\ge \mu (A) \cdot P$
, where P is the probability that for a randomly chosen
$\mu (A_{(I \cap I_1) \to (J \cap J_1)})\ge \mu (A) \cdot P$
, where P is the probability that for a randomly chosen
![]() $\tau \sim A$
, for all
$\tau \sim A$
, for all
![]() $j=1,\ldots ,s$
, the length of the cycle containing j in
$j=1,\ldots ,s$
, the length of the cycle containing j in
![]() $\tau $
is at least
$\tau $
is at least
![]() $i_j$
. By assumption,
$i_j$
. By assumption,
![]() $i_j \leq 2$
for all j, the permutations in A have at most
$i_j \leq 2$
for all j, the permutations in A have at most
![]() $3r/20$
elements in cycles of length
$3r/20$
elements in cycles of length
![]() $\leq 2$
, and we have
$\leq 2$
, and we have
![]() $s \leq d \leq \frac {n}{rC}$
. Hence,
$s \leq d \leq \frac {n}{rC}$
. Hence,
 $$\begin{align*}P \geq \left(1-\frac{3r}{20n}\right)^s \geq \left(1-\frac{3r}{20n}\right)^{\sqrt{n}/rC}>1/2, \end{align*}$$
$$\begin{align*}P \geq \left(1-\frac{3r}{20n}\right)^s \geq \left(1-\frac{3r}{20n}\right)^{\sqrt{n}/rC}>1/2, \end{align*}$$
provided that C is sufficiently large.
In order to bound
![]() $\mu (A_{I_1 \to J_1})/\mu (A)$
, we observe that as the ‘old’ restriction
$\mu (A_{I_1 \to J_1})/\mu (A)$
, we observe that as the ‘old’ restriction
![]() $I \to J$
consists only of 1-chains and 2-chains, each cycle of length
$I \to J$
consists only of 1-chains and 2-chains, each cycle of length
![]() $l \geq 3$
in
$l \geq 3$
in
![]() $I_1 \to J_1$
contains at least
$I_1 \to J_1$
contains at least
![]() $l/3$
elements from the ‘new’ restriction
$l/3$
elements from the ‘new’ restriction
![]() $I_1 \setminus I \to J_1 \setminus J$
. Similarly, each cycle of length
$I_1 \setminus I \to J_1 \setminus J$
. Similarly, each cycle of length
![]() $1$
or
$1$
or
![]() $2$
in
$2$
in
![]() $I_1 \to J_1$
contains at least one element from the restriction
$I_1 \to J_1$
contains at least one element from the restriction
![]() $I_1 \setminus I \to J_1 \setminus J$
. As was shown in the proof of Lemma 5.1, the density increase when removing a single cycle of length l from the conjugacy class of
$I_1 \setminus I \to J_1 \setminus J$
. As was shown in the proof of Lemma 5.1, the density increase when removing a single cycle of length l from the conjugacy class of
![]() $\sigma \in S_n$
is at most
$\sigma \in S_n$
is at most
![]() $l \cdot f_{\sigma }(l)$
. By assumption, we have
$l \cdot f_{\sigma }(l)$
. By assumption, we have
![]() $f_{\sigma }(l) \leq (r/20)^{l/3}$
for all
$f_{\sigma }(l) \leq (r/20)^{l/3}$
for all
![]() $l \geq 3$
, and also
$l \geq 3$
, and also
![]() $f_{\sigma }(l) \leq (r/20)^{l/2}$
for
$f_{\sigma }(l) \leq (r/20)^{l/2}$
for
![]() $l=2$
and
$l=2$
and
![]() $f_{\sigma }(l) \leq (r/20)^{l}$
for
$f_{\sigma }(l) \leq (r/20)^{l}$
for
![]() $l=1$
. It follows that the density increase when removing a cycle that contains
$l=1$
. It follows that the density increase when removing a cycle that contains
![]() $l'$
‘new’ coordinates is at most
$l'$
‘new’ coordinates is at most
![]() $3l' \cdot (r/20)^{l'} \leq (r/8)^{l'}$
. Since the number of ‘new’ coordinates is
$3l' \cdot (r/20)^{l'} \leq (r/8)^{l'}$
. Since the number of ‘new’ coordinates is
![]() $|I_1 \setminus I| \leq \ell $
, by sequentially removing cycles from
$|I_1 \setminus I| \leq \ell $
, by sequentially removing cycles from
![]() $\sigma $
and taking into account the measure increment at each step, we obtain
$\sigma $
and taking into account the measure increment at each step, we obtain
 $$ \begin{align} \frac{\mu(A_{I_1 \to J_1})}{\mu(A_{(I \cap I_1) \to (J \cap J_1)})} \leq 2 \cdot \frac{\mu(A_{I_1 \to J_1})}{\mu(A)} \leq 2(r/8)^{\ell} \leq r^{\ell}/4. \end{align} $$
$$ \begin{align} \frac{\mu(A_{I_1 \to J_1})}{\mu(A_{(I \cap I_1) \to (J \cap J_1)})} \leq 2 \cdot \frac{\mu(A_{I_1 \to J_1})}{\mu(A)} \leq 2(r/8)^{\ell} \leq r^{\ell}/4. \end{align} $$
Density increase in the restriction
![]() $I_2 \to J_2$
consisting of chains. Here, we have to bound the ratio between the density increases of the restrictions
$I_2 \to J_2$
consisting of chains. Here, we have to bound the ratio between the density increases of the restrictions
![]() $A_{I_1 \to J_1} \to A_{I_1 \cup I_2 \to J_1 \cup J_2}$
and
$A_{I_1 \to J_1} \to A_{I_1 \cup I_2 \to J_1 \cup J_2}$
and
![]() $A_{(I \cap I_1) \to (J \cap J_1)} \to A_{I \to J}$
. As these restrictions consist only of chains, we can estimate and compare their density increases using Claim 5.2.
$A_{(I \cap I_1) \to (J \cap J_1)} \to A_{I \to J}$
. As these restrictions consist only of chains, we can estimate and compare their density increases using Claim 5.2.
As the restriction
![]() $I\to J$
consists only of
$I\to J$
consists only of
![]() $1$
chains and 2 chains, the value P that corresponds to it in Claim 5.2 is at least
$1$
chains and 2 chains, the value P that corresponds to it in Claim 5.2 is at least
![]() $1/2$
. Therefore, we may assume that
$1/2$
. Therefore, we may assume that
![]() $\ell =|I'\setminus I|\le 4\sqrt {n}/C$
, as otherwise, we have
$\ell =|I'\setminus I|\le 4\sqrt {n}/C$
, as otherwise, we have
![]() $\mu (A_{I'\to J'})\le 1 \le 4^{\ell } \frac {1}{2}\mu (A) \le 4^{\ell } \mu (A_{I\to J})$
. We also have
$\mu (A_{I'\to J'})\le 1 \le 4^{\ell } \frac {1}{2}\mu (A) \le 4^{\ell } \mu (A_{I\to J})$
. We also have
![]() $|I|\le 4\sqrt {n}/C$
by hypothesis.
$|I|\le 4\sqrt {n}/C$
by hypothesis.
Denote the chain lengths of the restrictions
![]() $I_2 \to J_2$
and
$I_2 \to J_2$
and
![]() $(I \cap I_2) \to (J \cap J_2)$
by
$(I \cap I_2) \to (J \cap J_2)$
by
![]() $i^{\prime }_1-1,\ldots ,i^{\prime }_{s'}-1$
and
$i^{\prime }_1-1,\ldots ,i^{\prime }_{s'}-1$
and
![]() $i^{\prime \prime }_1-1,\ldots ,i^{\prime \prime }_{s"}-1$
, respectively, where
$i^{\prime \prime }_1-1,\ldots ,i^{\prime \prime }_{s"}-1$
, respectively, where
![]() $i^{\prime }_j \geq i^{\prime \prime }_j$
for any
$i^{\prime }_j \geq i^{\prime \prime }_j$
for any
![]() $1 \leq j \leq s"$
and
$1 \leq j \leq s"$
and
![]() $s' \geq s"$
. Note that
$s' \geq s"$
. Note that
![]() $s" \leq |I \cap I_2| \leq \frac {4\sqrt {n}}{C}$
and that
$s" \leq |I \cap I_2| \leq \frac {4\sqrt {n}}{C}$
and that
![]() $s'-s" \leq |I_2 \setminus I| \leq \ell \le \frac {4\sqrt {n}}{C}$
. Let
$s'-s" \leq |I_2 \setminus I| \leq \ell \le \frac {4\sqrt {n}}{C}$
. Let
![]() $n'=n-|I_1|\geq n-d-\ell $
and
$n'=n-|I_1|\geq n-d-\ell $
and
![]() $n"=n-|I\cap I_1| \geq n-d$
.
$n"=n-|I\cap I_1| \geq n-d$
.
As the corresponding value of P for the restriction
![]() $I\cap I_2\to J\cap J_2$
is also
$I\cap I_2\to J\cap J_2$
is also
![]() $\ge \frac {1}{2}$
, we may apply Claim 5.2 to obtain that
$\ge \frac {1}{2}$
, we may apply Claim 5.2 to obtain that
 $$\begin{align*}\frac{\mu(A_{I' \to J'})/\mu(A_{I_1 \to J_1})}{\mu(A_{I \to J})/\mu(A_{(I \cap I_1) \to (J \cap J_1)})} \leq 2\frac{\left(1-\frac{s"}{n"}\right)\left(1-\frac{s"}{n"-1}\right) \cdots \left(1-\frac{s"}{n"+1-|I \cap I_2|}\right)}{\left(1-\frac{s'}{n'}\right)\left(1-\frac{s'}{n'-1}\right)\cdots \left(1-\frac{s'}{n'+1-|I_2|}\right)} \le 4, \end{align*}$$
$$\begin{align*}\frac{\mu(A_{I' \to J'})/\mu(A_{I_1 \to J_1})}{\mu(A_{I \to J})/\mu(A_{(I \cap I_1) \to (J \cap J_1)})} \leq 2\frac{\left(1-\frac{s"}{n"}\right)\left(1-\frac{s"}{n"-1}\right) \cdots \left(1-\frac{s"}{n"+1-|I \cap I_2|}\right)}{\left(1-\frac{s'}{n'}\right)\left(1-\frac{s'}{n'-1}\right)\cdots \left(1-\frac{s'}{n'+1-|I_2|}\right)} \le 4, \end{align*}$$
provided that C is sufficiently large. Combining this with (5.1) and (5.2) completes the proof of the lemma.
6 Proof of Theorems 1.3, 1.4 and 1.5
In this section, we prove Theorem 1.3, which states that for a sufficiently large n, for any
![]() $\sigma \in S_n$
with less than
$\sigma \in S_n$
with less than
![]() $n^{2/5-\epsilon }$
cycles, we have
$n^{2/5-\epsilon }$
cycles, we have
![]() $(\sigma ^{S_n})^2=A_n.$
Then, we deduce from it Theorems 1.4 and 1.5.
$(\sigma ^{S_n})^2=A_n.$
Then, we deduce from it Theorems 1.4 and 1.5.
The proof of Theorem 1.3 proceeds in two stages. First, we show that we may strengthen the hypothesis of the theorem by adding the assumption that
![]() $\sigma $
has only a few short cycles, and at the same time weaken the assertion to claiming that
$\sigma $
has only a few short cycles, and at the same time weaken the assertion to claiming that
![]() $(\sigma ^{S_n})^2$
contains any fixed-point free
$(\sigma ^{S_n})^2$
contains any fixed-point free
![]() $\tau \in A_n$
. Afterward, we prove the ‘reduced’ statement.
$\tau \in A_n$
. Afterward, we prove the ‘reduced’ statement.
Formally, in Sections 6.1 and 6.2, we show that it is sufficient to prove the following lemma.
Lemma 6.1. For any
![]() $\epsilon>0$
, there exists
$\epsilon>0$
, there exists
![]() $n_0 \in \mathbb {N}$
such that the following holds for all
$n_0 \in \mathbb {N}$
such that the following holds for all
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $\tau \in A_n$
be a permutation with no fixed points. Suppose that
$\tau \in A_n$
be a permutation with no fixed points. Suppose that
![]() $\sigma \in S_n$
has at most
$\sigma \in S_n$
has at most
![]() $n^{2/5 -\epsilon }$
cycles overall and less than
$n^{2/5 -\epsilon }$
cycles overall and less than
![]() $10$
cycles of length
$10$
cycles of length
![]() $\ell $
for each
$\ell $
for each
![]() $2 \leq \ell \le \log n$
. Then
$2 \leq \ell \le \log n$
. Then
![]() $\tau \in \left (\sigma ^{S_n}\right )^2.$
$\tau \in \left (\sigma ^{S_n}\right )^2.$
The proof of Lemma 6.1 is presented in Section 6.3. The proofs of Theorems 1.4 and 1.5 is presented in Section 6.4.
6.1 Explicit computations
For
![]() $\sigma \in S_m$
and
$\sigma \in S_m$
and
![]() $\tau \in S_{n-m}$
, we write
$\tau \in S_{n-m}$
, we write
![]() $\sigma \oplus \tau $
for the element in
$\sigma \oplus \tau $
for the element in
![]() $S_n$
obtained by letting
$S_n$
obtained by letting
![]() $\sigma $
act on the first m elements and
$\sigma $
act on the first m elements and
![]() $\tau $
act on the last
$\tau $
act on the last
![]() $n-m$
elements. For a conjugacy class
$n-m$
elements. For a conjugacy class
![]() $C_1$
of
$C_1$
of
![]() $S_m$
and a conjugacy class
$S_m$
and a conjugacy class
![]() $C_2$
of
$C_2$
of
![]() $S_{n-m}$
, we write
$S_{n-m}$
, we write
![]() $C_1\oplus C_2$
for the conjugacy class obtained by concatenating their cycle decompositions.
$C_1\oplus C_2$
for the conjugacy class obtained by concatenating their cycle decompositions.
Lemma 6.2. Let
![]() $C_1,C^{\prime }_1,C_1"$
be conjugacy classes of
$C_1,C^{\prime }_1,C_1"$
be conjugacy classes of
![]() $S_m$
with
$S_m$
with
![]() $C_1"\subseteq C_1 \cdot C_1'$
, and let
$C_1"\subseteq C_1 \cdot C_1'$
, and let
![]() $C_2,C_2',C_2"$
be conjugacy classes of
$C_2,C_2',C_2"$
be conjugacy classes of
![]() $S_{n-m}$
with
$S_{n-m}$
with
![]() $C_2"\subseteq C_2 \cdot C_2'.$
Then the set
$C_2"\subseteq C_2 \cdot C_2'.$
Then the set
![]() $(C_1\oplus C_2)\cdot (C_1'\oplus C_2')$
contains
$(C_1\oplus C_2)\cdot (C_1'\oplus C_2')$
contains
![]() $C_1" \oplus C_2".$
$C_1" \oplus C_2".$
Proof. Let
![]() $\pi _1 \in C_1"$
and write
$\pi _1 \in C_1"$
and write
![]() $\pi _1 = \sigma _1\tau _1$
for
$\pi _1 = \sigma _1\tau _1$
for
![]() $\sigma _1'\in C_1,\tau _1 \in C_1'$
. Let
$\sigma _1'\in C_1,\tau _1 \in C_1'$
. Let
![]() $\pi _2\in C_2"$
and let
$\pi _2\in C_2"$
and let
![]() $\sigma _2,\tau _2$
be defined similarly. We have
$\sigma _2,\tau _2$
be defined similarly. We have
![]() $(\sigma _1\oplus \sigma _2)(\tau _1\oplus \tau _2) = (\pi _1 \oplus \pi _2 ).$
$(\sigma _1\oplus \sigma _2)(\tau _1\oplus \tau _2) = (\pi _1 \oplus \pi _2 ).$
Lemma 6.3. There exists
![]() $n_0 \in \mathbb {N}$
such that the following holds for any
$n_0 \in \mathbb {N}$
such that the following holds for any
![]() $n>n_0$
. Let
$n>n_0$
. Let
![]() $r,m$
be integers dividing n, with
$r,m$
be integers dividing n, with
![]() $r> 1$
and
$r> 1$
and
![]() $n/m$
even. Then
$n/m$
even. Then
![]() $(r^{n/r})^{2}\supseteq (m^{n/m}).$
$(r^{n/r})^{2}\supseteq (m^{n/m}).$
Proof. Let
![]() $B=(r^{n/r})$
. For
$B=(r^{n/r})$
. For
![]() $r \ge 4$
, by Theorem 2.12, we have
$r \ge 4$
, by Theorem 2.12, we have
![]() $B^2 = A_n$
. For
$B^2 = A_n$
. For
![]() $r=2$
, we may apply Theorem 2.13 which says that
$r=2$
, we may apply Theorem 2.13 which says that
![]() $B^2$
contains all the permutations that have an even number of cycles of each length. As
$B^2$
contains all the permutations that have an even number of cycles of each length. As
![]() $n/m$
is even by hypothesis, this proves the claim.
$n/m$
is even by hypothesis, this proves the claim.
It now remains to treat the case
![]() $r=3.$
For all
$r=3.$
For all
![]() $m \ge 4$
, we may apply Theorem 2.10 to prove our assertion, as
$m \ge 4$
, we may apply Theorem 2.10 to prove our assertion, as
![]() $E(m^{n/m})=1/m$
by definition. The case
$E(m^{n/m})=1/m$
by definition. The case
![]() $m=3$
is straightforward, as when squaring a permutation of cycle type
$m=3$
is straightforward, as when squaring a permutation of cycle type
![]() $(3^{n/3}),$
we obtain a permutation of the same cycle type. Finally, when
$(3^{n/3}),$
we obtain a permutation of the same cycle type. Finally, when
![]() $m=2$
and
$m=2$
and
![]() $r=3$
, we use the fact that in
$r=3$
, we use the fact that in
![]() $S_{12}$
, we have
$S_{12}$
, we have
 $$ \begin{align*} (2,7,5)(3,8,6)&(1,9,4)(12,11,10) \cdot (1,2,3)(7,4,11)(8,5,12)(9,6,10) = \\ &=(1,7)(2,8)(3,9)(4,10)(5,11)(6,12). \end{align*} $$
$$ \begin{align*} (2,7,5)(3,8,6)&(1,9,4)(12,11,10) \cdot (1,2,3)(7,4,11)(8,5,12)(9,6,10) = \\ &=(1,7)(2,8)(3,9)(4,10)(5,11)(6,12). \end{align*} $$
We now write
![]() $(3^{n/3}) = C_1\oplus \cdots \oplus C_{n/{12}}$
, where each
$(3^{n/3}) = C_1\oplus \cdots \oplus C_{n/{12}}$
, where each
![]() $C_i$
is the conjugacy class of
$C_i$
is the conjugacy class of
![]() $(1,2,3)(4,5,6)(7,8,9)(10,11,12)$
, and write
$(1,2,3)(4,5,6)(7,8,9)(10,11,12)$
, and write
![]() $(2^{n/2}) = D_1\oplus \cdots \oplus D_{n/12},$
where each
$(2^{n/2}) = D_1\oplus \cdots \oplus D_{n/12},$
where each
![]() $D_i$
is the conjugacy class of
$D_i$
is the conjugacy class of
![]() $(1,2)(3,4)(5,6)(7,8),(9,10),(11,12)$
. (Note that in this case, n is indeed divisible by
$(1,2)(3,4)(5,6)(7,8),(9,10),(11,12)$
. (Note that in this case, n is indeed divisible by
![]() $12$
since by assumption,
$12$
since by assumption,
![]() $r=3$
divides n and
$r=3$
divides n and
![]() $n/m=n/2$
is even). Lemma 6.2 now completes the proof.
$n/m=n/2$
is even). Lemma 6.2 now completes the proof.
6.2 Reducing to Lemma 6.1
In this subsection, we reduce the statement of Theorem 1.3 to the statement of Lemma 6.1.
Proof of Theorem 1.3, assuming Lemma 6.1.
Our goal is to show that for a sufficiently large n, for any
![]() $\sigma \in S_n$
with at most
$\sigma \in S_n$
with at most
![]() $n^{2/5 - \epsilon }$
cycles, the conjugacy class
$n^{2/5 - \epsilon }$
cycles, the conjugacy class
![]() $I=\sigma ^{S_n}$
satisfies
$I=\sigma ^{S_n}$
satisfies
![]() $I^2 = A_n$
. Equivalently, we have to show that
$I^2 = A_n$
. Equivalently, we have to show that
![]() $I^2\cap \tau ^{S_n}\ne \emptyset $
for each
$I^2\cap \tau ^{S_n}\ne \emptyset $
for each
![]() $\tau \in A_n$
. Fix such a
$\tau \in A_n$
. Fix such a
![]() $\tau $
and write
$\tau $
and write
![]() $A=\tau ^{S_n}.$
We split the proof into two cases:
$A=\tau ^{S_n}.$
We split the proof into two cases:
-
• Case 1: The number of fixed points of
 $\sigma $
is at most the number of fixed points of
$\sigma $
is at most the number of fixed points of
 $\tau $
.
$\tau $
. -
• Case 2: The number of fixed points of
 $\sigma $
is larger than the number of fixed points of
$\sigma $
is larger than the number of fixed points of
 $\tau $
.
$\tau $
.
Case 1:
![]() $\sigma $
has no more fixed points than
$\sigma $
has no more fixed points than
![]() $\tau $
. Write t for the number of fixed points in
$\tau $
. Write t for the number of fixed points in
![]() $\sigma $
. We may restrict both I and A to the t-umvirate
$\sigma $
. We may restrict both I and A to the t-umvirate
![]() $U = U_{[t]\to [t]}$
to obtain the conjugacy classes
$U = U_{[t]\to [t]}$
to obtain the conjugacy classes
![]() $I'$
,
$I'$
,
![]() $A'$
obtained by removing t fixed points from
$A'$
obtained by removing t fixed points from
![]() $\sigma ,\tau $
. It is clearly sufficient to show that
$\sigma ,\tau $
. It is clearly sufficient to show that
![]() $(I')^2\supseteq A'$
. We may therefore assume that
$(I')^2\supseteq A'$
. We may therefore assume that
![]() $\sigma $
is fixed points free. As
$\sigma $
is fixed points free. As
![]() $\sigma $
has less than
$\sigma $
has less than
![]() $n^{2/5}$
cycles overall, the assertion now follows from Theorem 2.11.
$n^{2/5}$
cycles overall, the assertion now follows from Theorem 2.11.
Case 2:
![]() $\sigma $
has more fixed points than
$\sigma $
has more fixed points than
![]() $\tau $
. By the same argument as in Case 1, we may assume without loss of generality that
$\tau $
. By the same argument as in Case 1, we may assume without loss of generality that
![]() $\tau $
has no fixed points. By Lemma 2.8 (applied with
$\tau $
has no fixed points. By Lemma 2.8 (applied with
![]() $\epsilon /3$
in place of
$\epsilon /3$
in place of
![]() $\epsilon $
), we have
$\epsilon $
), we have
![]() $E(\sigma )\le 2/5 - 2\epsilon /3$
. Theorem 2.10 now completes the proof if
$E(\sigma )\le 2/5 - 2\epsilon /3$
. Theorem 2.10 now completes the proof if
![]() $E(\tau )<1/5$
. Suppose on the contrary that
$E(\tau )<1/5$
. Suppose on the contrary that
![]() $E(\tau ) \geq 1/5$
. By Lemma 2.6, applied with
$E(\tau ) \geq 1/5$
. By Lemma 2.6, applied with
![]() $m=6$
and
$m=6$
and
![]() $\epsilon =1/60$
, this implies that for some
$\epsilon =1/60$
, this implies that for some
![]() $i \leq 5$
,
$i \leq 5$
,
![]() $\tau $
has at least
$\tau $
has at least
![]() $n^{\delta }\ i$
-cycles, for some explicit
$n^{\delta }\ i$
-cycles, for some explicit
![]() $\delta>0$
. (Actually, this holds for all
$\delta>0$
. (Actually, this holds for all
![]() $n>n_0$
, but we may absorb this into our assumption that n is sufficiently large).
$n>n_0$
, but we may absorb this into our assumption that n is sufficiently large).
Suppose that
![]() $\sigma $
has at least
$\sigma $
has at least
![]() $10\ \ell $
-cycles for some
$10\ \ell $
-cycles for some
![]() $2 \leq \ell \le \log n$
, as otherwise, we are done by Lemma 6.1. We may write
$2 \leq \ell \le \log n$
, as otherwise, we are done by Lemma 6.1. We may write
![]() $\sigma = \sigma _1 \oplus \sigma _1'$
and
$\sigma = \sigma _1 \oplus \sigma _1'$
and
![]() $\tau = \tau _1 \oplus \tau _1'$
, where
$\tau = \tau _1 \oplus \tau _1'$
, where
![]() $\tau _1'$
consists of
$\tau _1'$
consists of
![]() $2\ell \ i$
-cycles and
$2\ell \ i$
-cycles and
![]() $\sigma _1'$
consists of
$\sigma _1'$
consists of
![]() $2i\ \ell $
-cycles. (Note that as
$2i\ \ell $
-cycles. (Note that as
![]() $i \leq 5$
,
$i \leq 5$
,
![]() $\sigma $
contains at least
$\sigma $
contains at least
![]() $10 \ge 2i\ \ell $
-cycles, and as
$10 \ge 2i\ \ell $
-cycles, and as
![]() $\ell \leq \log n$
,
$\ell \leq \log n$
,
![]() $\tau $
contains at least
$\tau $
contains at least
![]() $n^{\delta } \ge 2\ell \ i$
-cycles, assuming n is sufficiently large. Hence, the decomposition is possible). By Lemma 6.3, we have
$n^{\delta } \ge 2\ell \ i$
-cycles, assuming n is sufficiently large. Hence, the decomposition is possible). By Lemma 6.3, we have
![]() $((\sigma ^{\prime }_1)^{S_n})^2\supseteq (\tau ^{\prime }_1)^{S_n}$
. Hence, by Lemma 6.2, it is sufficient to show that
$((\sigma ^{\prime }_1)^{S_n})^2\supseteq (\tau ^{\prime }_1)^{S_n}$
. Hence, by Lemma 6.2, it is sufficient to show that
![]() $(\sigma _1^{S_n})^2\supseteq \tau _1^{S_n}$
.
$(\sigma _1^{S_n})^2\supseteq \tau _1^{S_n}$
.
We can repeat the deletion process to obtain a sequence of restrictions
![]() $\sigma _1,\ldots , \sigma _j$
and
$\sigma _1,\ldots , \sigma _j$
and
![]() $\tau _1,\ldots , \tau _j$
, until either
$\tau _1,\ldots , \tau _j$
, until either
![]() $E(\tau _j)<1/5$
or
$E(\tau _j)<1/5$
or
![]() $\sigma _j$
has less than
$\sigma _j$
has less than
![]() $10\ \ell $
-cycles for all
$10\ \ell $
-cycles for all
![]() $2 \leq \ell \leq \log n.$
(Note that as
$2 \leq \ell \leq \log n.$
(Note that as
![]() $\sigma $
contains at most
$\sigma $
contains at most
![]() $n^{2/5}$
cycles, the process terminates when
$n^{2/5}$
cycles, the process terminates when
![]() $\sigma _j,\tau _j$
are permutations on at least
$\sigma _j,\tau _j$
are permutations on at least
![]() $n-10n^{2/5}\log n$
coordinates, and thus, for all
$n-10n^{2/5}\log n$
coordinates, and thus, for all
![]() $1 \leq l \leq j$
, we have
$1 \leq l \leq j$
, we have
![]() $E(\sigma _l)<2/5-\epsilon /2$
, provided that n is sufficiently large). In the former case, we are done by Theorem 2.10. In the latter case, we are done by Lemma 6.1. This completes the proof.
$E(\sigma _l)<2/5-\epsilon /2$
, provided that n is sufficiently large). In the former case, we are done by Theorem 2.10. In the latter case, we are done by Lemma 6.1. This completes the proof.
6.3 Proving Lemma 6.1
Proof of Lemma 6.1.
The proof consists of four steps.
Step 1: Reducing to the case where
![]() $\tau $
has many short cycles. If
$\tau $
has many short cycles. If
![]() $\tau $
has at most
$\tau $
has at most
![]() $n^{2/5 - \epsilon /3}$
cycles of length less than
$n^{2/5 - \epsilon /3}$
cycles of length less than
![]() $\frac {10}{\epsilon }$
, then by Lemma 2.7, we have
$\frac {10}{\epsilon }$
, then by Lemma 2.7, we have
![]() $E(\tau ) \le 1/5 - \epsilon /12$
(provided that n is sufficiently large). As
$E(\tau ) \le 1/5 - \epsilon /12$
(provided that n is sufficiently large). As
![]() $E(\sigma ) \le 2/5 -\epsilon /2$
by Lemma 2.8, Theorem 2.10 implies that
$E(\sigma ) \le 2/5 -\epsilon /2$
by Lemma 2.8, Theorem 2.10 implies that
![]() $\tau \in \left (\sigma ^{S_n}\right )^{2},$
completing the proof.
$\tau \in \left (\sigma ^{S_n}\right )^{2},$
completing the proof.
Hence, we may assume that
![]() $\tau $
has at least
$\tau $
has at least
![]() $n^{2/5 - \epsilon /3}$
cycles of length less than
$n^{2/5 - \epsilon /3}$
cycles of length less than
![]() $\frac {10}{\epsilon }$
. In particular, there exists
$\frac {10}{\epsilon }$
. In particular, there exists
![]() $m\le \frac {10}{\epsilon }$
, such that
$m\le \frac {10}{\epsilon }$
, such that
![]() $\tau $
has at least
$\tau $
has at least
![]() $\frac {\epsilon }{10}n^{2/5 - \epsilon /3}>n^{2/5-\epsilon /2}\ m$
-cycles. We fix such an m and proceed with it.
$\frac {\epsilon }{10}n^{2/5 - \epsilon /3}>n^{2/5-\epsilon /2}\ m$
-cycles. We fix such an m and proceed with it.
We note that this step, which allows us to assume that
![]() $\tau $
has more short cycles of a fixed length than the total number of fixed points of
$\tau $
has more short cycles of a fixed length than the total number of fixed points of
![]() $\sigma $
, is the only step where we crucially use the bound
$\sigma $
, is the only step where we crucially use the bound
![]() $n^{2/5-\epsilon }$
on the number of cycles in
$n^{2/5-\epsilon }$
on the number of cycles in
![]() $\sigma $
. The other steps can be adapted to work with up to
$\sigma $
. The other steps can be adapted to work with up to
![]() $n^{1/2-\epsilon }$
cycles in
$n^{1/2-\epsilon }$
cycles in
![]() $\sigma $
.
$\sigma $
.
Step 2: Removing almost all fixed points of
![]() $\sigma $
by restrictions. We perform a sequence of
$\sigma $
by restrictions. We perform a sequence of
![]() $2m$
-restrictions intended for removing almost all fixed points of
$2m$
-restrictions intended for removing almost all fixed points of
![]() $\sigma $
, in exchange for removing m-cycles of
$\sigma $
, in exchange for removing m-cycles of
![]() $\tau .$
The restrictions are of the form
$\tau .$
The restrictions are of the form
![]() $I_{S'\to T'}$
,
$I_{S'\to T'}$
,
![]() $I_{S'\to W'}$
, and
$I_{S'\to W'}$
, and
![]() $A_{T'\to W'},$
for appropriately chosen sets
$A_{T'\to W'},$
for appropriately chosen sets
![]() $S',T',W'$
. The way in which these restrictions are used is explained at the next step.
$S',T',W'$
. The way in which these restrictions are used is explained at the next step.
Assume for simplicity that m is even. Each
![]() $2m$
-restriction involves
$2m$
-restriction involves
![]() $4m$
coordinates denoted by
$4m$
coordinates denoted by
where
![]() $x_i=y_i$
and
$x_i=y_i$
and
![]() $x^{\prime }_i=y^{\prime }_i$
for all odd i, and except for this, all the coordinates are pairwise distinct. We define the restrictions by setting
$x^{\prime }_i=y^{\prime }_i$
for all odd i, and except for this, all the coordinates are pairwise distinct. We define the restrictions by setting
 $$ \begin{align*} S'=(x_1,x_2,\ldots,x_m&,x^{\prime}_1,x^{\prime}_2,\ldots,x^{\prime}_m), \qquad T'=(y_1,y_2,\ldots,y_m,y^{\prime}_m,y^{\prime}_1,\ldots,y^{\prime}_{m-1}), \\ &W'=(y_m,y_1,\ldots,y_{m-1},y^{\prime}_1,y^{\prime}_2,\ldots,y^{\prime}_m). \end{align*} $$
$$ \begin{align*} S'=(x_1,x_2,\ldots,x_m&,x^{\prime}_1,x^{\prime}_2,\ldots,x^{\prime}_m), \qquad T'=(y_1,y_2,\ldots,y_m,y^{\prime}_m,y^{\prime}_1,\ldots,y^{\prime}_{m-1}), \\ &W'=(y_m,y_1,\ldots,y_{m-1},y^{\prime}_1,y^{\prime}_2,\ldots,y^{\prime}_m). \end{align*} $$
As a result, each of the restrictions
![]() $I_{S'\to T'}, I_{S' \to W'}$
consists of
$I_{S'\to T'}, I_{S' \to W'}$
consists of
![]() $m/2$
1-cycles,
$m/2$
1-cycles,
![]() $m/2$
1-chains and
$m/2$
1-chains and
![]() $m/2$
2-chains, while the restriction
$m/2$
2-chains, while the restriction
![]() $A_{T' \to W'}$
consists of the two m-cycles
$A_{T' \to W'}$
consists of the two m-cycles
![]() $(y_1,y_2,\ldots ,y_m,y_1)$
and
$(y_1,y_2,\ldots ,y_m,y_1)$
and
![]() $(y^{\prime }_1,y^{\prime }_2,\ldots ,y^{\prime }_m,y^{\prime }_1)$
.
$(y^{\prime }_1,y^{\prime }_2,\ldots ,y^{\prime }_m,y^{\prime }_1)$
.
We perform
![]() $s=\lfloor \frac {2f_{\sigma }}{m}\rfloor $
such
$s=\lfloor \frac {2f_{\sigma }}{m}\rfloor $
such
![]() $2m$
-restrictions, where
$2m$
-restrictions, where
![]() $f_{\sigma }$
is the number of fixed points of
$f_{\sigma }$
is the number of fixed points of
![]() $\sigma $
. As a result, all fixed points of
$\sigma $
. As a result, all fixed points of
![]() $\sigma $
, except for at most
$\sigma $
, except for at most
![]() $m/2-1$
, are removed. (Note that we do not ‘get stuck’ on the side of A since the number of m-cycles in
$m/2-1$
, are removed. (Note that we do not ‘get stuck’ on the side of A since the number of m-cycles in
![]() $\tau $
is much larger than the number of fixed points of
$\tau $
is much larger than the number of fixed points of
![]() $\sigma $
, bounded by
$\sigma $
, bounded by
![]() $n^{2/5-\epsilon }$
). We let
$n^{2/5-\epsilon }$
). We let
![]() $I_{S\to T}, I_{S\to W}$
and
$I_{S\to T}, I_{S\to W}$
and
![]() $A_{T\to W}$
be the sets obtained at the end of the process.
$A_{T\to W}$
be the sets obtained at the end of the process.
Step 3: Reducing to edges between vertex sets in a Cayley graph. First, we perform a simple shifting procedure which allows us to ‘get rid’ of the coordinates in
![]() $S,T$
and W. We let
$S,T$
and W. We let
![]() $\pi _1$
be the permutation that fixes the set of coordinates not appearing in W and sends the tuple W to the tuple T. The permutation
$\pi _1$
be the permutation that fixes the set of coordinates not appearing in W and sends the tuple W to the tuple T. The permutation
![]() $\pi _1$
consists of
$\pi _1$
consists of
![]() $2s\ m$
-cycles on the elements appearing in W. Let
$2s\ m$
-cycles on the elements appearing in W. Let
![]() $\pi _2$
be an arbitrary permutation that sends S to W. Consider the sets
$\pi _2$
be an arbitrary permutation that sends S to W. Consider the sets
![]() $ B_1 = \pi _2 I \pi _1, B_2 = \pi _2 I, B_3 = A \pi _1.$
As
$ B_1 = \pi _2 I \pi _1, B_2 = \pi _2 I, B_3 = A \pi _1.$
As
![]() $(B_2)^{-1} B_1 = I^{-1}I \pi _1$
and
$(B_2)^{-1} B_1 = I^{-1}I \pi _1$
and
![]() $I^{-1}I=I^2$
; it is sufficient to prove that
$I^{-1}I=I^2$
; it is sufficient to prove that
![]() $(B_2)^{-1} B_1$
has a nonempty intersection with
$(B_2)^{-1} B_1$
has a nonempty intersection with
![]() $B_3 = A\pi _1.$
In fact, we show that
$B_3 = A\pi _1.$
In fact, we show that
![]() $(B_2^{-1})_{W\to W}(B_1)_{W\to W}$
has a nonempty intersection with
$(B_2^{-1})_{W\to W}(B_1)_{W\to W}$
has a nonempty intersection with
![]() $(B_3)_{W\to W}.$
$(B_3)_{W\to W}.$
Assume without loss of generality that
![]() $W = \{(n-2sm+1,\ldots , n)\}$
and identify
$W = \{(n-2sm+1,\ldots , n)\}$
and identify
![]() $S_{n-2ms}$
with the set of permutations in
$S_{n-2ms}$
with the set of permutations in
![]() $S_n$
fixing W. Then
$S_n$
fixing W. Then
![]() $(A\pi _1)_{W\to W}$
is the conjugacy class
$(A\pi _1)_{W\to W}$
is the conjugacy class
![]() $(\tau ')^{S_{n-2ms}}$
of
$(\tau ')^{S_{n-2ms}}$
of
![]() $S_{n-2ms}$
, obtained by deleting
$S_{n-2ms}$
, obtained by deleting
![]() $2s\ m$
-cycles from
$2s\ m$
-cycles from
![]() $\tau $
. Our goal is showing that the sets
$\tau $
. Our goal is showing that the sets
![]() $(B_2)_{W\to W}, (B_1)_{W\to W}$
span an edge in the Cayley graph
$(B_2)_{W\to W}, (B_1)_{W\to W}$
span an edge in the Cayley graph
![]() $\mathrm {Cay}(S_n, (\tau ')^{S_{n-2ms}})$
. Furthermore, we can reduce the problem to
$\mathrm {Cay}(S_n, (\tau ')^{S_{n-2ms}})$
. Furthermore, we can reduce the problem to
![]() $A_{n-2ms}$
(i.e., assume w.l.o.g. that
$A_{n-2ms}$
(i.e., assume w.l.o.g. that
![]() $B_2,B_3$
are contained in
$B_2,B_3$
are contained in
![]() $A_{n-2ms}$
by multiplying all odd permutations in
$A_{n-2ms}$
by multiplying all odd permutations in
![]() $B_2$
by some fixed permutation and multiplying all odd permutations in
$B_2$
by some fixed permutation and multiplying all odd permutations in
![]() $B_3$
by its inverse).
$B_3$
by its inverse).
Step 4: Completing the proof using Proposition 4.1. The sets
![]() $(B_1)_{W\to W}, (B_2)_{W\to W}$
are shifts of the sets
$(B_1)_{W\to W}, (B_2)_{W\to W}$
are shifts of the sets
![]() $I_{S\to T}, I_{S\to W}$
, and therefore inherit their spreadness. In order to apply Proposition 4.1, we establish the
$I_{S\to T}, I_{S\to W}$
, and therefore inherit their spreadness. In order to apply Proposition 4.1, we establish the
![]() $\delta $
-spreadness of
$\delta $
-spreadness of
![]() $I_{S\to T}$
and
$I_{S\to T}$
and
![]() $I_{S\to W}$
. We may view the restrictions
$I_{S\to W}$
. We may view the restrictions
![]() $I_{S \to T}$
and
$I_{S \to T}$
and
![]() $I_{S \to W}$
as a composition of two restrictions – a restriction that removes all fixed points except for at most
$I_{S \to W}$
as a composition of two restrictions – a restriction that removes all fixed points except for at most
![]() $m/2-1$
and a restriction that consists only of 1-chains and 2-chains. By the assumption on
$m/2-1$
and a restriction that consists only of 1-chains and 2-chains. By the assumption on
![]() $\sigma $
, this allows us to apply Lemma 5.3, with any constant
$\sigma $
, this allows us to apply Lemma 5.3, with any constant
![]() $r>\max (10m,200)$
, to deduce that the sets
$r>\max (10m,200)$
, to deduce that the sets
![]() $B_2$
and
$B_2$
and
![]() $B_3$
are r-global. It follows that
$B_3$
are r-global. It follows that
![]() $B_2,B_3$
are
$B_2,B_3$
are
![]() $\delta $
-spread for an arbitrarily small
$\delta $
-spread for an arbitrarily small
![]() $\delta>0$
, provided that n is sufficiently large.
$\delta>0$
, provided that n is sufficiently large.
As follows from Claim 5.2, a restriction that consists of 1-cycles, 1-chains and 2-chains cannot decrease the measure of a conjugacy class by more than a factor of 2, and hence, we have
![]() $\mu (I'),\mu (I") \geq e^{-n^{2/5-2\epsilon }}$
. Furthermore,
$\mu (I'),\mu (I") \geq e^{-n^{2/5-2\epsilon }}$
. Furthermore,
![]() $\tau '$
is fixed-point free, and hence, by Lemma 2.5, we have
$\tau '$
is fixed-point free, and hence, by Lemma 2.5, we have
![]() $E(\tau ')\leq 1/2$
. Consequently, by Theorem 2.2, we have
$E(\tau ')\leq 1/2$
. Consequently, by Theorem 2.2, we have
 $$\begin{align*}\frac{\widehat{1_{A'}}(\chi)}{\mu(A')}=\chi(\tau') < \chi(1)^{1/2+\epsilon},\end{align*}$$
$$\begin{align*}\frac{\widehat{1_{A'}}(\chi)}{\mu(A')}=\chi(\tau') < \chi(1)^{1/2+\epsilon},\end{align*}$$
for any
![]() $\chi \in \widehat {A_{n-2ms}}$
, provided that n is sufficiently large. Hence, Proposition 4.1, applied with
$\chi \in \widehat {A_{n-2ms}}$
, provided that n is sufficiently large. Hence, Proposition 4.1, applied with
![]() $\alpha =1/2+\epsilon $
, implies that the sets
$\alpha =1/2+\epsilon $
, implies that the sets
![]() $I',I"$
span an edge in the Cayley graph
$I',I"$
span an edge in the Cayley graph
![]() $\mathrm {Cay}(A_{n-2ms}, A')$
, completing the proof.
$\mathrm {Cay}(A_{n-2ms}, A')$
, completing the proof.
6.4 Proving Theorems 1.4 and 1.5
In the proof of Theorem 1.4, we use the following standard fact regarding the cycle structure of random permutations. For
![]() $\sigma \in S_n$
, denote by
$\sigma \in S_n$
, denote by
![]() $C(\sigma )$
the total number of cycles in
$C(\sigma )$
the total number of cycles in
![]() $\sigma $
.
$\sigma $
.
Proposition 6.4 [Reference Ford9, Corollary 1.6].
For any
![]() $n \in \mathbb {N}$
and any
$n \in \mathbb {N}$
and any
![]() $0 \leq m \le n$
, we have
$0 \leq m \le n$
, we have
![]() $\Pr _{\sigma \sim S_n}[C(\sigma )=m]\le \frac {(2\log (n))^{m-1}}{(m-1)!}. $
$\Pr _{\sigma \sim S_n}[C(\sigma )=m]\le \frac {(2\log (n))^{m-1}}{(m-1)!}. $
Proof of Theorem 1.4.
Let A be a normal set with
![]() $\mu (A)\ge e^{-n^{2/5 -\epsilon }}$
. We claim that for a sufficiently large n, A contains a conjugacy class
$\mu (A)\ge e^{-n^{2/5 -\epsilon }}$
. We claim that for a sufficiently large n, A contains a conjugacy class
![]() $C=\sigma ^{S_n}$
with
$C=\sigma ^{S_n}$
with
![]() $\mu (C)\ge e^{-n^{2/5 -\epsilon /3}}$
. Once we show this, the assertion of the theorem follows by applying Theorem 1.3 to C.
$\mu (C)\ge e^{-n^{2/5 -\epsilon /3}}$
. Once we show this, the assertion of the theorem follows by applying Theorem 1.3 to C.
It is clearly sufficient to show that for a sufficiently large n, the union of all conjugacy classes
![]() $C'=(\sigma ')^{S_n}$
with
$C'=(\sigma ')^{S_n}$
with
![]() $\mu (C')<e^{-n^{2/5-\epsilon /3}}$
has measure
$\mu (C')<e^{-n^{2/5-\epsilon /3}}$
has measure
![]() $< e^{-n^{2/5-\epsilon }}$
.
$< e^{-n^{2/5-\epsilon }}$
.
Recall that
![]() $\mu (C')=[\prod _{i=1}^n (i^{f_{\sigma '}(i)}\cdot f_{\sigma '}(i)!)]^{-1}$
. Hence, by taking logaritheorems, the assumption
$\mu (C')=[\prod _{i=1}^n (i^{f_{\sigma '}(i)}\cdot f_{\sigma '}(i)!)]^{-1}$
. Hence, by taking logaritheorems, the assumption
![]() $\mu (C')<e^{-n^{2/5-\epsilon /3}}$
implies
$\mu (C')<e^{-n^{2/5-\epsilon /3}}$
implies
 $$\begin{align*}\sum_{i=1}^n f_{\sigma'}(i)\log(i)+ f_{\sigma'}(i)\log(f_{\sigma'}(i)) \ge n^{2/5-\epsilon/3}, \end{align*}$$
$$\begin{align*}\sum_{i=1}^n f_{\sigma'}(i)\log(i)+ f_{\sigma'}(i)\log(f_{\sigma'}(i)) \ge n^{2/5-\epsilon/3}, \end{align*}$$
and subsequently,
![]() $C(\sigma ')=\sum _{i=1}^n f_{\sigma '}(i) \geq n^{2/5-2\epsilon /3}$
, provided that n is sufficiently large. By Proposition 6.4, the probability that a random
$C(\sigma ')=\sum _{i=1}^n f_{\sigma '}(i) \geq n^{2/5-2\epsilon /3}$
, provided that n is sufficiently large. By Proposition 6.4, the probability that a random
![]() $\sigma '$
satisfies this condition is less than
$\sigma '$
satisfies this condition is less than
![]() $e^{-n^{2/5-\epsilon }}$
, provided that n is sufficiently large. The assertion follows.
$e^{-n^{2/5-\epsilon }}$
, provided that n is sufficiently large. The assertion follows.
Proof of Theorem 1.5.
Let A be a normal subset of
![]() $A_n$
of density
$A_n$
of density
![]() $\ge e^{-n^{2/5 -\epsilon }}$
. If A is a normal subset of
$\ge e^{-n^{2/5 -\epsilon }}$
. If A is a normal subset of
![]() $S_n$
as well, then the statement follows from Theorem 1.4. Otherwise, A contains a permutation
$S_n$
as well, then the statement follows from Theorem 1.4. Otherwise, A contains a permutation
![]() $\sigma $
with
$\sigma $
with
![]() $\sigma ^{A_n} \ne \sigma ^{S_n}$
, in which case the statement follows from Theorem 2.9.
$\sigma ^{A_n} \ne \sigma ^{S_n}$
, in which case the statement follows from Theorem 2.9.
Acknowledgements
This work was done while N. L. and O. S. were visiting the Simons Institute for the Theory of Computing.
Competing interest
The authors certify that there are no actual or potential competing interest.
Financial support
N. K. is supported by the Israel Science Foundation (grant no. 2669/21). N. L. is supported by the Israel Science Foundation (grant no.1980/22).