Published online by Cambridge University Press: 15 October 2004
We study the corrector matrix $P^{\varepsilon}$ 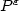 to the conductivity equations. We showthat if $P^{\varepsilon}$
to the conductivity equations. We showthat if $P^{\varepsilon}$ 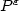 converges weakly to the identity, then for any laminate $\det P^{\varepsilon}\geq 0$
converges weakly to the identity, then for any laminate $\det P^{\varepsilon}\geq 0$ 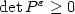 at almost every point. This simple property is shown to be false forgeneric microgeometries if the dimension is greater than two in the work Briane et al. [Arch. Ration. Mech. Anal., to appear].In two dimensions it holds true for any microgeometry as a corollary of the work in Alessandrini and Nesi [Arch. Ration. Mech. Anal.158 (2001) 155-171]. We use this property of laminates to prove that, in any dimension, the classicalHashin-Shtrikman bounds are not attained by laminates, in certain regimes, when the number ofphases is greater than two. In addition we establish new bounds for the effective conductivity,which are asymptotically optimal for mixtures of three isotropic phases among a certain class ofmicrogeometries, including orthogonal laminates, which we then call quasiorthogonal.
at almost every point. This simple property is shown to be false forgeneric microgeometries if the dimension is greater than two in the work Briane et al. [Arch. Ration. Mech. Anal., to appear].In two dimensions it holds true for any microgeometry as a corollary of the work in Alessandrini and Nesi [Arch. Ration. Mech. Anal.158 (2001) 155-171]. We use this property of laminates to prove that, in any dimension, the classicalHashin-Shtrikman bounds are not attained by laminates, in certain regimes, when the number ofphases is greater than two. In addition we establish new bounds for the effective conductivity,which are asymptotically optimal for mixtures of three isotropic phases among a certain class ofmicrogeometries, including orthogonal laminates, which we then call quasiorthogonal.