Article contents
Spectre et géométrie conforme des variétés compactes à bord
Published online by Cambridge University Press: 28 October 2014
Abstract
We prove that on any compact manifold 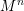 $M^{n}$ with boundary, there exists a conformal class
$M^{n}$ with boundary, there exists a conformal class  $C$ such that for any Riemannian metric
$C$ such that for any Riemannian metric 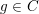 $g\in C$ of unit volume, the first positive eigenvalue of the Neumann Laplacian satisfies
$g\in C$ of unit volume, the first positive eigenvalue of the Neumann Laplacian satisfies 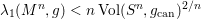 ${\it\lambda}_{1}(M^{n},g)<n\,\text{Vol}(S^{n},g_{\text{can}})^{2/n}$. We also prove a similar inequality for the first positive Steklov eigenvalue. The proof relies on a handle decomposition of the manifold. We also prove that the conformal volume of
${\it\lambda}_{1}(M^{n},g)<n\,\text{Vol}(S^{n},g_{\text{can}})^{2/n}$. We also prove a similar inequality for the first positive Steklov eigenvalue. The proof relies on a handle decomposition of the manifold. We also prove that the conformal volume of  $(M,C)$ is
$(M,C)$ is 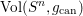 $\text{Vol}(S^{n},g_{\text{can}})$, and that the Friedlander–Nadirashvili invariant and the Möbius volume of
$\text{Vol}(S^{n},g_{\text{can}})$, and that the Friedlander–Nadirashvili invariant and the Möbius volume of 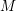 $M$ are equal to those of the sphere. If
$M$ are equal to those of the sphere. If  $M$ is a domain in a space form,
$M$ is a domain in a space form, 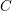 $C$ is the conformal class of the canonical metric.
$C$ is the conformal class of the canonical metric.
Information
- Type
- Research Article
- Information
- Copyright
- © The Author 2014
References
- 1
- Cited by

