Article contents
On the Radius of Comparison of a Commutative C*-algebra
Published online by Cambridge University Press: 20 November 2018
Abstract.
Let  $X$ be a compact metric space. A lower bound for the radius of comparison of the
$X$ be a compact metric space. A lower bound for the radius of comparison of the 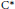 ${{\text{C}}^{*}}$ -algebra
${{\text{C}}^{*}}$ -algebra  $\text{C}\left( X \right)$ is given in terms of
$\text{C}\left( X \right)$ is given in terms of  ${{\dim}_{\mathbb{Q}}}\,X$ , where
${{\dim}_{\mathbb{Q}}}\,X$ , where  ${{\dim}_{\mathbb{Q}}}\,X$ is the cohomological dimension with rational coefficients. If
${{\dim}_{\mathbb{Q}}}\,X$ is the cohomological dimension with rational coefficients. If 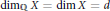 ${{\dim}_{\mathbb{Q}}}\,X\,=\,\dim\,X\,=\,d$ , then the radius of comparison of the
${{\dim}_{\mathbb{Q}}}\,X\,=\,\dim\,X\,=\,d$ , then the radius of comparison of the 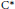 ${{\text{C}}^{*}}$ -algebra
${{\text{C}}^{*}}$ -algebra  $C\left( X \right)$ is
$C\left( X \right)$ is 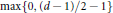 $\max \left\{ 0,\,\left( d\,-\,1 \right)/\,2\,-\,1 \right\}$ if
$\max \left\{ 0,\,\left( d\,-\,1 \right)/\,2\,-\,1 \right\}$ if  $d$ is odd, and must be either
$d$ is odd, and must be either 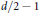 ${d}/{2}\;\,-\,1$ or
${d}/{2}\;\,-\,1$ or  ${d}/{2}\;\,-\,2$ if
${d}/{2}\;\,-\,2$ if  $d$ is even (the possibility
$d$ is even (the possibility 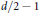 ${d}/{2}\;\,-\,1$ does occur, but we do not know if the possibility
${d}/{2}\;\,-\,1$ does occur, but we do not know if the possibility  ${d}/{2}\;\,-\,2$ can also occur).
${d}/{2}\;\,-\,2$ can also occur).
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2013
References
- 7
- Cited by

