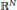Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
DEL PEZZO, LEANDRO M.
2010.
OPTIMIZATION PROBLEM FOR EXTREMALS OF THE TRACE INEQUALITY IN DOMAINS WITH HOLES.
Communications in Contemporary Mathematics,
Vol. 12,
Issue. 04,
p.
569.
Lamberti, Pier Domenico
2014.
Steklov-type eigenvalues associated with best Sobolev trace constants: domain perturbation and overdetermined systems.
Complex Variables and Elliptic Equations,
Vol. 59,
Issue. 3,
p.
309.
Ferone, Vincenzo
Nitsch, Carlo
and
Trombetti, Cristina
2014.
On a conjectured reverse Faber-Krahn inequality for a Steklov--type Laplacian eigenvalue.
Communications on Pure and Applied Analysis,
Vol. 14,
Issue. 1,
p.
63.
Kovařík, Hynek
and
Pankrashkin, Konstantin
2017.
On the p-Laplacian with Robin boundary conditions and boundary trace theorems.
Calculus of Variations and Partial Differential Equations,
Vol. 56,
Issue. 2,
Hyvönen, N.
Kaarnioja, V.
Mustonen, L.
and
Staboulis, S.
2017.
Polynomial Collocation for Handling an Inaccurately Known Measurement Configuration in Electrical Impedance Tomography.
SIAM Journal on Applied Mathematics,
Vol. 77,
Issue. 1,
p.
202.
Cianchi, Andrea
Ferone, Vincenzo
Nitsch, Carlo
and
Trombetti, Cristina
2017.
Balls minimize trace constants in BV.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2017,
Issue. 725,
p.
41.
Cianchi, Andrea
Ferone, Vincenzo
Nitsch, Carlo
and
Trombetti, Cristina
2018.
Poincaré Trace Inequalities in $$\textit{BV}({\mathbb {B}}^n)$$ BV ( B n ) with Non-standard Normalization.
The Journal of Geometric Analysis,
Vol. 28,
Issue. 4,
p.
3522.