No CrossRef data available.
Published online by Cambridge University Press: 13 October 2020
We show that the space 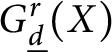 $G^r_{\underline d}(X)$
of linear series of certain multi-degree
$G^r_{\underline d}(X)$
of linear series of certain multi-degree
 $\underline d=(d_1,d_2)$
(including the balanced ones) and rank r on a general genus-g binary curve X has dimension
$\underline d=(d_1,d_2)$
(including the balanced ones) and rank r on a general genus-g binary curve X has dimension
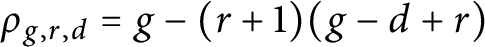 $\rho _{g,r,d}=g-(r+1)(g-d+r)$
if nonempty, where
$\rho _{g,r,d}=g-(r+1)(g-d+r)$
if nonempty, where
 $d=d_1+d_2$
. This generalizes Caporaso’s result from the case
$d=d_1+d_2$
. This generalizes Caporaso’s result from the case
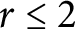 $r\leq 2$
to arbitrary rank, and shows that the space of Osserman-limit linear series on a general binary curve has the expected dimension, which was known for
$r\leq 2$
to arbitrary rank, and shows that the space of Osserman-limit linear series on a general binary curve has the expected dimension, which was known for
 $r\leq 2$
. In addition, we show that the space
$r\leq 2$
. In addition, we show that the space
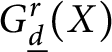 $G^r_{\underline d}(X)$
is still of expected dimension after imposing certain ramification conditions with respect to a sequence of increasing effective divisors supported on two general points
$G^r_{\underline d}(X)$
is still of expected dimension after imposing certain ramification conditions with respect to a sequence of increasing effective divisors supported on two general points
 $P_i\in Z_i$
, where
$P_i\in Z_i$
, where
 $i=1,2$
and
$i=1,2$
and
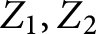 $Z_1,Z_2$
are the two components of X. Our result also has potential application to the lifting problem of divisors on graphs to divisors on algebraic curves.
$Z_1,Z_2$
are the two components of X. Our result also has potential application to the lifting problem of divisors on graphs to divisors on algebraic curves.