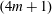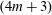Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Saha, Kuldeep
2020.
Contact and isocontact embedding of $${\varvec{\pi }}$$-manifolds.
Proceedings - Mathematical Sciences,
Vol. 130,
Issue. 1,
Ghanwat, Abhijeet
Nath, Arijit
and
Saha, Kuldeep
2022.
Relative LF embeddings of 4-manifolds.
Proceedings - Mathematical Sciences,
Vol. 132,
Issue. 2,
Nath, Arijit
and
Saha, Kuldeep
2023.
Open books and embeddings of smooth and contact manifolds.
Advances in Geometry,
Vol. 23,
Issue. 2,
p.
247.