Published online by Cambridge University Press: 20 November 2018
Culler and Vogtmann defined a simplicial space  $O\left( g \right)$, called outer space, to study the outer automorphism group of the free group
$O\left( g \right)$, called outer space, to study the outer automorphism group of the free group  ${{F}_{g}}$. Using representation theoretic methods, we give an embedding of
${{F}_{g}}$. Using representation theoretic methods, we give an embedding of  $O\left( g \right)$ into the analytification of
$O\left( g \right)$ into the analytification of  $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$, the
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$, the 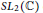 $S{{L}_{2}}\left( \mathbb{C} \right)$ character variety of
$S{{L}_{2}}\left( \mathbb{C} \right)$ character variety of  ${{F}_{g}}$, reproving a result of Morgan and Shalen. Then we show that every point
${{F}_{g}}$, reproving a result of Morgan and Shalen. Then we show that every point  $v$ contained in a maximal cell of
$v$ contained in a maximal cell of  $O\left( g \right)$ defines a flat degeneration of
$O\left( g \right)$ defines a flat degeneration of  $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ to a toric variety
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ to a toric variety 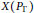 $X\left( {{P}_{\Gamma }} \right)$. We relate
$X\left( {{P}_{\Gamma }} \right)$. We relate
 $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ and
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ and 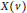 $X\left( v \right)$ topologically by showing that there is a surjective, continuous, proper map
$X\left( v \right)$ topologically by showing that there is a surjective, continuous, proper map 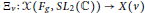 ${{\Xi }_{v}}\,:\,x\left( {{F}_{g}}\,,\,S{{L}_{2}}\,\left( \mathbb{C} \right) \right)\,\to \,X\left( v \right)$. We then show that this map is a symplectomorphism on a dense open subset of
${{\Xi }_{v}}\,:\,x\left( {{F}_{g}}\,,\,S{{L}_{2}}\,\left( \mathbb{C} \right) \right)\,\to \,X\left( v \right)$. We then show that this map is a symplectomorphism on a dense open subset of  $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ with respect to natural symplectic structures on
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ with respect to natural symplectic structures on  $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ and
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ and 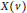 $X\left( v \right)$. In this way, we construct an integrable Hamiltonian system in
$X\left( v \right)$. In this way, we construct an integrable Hamiltonian system in  $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ for each point in a maximal cell of
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ for each point in a maximal cell of  $O\left( g \right)$, and we show that each
$O\left( g \right)$, and we show that each  $v$ defines a topological decomposition of
$v$ defines a topological decomposition of
 $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ derived from the decomposition of
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$ derived from the decomposition of 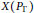 $X\left( {{P}_{\Gamma }} \right)$ by its torus orbits. Finally, we show that the valuations coming from the closure of a maximal cell in
$X\left( {{P}_{\Gamma }} \right)$ by its torus orbits. Finally, we show that the valuations coming from the closure of a maximal cell in  $O\left( g \right)$ all arise as divisorial valuations built from an associated projective compactification of
$O\left( g \right)$ all arise as divisorial valuations built from an associated projective compactification of  $x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$.
$x\left( {{F}_{g}}\,,\,S{{L}_{2}}\left( \mathbb{C} \right) \right)$.