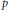 $p$-Convex,
$p$-Convex, 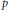 $p$-Concave and Positive
$p$-Concave and Positive 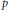 $p$-Summing Operators
$p$-Summing OperatorsPublished online by Cambridge University Press: 20 November 2018
We use Krivine's form of the Grothendieck inequality to renorm the space of bounded linear maps acting between Banach lattices. We construct preduals and describe the nuclear operators associated with these preduals for this renormed space of bounded operators as well as for the spaces of 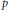 $p$-convex,
$p$-convex, 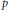 $p$-concave and positive
$p$-concave and positive 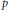 $p$-summing operators acting between Banach lattices and Banach spaces. The nuclear operators obtained are described in terms of factorizations through classical Banach spaces via positive operators.
$p$-summing operators acting between Banach lattices and Banach spaces. The nuclear operators obtained are described in terms of factorizations through classical Banach spaces via positive operators.