Published online by Cambridge University Press: 20 November 2018
Nous établissons une variante infinitésimale de la formule des traces de Jacquet-Rallis pour les groupes unitaires. Notre formule s’obtient par intégration d'un noyau tronqué á la Arthur. Elle posséde un côté géométrique qui est une somme de distributions 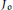 ${{J}_{\mathfrak{o}}}$ indexée par les classes d'éléments de l'algébre de Lie de
${{J}_{\mathfrak{o}}}$ indexée par les classes d'éléments de l'algébre de Lie de  $U\,\left( n\,+\,1 \right)$ stables par
$U\,\left( n\,+\,1 \right)$ stables par 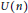 $U\left( n \right)$-conjugaison ainsi qu'un “côté spectral” formé des transformées de Fourier des distributions précédentes. On démontre que les distributions
$U\left( n \right)$-conjugaison ainsi qu'un “côté spectral” formé des transformées de Fourier des distributions précédentes. On démontre que les distributions 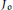 ${{J}_{\mathfrak{o}}}$ sont invariantes et ne dépendent que du choix de la mesure de Haar sur
${{J}_{\mathfrak{o}}}$ sont invariantes et ne dépendent que du choix de la mesure de Haar sur  $U\left( n \right)\left( \mathbb{A} \right)$. Pour des classes
$U\left( n \right)\left( \mathbb{A} \right)$. Pour des classes  $\mathfrak{o}$ semi-simples réguliéres,
$\mathfrak{o}$ semi-simples réguliéres, 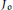 ${{J}_{\mathfrak{o}}}$ est une intégrale orbitale relative de Jacquet-Rallis. Pour les classes
${{J}_{\mathfrak{o}}}$ est une intégrale orbitale relative de Jacquet-Rallis. Pour les classes  $\mathfrak{o}$ dites relativement semi-simples régulières, on exprime
$\mathfrak{o}$ dites relativement semi-simples régulières, on exprime 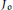 ${{J}_{\mathfrak{o}}}$ en terme des intégrales orbitales relatives régularisées á l'aide des fonctions zêta.
${{J}_{\mathfrak{o}}}$ en terme des intégrales orbitales relatives régularisées á l'aide des fonctions zêta.