No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Let A be a (k + 1) × (k + 1) nonzero matrix. For polynomials p ∈ Pn, set  and
and 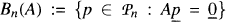 . Let E ⊂ C be a compact set that does not separate the plane and f be a function continuous on E and analytic in the interior of E. Set
. Let E ⊂ C be a compact set that does not separate the plane and f be a function continuous on E and analytic in the interior of E. Set 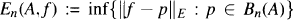 and
and  . Our goal is to study approximation to f on E by polynomials from Bn(A). We obtain necessary and sufficient conditions on the matrix A for the convergence En(A,f) → 0 to take place. These results depend on whether zero lies inside, on the boundary or outside E and yield generalizations of theorems of Clunie, Hasson and Saff for approximation by polynomials that omit a power of z. Let
. Our goal is to study approximation to f on E by polynomials from Bn(A). We obtain necessary and sufficient conditions on the matrix A for the convergence En(A,f) → 0 to take place. These results depend on whether zero lies inside, on the boundary or outside E and yield generalizations of theorems of Clunie, Hasson and Saff for approximation by polynomials that omit a power of z. Let 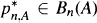 be such that
be such that 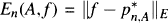 . We also study the asymptotic behavior of the zeros of
. We also study the asymptotic behavior of the zeros of 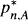 and the asymptotic relation between En(f) and En(A,f).
and the asymptotic relation between En(f) and En(A,f).