No CrossRef data available.
Published online by Cambridge University Press: 12 July 2021
Similarly to the Frobenius–Schur indicator of irreducible characters, we consider higher Frobenius–Schur indicators
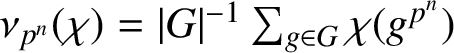 $\nu _{p^n}(\chi ) = |G|^{-1} \sum _{g \in G} \chi (g^{p^n})$
for primes p and
$\nu _{p^n}(\chi ) = |G|^{-1} \sum _{g \in G} \chi (g^{p^n})$
for primes p and
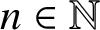 $n \in \mathbb {N}$
, where G is a finite group and
$n \in \mathbb {N}$
, where G is a finite group and
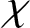 $\chi $
is a generalised character of G. These invariants give answers to interesting questions in representation theory. In particular, we give several characterisations of groups via higher Frobenius–Schur indicators.
$\chi $
is a generalised character of G. These invariants give answers to interesting questions in representation theory. In particular, we give several characterisations of groups via higher Frobenius–Schur indicators.
Both authors were supported by NSFC (11661042 and 11761034) and the Natural Science Foundation of Jiangxi Province (20192ACB21008).