Published online by Cambridge University Press: 20 December 2017
We construct travelling waves in the Burgers equation with the fractional Laplacian 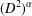 $(D^{2})^{\unicode[STIX]{x1D6FC}}$,
$(D^{2})^{\unicode[STIX]{x1D6FC}}$,  $\unicode[STIX]{x1D6FC}\in (1/2,1)$. This is done by first constructing odd solutions
$\unicode[STIX]{x1D6FC}\in (1/2,1)$. This is done by first constructing odd solutions  $u_{\unicode[STIX]{x1D700}}$ of
$u_{\unicode[STIX]{x1D700}}$ of 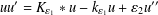 $uu^{\prime }=K_{\unicode[STIX]{x1D700}_{1}}\ast u-k_{\unicode[STIX]{x1D700}_{1}}u+\unicode[STIX]{x1D700}_{2}u^{\prime \prime }$,
$uu^{\prime }=K_{\unicode[STIX]{x1D700}_{1}}\ast u-k_{\unicode[STIX]{x1D700}_{1}}u+\unicode[STIX]{x1D700}_{2}u^{\prime \prime }$, 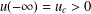 $u(-\infty )=u_{c}>0$, with
$u(-\infty )=u_{c}>0$, with 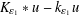 $K_{\unicode[STIX]{x1D700}_{1}}\ast u-k_{\unicode[STIX]{x1D700}_{1}}u$ nonsingular, and then passing to the limit
$K_{\unicode[STIX]{x1D700}_{1}}\ast u-k_{\unicode[STIX]{x1D700}_{1}}u$ nonsingular, and then passing to the limit 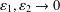 $\unicode[STIX]{x1D700}_{1},\unicode[STIX]{x1D700}_{2}\rightarrow 0$, to give
$\unicode[STIX]{x1D700}_{1},\unicode[STIX]{x1D700}_{2}\rightarrow 0$, to give 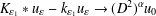 $K_{\unicode[STIX]{x1D700}_{1}}\ast u_{\unicode[STIX]{x1D700}}-k_{\unicode[STIX]{x1D700}_{1}}u_{\unicode[STIX]{x1D700}}\rightarrow (D^{2})^{\unicode[STIX]{x1D6FC}}u_{0}$ pointwise. The proof relies on operator splitting.
$K_{\unicode[STIX]{x1D700}_{1}}\ast u_{\unicode[STIX]{x1D700}}-k_{\unicode[STIX]{x1D700}_{1}}u_{\unicode[STIX]{x1D700}}\rightarrow (D^{2})^{\unicode[STIX]{x1D6FC}}u_{0}$ pointwise. The proof relies on operator splitting.