No CrossRef data available.
 $(H, G)$-COINCIDENCES SET IN REPRESENTATION SPHERES
$(H, G)$-COINCIDENCES SET IN REPRESENTATION SPHERESPublished online by Cambridge University Press: 17 October 2022
Let W be a real vector space and let V be an orthogonal representation of a group G such that
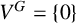 $V^{G} = \{0\}$
(for the set of fixed points of G). Let
$V^{G} = \{0\}$
(for the set of fixed points of G). Let
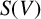 $S(V)$
be the sphere of V and suppose that
$S(V)$
be the sphere of V and suppose that
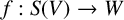 $f: S(V) \to W$
is a continuous map. We estimate the size of the
$f: S(V) \to W$
is a continuous map. We estimate the size of the
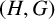 $(H, G)$
-coincidences set if G is a cyclic group of prime power order
$(H, G)$
-coincidences set if G is a cyclic group of prime power order
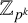 $\mathbb {Z}_{p^k}$
or a p-torus
$\mathbb {Z}_{p^k}$
or a p-torus
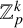 $\mathbb {Z}_p^k$
.
$\mathbb {Z}_p^k$
.